
Board of Governors of the Federal Reserve System
International Finance Discussion Papers
Number 817, August 2004, revised: October 2004 --- Screen Reader Version*
The Performance of International Equity Portfolios
NOTE: International Finance Discussion Papers are preliminary materials circulated to stimulate discussion and critical comment. References in publications to International Finance Discussion Papers (other than an acknowledgment that the writer has had access to unpublished material) should be cleared with the author or authors. Recent IFDPs are available on the Web at http://www.federalreserve.gov/pubs/ifdp/. This paper can be downloaded without charge from the Social Science Research Network electronic library at http://www.ssrn.com/.
Abstract:
This paper evaluates the performance of U.S. investors' portfolios in the equities of over 40 countries over a 25-year period. We find that these portfolios achieved a significantly higher Sharpe ratio than foreign benchmarks, especially since 1990. We uncover three potential reasons for this success. First, U.S. investors abstained from momentum trading and instead sold past winners. Second, conditional performance tests provide no evidence that the superior (unconditional) performance owed to private information, suggesting that the successful exploitation of publicly available information played a role. Third, the documented preference for cross-listed and well-governed foreign firms appears to have served U.S. investors well. We conclude with a short discussion of the implications of our findings for the home bias literature.
Keywords: home bias, momentum, contrarian, conditional performance measures, equities, bonds
JEL classification: G11, G12, F21
*The authors are in the International Finance Division of the Federal Reserve Board. Thomas is Chief of the International Financial Transactions Section; Warnock and Wongswan are staff economists. Warnock is also Visiting Associate Professor at the Darden Graduate School of Business Administration, University of Virginia. This paper is a condensed and revised version of Thomas, Warnock, and Wongswan (2004). The authors thank Jillian Faucette, Tamara Hayford, Sara Holland, and Sarita Subramanian for research assistance. Mark Carey, Charlie Hadlock, Michael Schill, and participants at the IF Monday Workshop and Darden�s Finance Seminar provided helpful comments. The views in this paper are solely the responsibility of the authors and should not be interpreted as reflecting the views of the Board of Governors of the Federal Reserve System or of any other person associated with the Federal Reserve System. email: [email protected], [email protected], [email protected]. Return to text
1 Introduction
The performance literature is vast and spans several decades. Many researchers have evaluated the performance of recommendations, either by specific investment advisors (e.g., the Black (1971) and Copeland and Mayers (1982) studies of Value Line recommendations) or by a wide range of newsletters (Graham and Harvey, 1996; Metrick, 1999). The literature on mutual fund performance blossomed in the 1960s (Sharpe, 1966; Treynor and Mazuy, 1966; Jensen, 1969) and has yet to slow (Grinblatt, Titman, and Wermers, 1995; Ferson and Schadt, 1996; Becker, Ferson, Myers, and Schill, 1999). The performance of other types of investors, including pension funds (Lakonishok, Shleifer, and Vishny, 1992; Ferson and Khang, 2002), retail investors (Barber and Odean, 2000), and insiders (Eckbo and Smith, 1999), has also been thoroughly documented. All of these studies, and the many others that they cite, share a common theme: They analyze the performance of domestic investors' domestic portfolios.
In sharp contrast, the literature on the performance of international investors' portfolios is sparse, even though cross-border trading has skyrocketed in the past few decades.1 The most prominent published study is Cumby and Glen (1990), which finds that mutual funds performed poorly in 14 foreign markets, although not in a statistically significant sense. Two other studies in this relatively underdeveloped literature focus on the performance of U.K. fund managers: Shukla and van Inwegen (1995) find that their U.S. equity portfolios underperform domestic ones, while Blake and Timmermann (2004) expand the destination markets to four major regions and find similarly poor performance. Another literature that is tangentially related to performance includes studies of the relationship between international capital flows and returns (e.g., Bohn and Tesar,1996).2
In this paper we aim to fill the void in the international performance literature by evaluating the performance of the largest group of international equity investors in the world, U.S. investors, over a 25-year period. By the end of our sample, these investors had a foreign equity portfolio of nearly $2 trillion, or roughly half the holdings of all foreign investors in non-U.S. markets. Our analysis of the performance and trading strategy of such a large group of international investors will add not only to the performance literature but can also shed light on other subjects, such as the home bias in equity holdings and the role of global investors in emerging market crises.
Perhaps surprisingly, we find that compared to global benchmarks U.S. investors' foreign equity portfolios earned substantially higher Sharpe ratios. This performance is particularly evident since 1990 and occurs in both emerging and developed markets. We investigate three non-mutually exclusive reasons for the strong unconditional performance: trading strategy, private information, and preferences for two types of foreign firms--well-governed and cross-listed--that have alleviated information asymmetries.
The first piece of evidence comes from a characterization of the trading strategy employed by U.S. investors. This characterization--implemented using the Badrinath and Wahal (2002) and Ferson and Khang (2002) refinement of the Grinblatt, Titman, and Wermers (1995) methodology-- is important in its own right, as it is the first time that all U.S. investors' international trading strategy has been analyzed. In the current context, it is important because Choe, Kho, and Stulz (2004) find that foreigners' penchant for momentum trading hampers their performance. In contrast, we find little evidence of momentum trading, but very strong evidence that U.S. investors can be characterized as contrarian, especially when selling. This characterization is apparent in both developed and emerging markets and suggests that the superior performance that we document could owe to a tendency to sell past winners. It also suggests that U.S. investors as a whole do not exacerbate crises; we find no evidence of a tendency to sell past losers.
Our second investigation indicates that U.S. investors appear to have successfully traded on publicly available information. Specifically, the conditional returns-based and weight-based performance measures of Grinblatt and Titman (1993), Eckbo and Smith (1998), and Ferson and Khang (2002), which are designed to detect whether private information produced superior performance, provide no indication of superior conditional performance.
The third possible cause for the superior performance is U.S. investors' preference for cross-listed and well-governed foreign firms (Ammer, Holland, Smith, and Warnock, 2004; Lins and Warnock, 2004). Foreign firms that are cross-listed or have better expected governance have higher value, so the superior performance that we document could owe to the fact that these firms have greater weights in U.S. portfolios than in global benchmark indexes.3 We show that cross-listed and governance portfolios also performed significantly better than the MSCI index, suggesting that U.S. performance owes in part to the preference for these types of firms.
While much of our paper focuses on foreign portfolios, we also analyze the performance of U.S. investors' global portfolios of U.S. and non-U.S. equities. Owing to the relatively small weight of foreign securities in U.S. portfolios and to the fact that large equity markets tend to be highly correlated, this analysis is more or less reduced to a study of long swings in the dollar. When the dollar is appreciating, U.S. investors look smart as their portfolio, heavily tilted toward U.S. securities, outperforms market-capitalization-weighted global benchmarks. The opposite is true when the dollar is depreciating.
The main reason that the literature on international portfolio
performance is relatively underdeveloped is that the data are
difficult to obtain. Another major contribution of our paper is the
formation of U.S. investors' monthly holdings in over 40 equity
markets for the period from December 1976 to December 2003. The
bilateral holdings data provide the country weights in U.S.
investors' portfolios; armed with these weights, and assuming that
within each country the market is held, we are able to compute the
(unhedged) foreign returns earned by U.S. investors.4![]() 5
5
Our paper proceeds as follows. In the next section we present monthly estimates of U.S. investors' equity portfolios in over 40 countries over a 27-year period. In Section 3 we analyze the unconditional performance of U.S. investors' portfolio of foreign equities. In Section 4 we investigate causes of the superior unconditional performance by characterizing U.S. investors' trading strategy; examining the role of private information using conditional returns-based and weight-based performance measures; and comparing the performance of U.S. portfolios with those of well-governed and cross-listed foreign firms. In Section 5 we analyze U.S. investors' global portfolios of U.S. and non-U.S. equities. In Section 6 we present concluding remarks and discuss the possible implications of our results for the home bias in international portfolios. Details on the methodologies used to form portfolio weights, characterize trading strategies, and evaluate conditional performance are included in appendices.
2 U.S. Investors' International Equity Portfolios
We use publicly available data to create monthly estimates of U.S. investors' holdings of equities in 44 countries for the period from December 1976 to December 2003.6 The underlying data and the methodology are discussed in detail in Appendix A. Briefly, our methodology involves adding capital flows and valuation adjustments to a past known holdings amount (from an infrequent benchmark survey) to form naive baseline estimates.7 These naive estimates, as we show below, are in many cases inaccurate, primarily because of the financial center bias in the capital flows data (Warnock and Cleaver, 2003). The benchmark survey data do not suffer from this bias, so we then adjust the capital flows to ensure that our holdings estimates are consistent with the next known holdings amount (from the next benchmark survey); the resulting holdings data are our benchmark-consistent holdings estimates. For selected countries, our naive (thin lines) and benchmark-consistent (thick lines) holdings estimates are depicted in Figures 1(a) - 1(g) for the period January 1977 - December 2003, with benchmark survey dates shown as the vertical lines at March 1994, December 1997, and December 2001. Estimates that postdate the last benchmark survey should be viewed as preliminary and are subject to substantial revisions after a new benchmark becomes available; accordingly, they will not be utilized in our performance analysis.
As shown in Figure 1a, naive estimates understated U.S. positions in foreign equities as of the 1994 and 1997 benchmarks by 36 and 20 percent, respectively. These discrepancies can mask large, offsetting errors in bilateral positions. For example, as of end-2001 the naive estimate of holdings of U.K. securities (Figure 1b) was 19 percent too low while the estimate of holdings of Canadian equities (Figure 1e) was 34 percent too high. From this point on we discard the naive estimates and proceed to analyze the benchmark-consistent estimates.
Table 1, which provides a complete list of all countries for which we estimate U.S. investors' holdings, shows the country weights in U.S. investors' foreign equity portfolios for selected years. For comparison purposes, we also include the composition of a benchmark portfolio, the MSCI World ex US. Not surprisingly, U.S. investors' foreign equity positions are heavily weighted toward developed countries. The developed country weight was over 90 percent in 1989, but fell to roughly 80 percent by the mid-1990s as emerging equity markets liberalized and became a larger portion of the global equity market. Emerging markets then shrank in both the MSCI and U.S. portfolios with the crises of the late 1990s.
A comparison of the composition of the MSCI World ex US and U.S. investors' foreign portfolios shows that U.S. investors were roughly in line with the benchmark allocations for developed and emerging markets. Within these groups, however, there were sizeable deviations from benchmark weightings. For example, European countries were overweighted, especially in the earlier years, but less so at the end of the sample. Within Europe, early in the sample U.S. investors were particularly overweight the U.K. and the Netherlands. By the middle of the sample the Netherlands was still overweight but the U.K. less so as emphasis shifted to Scandinavian equities. In contrast to the overweighting of European markets, U.S. investors have maintained a substantial underweighting of Japanese equities throughout this period.
Within emerging markets, long-standing over- or underweightings are less apparent, with the exception of consistently large positions in Mexico and, to a lesser extent, Hong Kong. The norm in emerging markets appears to be country allocations that change substantially over time. For example, early in the sample U.S. investors were substantially underweight Korea and Taiwan, but that ended near the end of the sample. That U.S. investors' deviations from benchmark weightings on particular emerging markets change rather rapidly could owe to underlying characteristics of emerging markets or to the fact that U.S. investors are large relative to these markets.
3 Performance Analysis of Foreign Equity Portfolios
In this section we investigate the unconditional performance of U.S. investors' portfolios of non-U.S. equities. Because our holdings estimates that postdate the last benchmark asset survey are subject to substantial revisions, for all of the performance analysis in this paper we end the sample at December 2001.
We test the unconditional performance of U.S. investors' foreign equity portfolios by asking the simple question: Did U.S. investors choose the right markets when venturing abroad? To gauge this we compare returns of the foreign portfolio of U.S. investors--the composition of which changes month-to-month--to returns of value-weighted benchmark portfolios. Not having time-series data on security-level holdings within countries, we perform our analysis at the country level and implicitly assume that the composition of U.S. investors' holdings in each country is similar to the composition of the country's MSCI index. This simplification--imposed out of necessity--implies that we do not evaluate within country stock-picking ability, but rather the cross-country allocations.
Our unconditional performance analysis (Table 2) provides
evidence that, within their foreign equity portfolios, U.S.
investors exhibited skill in reallocating across markets,
especially after 1989. This skill is not evident in a statistically
significant sense over the full sample from 1977 to 2001 (Panel A).
U.S. investors' foreign portfolios earned a higher Sharpe ratio
than the value-weighted foreign benchmark (11.3% vs. 9.1%), but the
difference is not statistically significant; the p-value of a
![]() test of the
difference in the Sharpe ratios is 0.239. When we split the sample
(Panel B), we see that the Sharpe ratios were also nearly identical
in the 1977 - 1989 period. However, in the 1990 - 2001 period U.S.
investors' foreign equity portfolio produced a significantly higher
Sharpe ratio than the value-weighted benchmark. This superior
performance was obtained through much higher average excess
returns--positive 0.13 percent per month versus negative 0.11
percent--and less risk.
test of the
difference in the Sharpe ratios is 0.239. When we split the sample
(Panel B), we see that the Sharpe ratios were also nearly identical
in the 1977 - 1989 period. However, in the 1990 - 2001 period U.S.
investors' foreign equity portfolio produced a significantly higher
Sharpe ratio than the value-weighted benchmark. This superior
performance was obtained through much higher average excess
returns--positive 0.13 percent per month versus negative 0.11
percent--and less risk.
Panel C compares equity investment performance in different groups of countries. Because most emerging markets became important in global investment in the early 1990s--and because we have more faith in our holdings estimates that are relatively close to benchmark surveys--we focus on the period from 1990 onward. In each of the two country groups, we restrict the investment strategy to contain only assets in that group and reweight the asset allocation within a group to sum to one. The results on investment in developed and emerging markets are qualitatively similar to the aggregate results: Over this period U.S. investors' foreign equity portfolios produced significantly higher Sharpe ratios than the value-weighted foreign portfolios.8 In the next section, we attempt to ascertain the source of this strong performance.
4 Reasons for the Superior Unconditional Performance
U.S. investors earned higher Sharpe ratios than foreign benchmarks, especially since 1990. In this section we explore three non-mutually exclusive reasons for the superior (unconditional) performance: the trading strategy employed, the skillful use of publicly available information, and a preference for certain types of foreign firms that performed well over a long span of time.
4.1 Trading Strategy
The standard presumption in the international finance literature is that investors are at an informational disadvantage when they venture abroad. This view is based in part on empirical studies that have found that foreigners perform poorly when investing in countries ranging from Indonesia (Dvorak, 2004) and Korea (Choe, Kho, and Stulz, 2001) to Germany (Hau, 2001). In the theoretical model of Brennan and Cao (1997), the informational disadvantage results in returns-chasing behavior, which Choe, Kho, and Stulz (2004) argue leads to poor portfolio performance. Was the good performance we documented in spite of returns-chasing behavior, or did U.S. investors follow another trading strategy?
We describe the trading strategy of U.S. investors using the
Ferson and Khang (2002) and Badrinath and Wahal (2002) refinement
of the Grinblatt, Titman, and Wermers (1995) methodology (details
are in Appendix B). Briefly, the overall momentum statistic, LM, is
intended to measure the degree to which U.S. investors actively
change their portfolio holdings in the direction of the past
![]() periods' stock
returns. A significantly positive (negative) value of LM would
constitute evidence of a momentum (contrarian) trading
strategy.9 Because
investors may exhibit different styles when increasing and
decreasing country weights--perhaps aggressively increasing the
weights on past winners while not showing evidence of any specific
trading style when reducing country weights--we also compute BM
(Buy Only) and SM (Sell Only) statistics. The BM statistic will
indicate whether momentum trading is evident when investors
increase country weights; SM applies when investors decrease
country weights.
periods' stock
returns. A significantly positive (negative) value of LM would
constitute evidence of a momentum (contrarian) trading
strategy.9 Because
investors may exhibit different styles when increasing and
decreasing country weights--perhaps aggressively increasing the
weights on past winners while not showing evidence of any specific
trading style when reducing country weights--we also compute BM
(Buy Only) and SM (Sell Only) statistics. The BM statistic will
indicate whether momentum trading is evident when investors
increase country weights; SM applies when investors decrease
country weights.
Panel A of Table 3 shows results for the momentum measures using
the past one-, two-, and three-month returns for the full sample
(from 1977) and two subsamples (1977 - 1989 and 1990 - 2001). Very
few of the LM (Buy and Sell) coefficients are significant,
indicating that by this metric when U.S. investors venture abroad
their trading strategy cannot be characterized as momentum
following or contrarian. When we focus on instances in which U.S.
investors increased the portfolio weight on country ![]() (BM Buy Only), we again see very
little evidence of momentum trading; the coefficients on the BM
statistic are usually positive, indicating that U.S. investors
moved into markets that recently performed well, but the statistic
is almost never significant. In contrast, almost all of the SM
(Sell Only) coefficients are negative and significant, especially
in the full and more recent samples, indicating that U.S. investors
exhibit a contrarian strategy when selling; that is, they sell past
winners.
(BM Buy Only), we again see very
little evidence of momentum trading; the coefficients on the BM
statistic are usually positive, indicating that U.S. investors
moved into markets that recently performed well, but the statistic
is almost never significant. In contrast, almost all of the SM
(Sell Only) coefficients are negative and significant, especially
in the full and more recent samples, indicating that U.S. investors
exhibit a contrarian strategy when selling; that is, they sell past
winners.
In Panel B, we separate trading in developed and emerging markets for the 1990 - 2001 period. Again, the evidence points clearly to a tendency to sell past winners. In both developed and emerging markets, most of the SM (Sell Only)statistics are significant and all are negative, more so for emerging markets. As in Panel A, evidence of momentum trading is scant: Of the 18 cells in Panel B, only one has a positive and significant coefficient, the BM (Buy Only) at lag 1 for emerging markets.
Our findings in this subsection are consistent with Badrinath and Wahal (2002), who find that institutional investors follow a contrarian strategy in the U.S. market when liquidating or adjusting existing positions. Interestingly, they find that the effect is more pronounced in small and volatile firms, which is consistent with our finding of stronger evidence of contrarian trading in emerging markets (or that foreign stocks are viewed as being similar to small domestic stocks).10 Our results imply that the factor Choe, Kho, and Stulz (2004) highlight as a root cause of poor performance of foreigners--returns-chasing behavior--does not appear to be evident in our sample.
4.2 The Role of Private Information: Conditional Portfolio Performance Evaluation
U.S. investors' portfolios earned higher Sharpe ratios than global benchmarks, perhaps because of their strategy of selling past winners. It is also possible that the superior performance owed to private information or, failing that, to skill at using publicly available information. In this section, we analyze the performance of U.S. investors by accounting for the predictability in international returns documented in Ferson and Harvey (1993) and Harvey (1995).11 In other words, we ask the question of whether U.S. investors have private information that enables them to move into (out of) markets before returns are higher (lower) than anticipated from using publicly available information. If U.S. investors based their trading strategy solely on public information, the conditional measures in this section will show no evidence of superior performance. We use two types of performance evaluation methods. The first is a conditional returns-based measure (CRM) that evaluates the conditional Jensen's alpha--the abnormal returns of U.S. investors' portfolio over a benchmark factor model. The second is a conditional weight-based measure (CWM), which does not rely on an asset pricing model (Grinblatt and Titman, 1990,1993; Eckbo and Smith, 1998; and Ferson and Khang, 2002). Complete details on both measures are provided in Appendix C.
4.2.1 Conditional returns-based measure
The basic intuition behind the CRM is to assume a conditional asset pricing model and estimate it with an intercept term, the conditional Jensen's alpha. A significantly positive intercept term would be evidence of superior performance that owed to private information about future returns beyond what can be exploited from public information, whereas a portfolio strategy that relied only on public information would produce an insignificant intercept term, or zero conditional Jensen's alpha.
The returns-based measure requires a stand on an asset pricing model. With no general consensus about the ``correct'' international asset pricing model, we utilize three widely used models. The first is the conditional global version of the CAPM with the world market portfolio as a factor.12Second, as Fama and French (1998) find that the one-factor world CAPM fails to explain the value premium in the global equity markets (that is, average returns on a high book-to-market portfolio are higher than average returns on a low book-to-market portfolio), we use a two-factor model that includes the world market portfolio and the difference between returns on a global portfolio of high book-to-market and low book-to-market firms (HML).13 Lastly, Solnik (1974) and Adler and Dumas (1983) illustrate that when purchasing power parity does not hold, in addition to the world market portfolio, foreign exchange risk will be priced in financial markets. We proxy for foreign exchange risk with the excess returns from investing in foreign currencies. In principal, we should include as many currencies as we have different foreign assets. However, for tractability reasons, we only use excess returns deposited in euro (Deutsche mark before January 1999), sterling, and yen; see, e.g., Dumas and Solnik (1995) and De Santis and Gerard (1998).14
Table 4 reports estimates of the conditional Jensen's alpha,
![]() ,under the
different factor pricing model specifications and under both
time-varying and constant betas. Panel A shows estimates for the
full sample (1977-2001). In the left side of the panel, we use
three different factor pricing models and allow beta to be
time-varying. For the full sample, the CRW measure is positive but
not significant, indicating that we do not find evidence of
superior conditional performance. Specifically, we fail to reject
the null hypothesis of no superior conditional performance, because
Jensen's alpha is not significantly different from zero. In
addition, we find that the time-varying risk premium is an
important factor in explaining time-varying expected returns; the
highly significant
,under the
different factor pricing model specifications and under both
time-varying and constant betas. Panel A shows estimates for the
full sample (1977-2001). In the left side of the panel, we use
three different factor pricing models and allow beta to be
time-varying. For the full sample, the CRW measure is positive but
not significant, indicating that we do not find evidence of
superior conditional performance. Specifically, we fail to reject
the null hypothesis of no superior conditional performance, because
Jensen's alpha is not significantly different from zero. In
addition, we find that the time-varying risk premium is an
important factor in explaining time-varying expected returns; the
highly significant ![]() test statistic (21.5) indicates a strong rejection of the null that
the estimates in the vector
test statistic (21.5) indicates a strong rejection of the null that
the estimates in the vector ![]() in equation (C2), except the constant, are
jointly insignificant. In the right half of the panel, we use the
same factor models but, because we tested for and found little
evidence of time-varying betas, constrain beta to be constant. The
results--with positive but insignificant Jensen's alphas--are
qualitatively similar to the results with time-varying betas.
in equation (C2), except the constant, are
jointly insignificant. In the right half of the panel, we use the
same factor models but, because we tested for and found little
evidence of time-varying betas, constrain beta to be constant. The
results--with positive but insignificant Jensen's alphas--are
qualitatively similar to the results with time-varying betas.
As mentioned earlier, most emerging markets became accessible to international investors in the early 1990s. Thus, to evaluate the performance before and after U.S. investors expanded their opportunity set to include emerging markets, we investigate in Panel B two subsamples, one pre-1990 and the other from 1990 to 2001.15 For both time-varying and constant betas, the results show that skill in the early period was followed by poor performance in the more recent period, although in neither period is the performance measure statistically significant. We also fail to reject the null hypothesis of equal, insignificant Jensen's alphas for the samples before and after 1990. Panel C shows estimates of Jensen's alpha for portfolios of developed and emerging markets from 1990, re-weighting country weights in each portfolio to sum to one. Again, we find no evidence of superior performance by U.S. investors in global equity markets; Jensen's alphas are generally negative for developed markets and positive for emerging markets, but insignificant throughout. In all samples, we tested for and found little evidence of time-varying betas, so we re-estimated constraining beta to be constant; the results are qualitatively similar.
4.2.2 Conditional portfolio weight-based measure
Because the returns-based performance measure is a joint test of investor performance and the underlying assumed asset pricing model, evidence of superior performance could come from truly superior information, failure of an asset pricing model, or both. Grinblatt and Titman (1989, 1993) propose a portfolio weight-based measure that does not rely on an assumption about an asset pricing model.16 They show in the case of constant expected returns that an investor who has private information and uses that information to reallocate his portfolio weight would generate a positive estimate of the sum of the covariances between changes in portfolio weight and future abnormal returns, above a constant expected return, given a nonincreasing absolute risk aversion preference as defined in Rubinstein (1973). Such an investor would move into a market when private information indicated positive future abnormal returns and out of a market in which private information indicated negative future abnormal returns. Eckbo and Smith (1998) and Ferson and Khang (2002) extend this framework to allow for time-varying expected returns. Under time-varying expected returns, an investor would move into (out of) the market when private information indicates a positive (negative) abnormal return--above an expected return predicted from using public information. Evidence of private information would be a positive estimate of the sum of the conditional covariances between changes in portfolio weight and future abnormal returns.
Table 5 shows estimates of the average
conditional portfolio weight measure, ![]() , estimated from the system
of equations (C9) and (C10). The top panel shows estimates of CWM
for the full sample period against one-, two-, and three-month
benchmark buy-and-hold strategies (
, estimated from the system
of equations (C9) and (C10). The top panel shows estimates of CWM
for the full sample period against one-, two-, and three-month
benchmark buy-and-hold strategies (![]() =1, 2, 3, respectively). The CWM is always positive,
but never significant. That is, we find no evidence of superior
conditional performance by U.S. investors, implying that U.S.
investors do not have private information about future returns
above what is available publicly. The results in the bottom panels
are qualitatively similar. CWM is higher in the more recent period,
but not significantly so; similar to the results for the full
sample, we find no evidence of superior conditional performance in
the early or more recent period (Panel B). For portfolios of
developed and emerging markets (Panel C) from 1990, we again find
no evidence of superior conditional performance.
=1, 2, 3, respectively). The CWM is always positive,
but never significant. That is, we find no evidence of superior
conditional performance by U.S. investors, implying that U.S.
investors do not have private information about future returns
above what is available publicly. The results in the bottom panels
are qualitatively similar. CWM is higher in the more recent period,
but not significantly so; similar to the results for the full
sample, we find no evidence of superior conditional performance in
the early or more recent period (Panel B). For portfolios of
developed and emerging markets (Panel C) from 1990, we again find
no evidence of superior conditional performance.
In summary, the evidence in this subsection does not suggest that U.S. investors utilized private information to obtain the high Sharpe ratios documented in Section 3. Thus, one can conclude that the superior unconditional performance owes at least in part to the successful processing of publicly available information.
4.3 Preferences for Cross-Listed and Well-Governed Foreign Firms
The superior unconditional performance we documented could have owed to active reallocations between foreign markets from month to month or, alternatively, to a general preference for certain stocks or countries that performed well ex post. U.S. investors exhibit a preference for cross-listed firms (Ammer et al., 2004) and firms that do not have poor expected corporate governance (Lins and Warnock, 2004), and these preferences are also evident in their country allocations (Ahearne et al., 2004; Dahlquist et al., 2003). Foreign firms that are cross-listed or have better expected governance have higher value, so the superior performance that we document could owe to the fact that these firms have greater weights in U.S. portfolios than in global benchmark indexes.17
Data limitations preclude us from rigorously testing whether the high Sharpe ratio obtained by U.S. investors' foreign equity portfolios owed to their preference for cross-listed or well-governed firms. However, we can conduct a simple comparison of the performance of two portfolios, which we call Governance and Cross-Listed, with the performance of U.S. investors' and MSCI portfolios. Firms whose shares are closely held are more likely to have agency and entrenchment problems that erode shareholder value, so dispersed ownership is one proxy for good corporate governance (Claessens, Djankov, and Lang, 2000; Faccio and Lang, 2002; Lins, 2003). Hence, we form the governance portfolio by using (one minus) the Dahlquist et al. (2003) country-level measure of insider holdings. Similarly, the cross-listed portfolio is formed using the Ahearne et al. (2004) data on the percent of each foreign market that is available on a U.S. exchange. Because time-varying measures of insider holdings and cross-listings are not readily available, we form these portfolios using fixed, end-1997 weights.
The first two columns of Table 6 reproduce numbers from Table 2. As noted, over the period from 1990 to 2001, U.S. investors outperformed the MSCI index by earning higher returns with less risk. The governance and cross-listed portfolios (columns c and d) performed even better, cross-listed significantly so. Thus, it would appear that by overweighting foreign firms that are cross-listed and have good corporate governance--and, thus, overweighting foreign countries whose firms tend to cross-list or be well governed--U.S. investors were able to obtain superior portfolio results.18 We take the evidence in Table 6 as providing partial support for the notion that the superior performance we have documented owes at least in part to U.S. investors' preferences for a subset of foreign firms (cross-listed and well-governed ones) that have less severe information asymmetries and performed better than the world value-weighted portfolio.
5 The Performance of Global Equity Portfolios
While the focus of our study is on foreign portfolios, in this section we briefly analyze the performance of U.S. investors' global portfolios of U.S. and foreign equities. As Figure 2 shows, U.S. portfolios are heavily tilted toward U.S. equities. As we will show, in a world with home bias and high correlations between major equity markets, global performance owes first and foremost to long swings in the exchange value of the dollar.
We begin with some background information. Columns (a)-(c) of Table 7 report the performance of value-weighted benchmarks. These columns indicate whether--from the perspective of a U.S. investor--foreign markets provided higher risk-adjusted returns than U.S. markets. Foreign returns in column (b) are comprised of monthly (unhedged) excess returns in 44 countries; global returns (column c) are a value-weighted average of these 44 markets and the U.S. market.
Equity market performance over the 1977 - 2001 period (Panel A) was quite similar in the U.S. and abroad, with the Sharpe ratio on U.S. stocks (12.5%) only slightly higher than on foreign stocks (9.1%). This similarity masks considerable differences in subsamples (Panel B): In dollar terms, foreign stocks outperformed U.S. stocks in the 1977-1989 period (Sharpe ratio of 19.5% vs. 9.2%) by providing much higher returns at comparable risk. In contrast, U.S. markets produced a much higher Sharpe ratio in the 1990-2001 period (16.3% vs. -2.2%), when foreign stocks had negative excess returns and higher volatility.
Column (d) of Table 6 reports statistics on the global
portfolios of U.S. investors. In these portfolios, which are
comprised of securities from the same 44 foreign markets in column
(c) as well as U.S. securities, the weights change monthly as U.S.
investors reallocate across countries. A comparison of columns (c)
and (d) shows that over the period from 1977 to 2001 U.S.
investors' global equity portfolios performed better than the
value-weighted global portfolio, but not significantly so; the
p-value of a ![]() test
of a difference between the Sharpe ratios is 0.698. Given the very
low weight of foreign securities in U.S. portfolios and high
correlations between major equity markets, this result was
foreshadowed by the relative performance of the value-weighted
benchmarks. Over the entire sample, U.S. equity markets performed
slightly better than foreign equity markets; thus, U.S. investors'
global equity portfolios--heavily weighted toward U.S.
equities--performed slightly better than the global benchmark.
Similarly, in the pre-1990 period when foreign markets performed
better than the U.S. market, U.S. investors' global portfolios
underperformed the global benchmark (Sharpe of 0.095, compared to
0.165 for the global benchmark), although the difference in Sharpe
ratios is not statistically significant. In the more recent period
(1990-2001), U.S. markets outperformed and U.S. investors' global
portfolios had a Sharpe ratio of 0.152, significantly greater than
the Sharpe ratio of the global market portfolio (0.044).
test
of a difference between the Sharpe ratios is 0.698. Given the very
low weight of foreign securities in U.S. portfolios and high
correlations between major equity markets, this result was
foreshadowed by the relative performance of the value-weighted
benchmarks. Over the entire sample, U.S. equity markets performed
slightly better than foreign equity markets; thus, U.S. investors'
global equity portfolios--heavily weighted toward U.S.
equities--performed slightly better than the global benchmark.
Similarly, in the pre-1990 period when foreign markets performed
better than the U.S. market, U.S. investors' global portfolios
underperformed the global benchmark (Sharpe of 0.095, compared to
0.165 for the global benchmark), although the difference in Sharpe
ratios is not statistically significant. In the more recent period
(1990-2001), U.S. markets outperformed and U.S. investors' global
portfolios had a Sharpe ratio of 0.152, significantly greater than
the Sharpe ratio of the global market portfolio (0.044).
Figure 3a depicts the evidence for equity portfolios in a different way. The solid line shows the difference, since end-December 1976, between the total excess return on U.S. residents' holdings of U.S. equities and the total excess return on U.S. residents' holdings of foreign equities (expressed as a percent of the total excess return to holding U.S. equities).19 When this measure is increasing, as occurred in the early 1980s and for most of the 1990s, U.S. investors earned higher rates of return on their domestic portfolios than they did on their foreign portfolios. When the measure is decreasing, as occurred in the late 1970s and late 1980s, foreign returns are stronger than U.S. returns. The fact that the line ends near zero indicates that over the entire period U.S. investors earned similar rates of return on their foreign and U.S. equity portfolios.
The dashed line in Figure 3a depicts an asset-weighted index of the exchange value of the dollar, formed by aggregating bilateral exchange rates according to countries' weights in U.S. investors' foreign equity portfolio.20 An increase in the dollar index represents dollar appreciation. The sample correlation between the dollar index and the relative returns differentials is positive 0.70, indicating that the relative performance of foreign and U.S. equities owes significantly to movements in the dollar. From the perspective of a U.S. investors, when the dollar appreciates--as in the early 1980s and late 1990s--U.S. markets outperform. When the dollar depreciates--as in the late 1970s and late 1980s--the foreign portfolio performs much better. This is not terribly surprising, but suggests that the relative performance of U.S. investors' global equity portfolios owes importantly to long swings in the dollar.
Figure 3b provides yet another way of looking at our full sample results. The figure shows the Sharpe ratio for portfolios comprised of U.S. and foreign equities. The foreign component is formed by aggregating country returns using two different (time-varying) weighting schemes: a benchmark scheme that uses market weights (dotted line) and a scheme using the country weights in U.S. investors' foreign equity portfolios (solid line). A portfolio of all U.S. equities and a zero weight on foreign equities is represented at the left axis. The maximum point on each line, indicated by a triangle, represents the maximal Sharpe ratio attainable by mixing U.S. equities with the benchmark (market-cap-weighted) and U.S. investors' foreign portfolios. Consistent with our statistical tests, the figure shows evidence of skill in choosing country weights within the foreign portfolios: The Sharpe ratios of portfolios formed with country weights from U.S. investors' foreign portfolios (solid line) are everywhere greater than those from value-weighted foreign portfolios (dashed line). That said, there is evidence of unexploited international diversification benefits; U.S. investors attained a Sharpe ratio of about 0.121 on their global portfolio (not shown), but could have done better had they the (ex post) optimal weight of foreign equities of about 23 percent in a value-weighted benchmark portfolio or 40 percent using their foreign portfolios.
6 Conclusion
U.S. investors substantially increased the weight of foreign equities in their portfolios by the early 1990s, in time for a decade long surge in U.S. equity prices. Does this poor timing portend poor skill in choosing among investments abroad? Not necessarily. Our evidence indicates that while the movement into foreign markets was inopportune (ex post), U.S. investors exhibited skill in choosing the country composition within their foreign portfolios. In particular, the Sharpe ratio on U.S. investors' foreign equity portfolio is significantly greater than that on foreign benchmarks, especially since 1990. Subsequent analysis suggests that this superior (unconditional) skill owes to three non-mutually exclusive factors: the selling of past winners and avoidance of returns-chasing behavior, the successful use of publicly available information, and a preference for cross-listed and well-governed foreign firms.
Another important contribution of this paper is the formation of monthly bilateral estimates of U.S. investors' holding of the equities of 44 foreign countries for the period from December 1976 to December 2003. These holdings data should be useful for many other applications going forward. We showed one application here, the formation of a portfolio-weighted exchange rate index. Other applications include using the monthly bilateral holdings data in empirical models of exchange rate determination (Rogers and Warnock, 2004) and in descriptions of external adjustment (Freund and Warnock, 2004).
Our results have implications for the home bias literature. It is well known that foreign securities have only a small weight in investors' portfolios, but it is not known whether this weight is inordinately small, in part because we have not had a clear picture of the returns investors earn on their foreign portfolios. If investors are at a severe informational disadvantage when they venture abroad, the optimal weight on foreign securities would be far smaller than implied by standard metrics.21 One interpretation of the skill exhibited by U.S. investors that we document is that information asymmetries are not severe: If investors are at a substantial disadvantage when they venture abroad, it would be unlikely that U.S. investors could reallocate across foreign markets in a manner that would produce superior portfolio performance--even in an unconditional sense--over a full decade. A competing interpretation, for which we find some supporting evidence, is that information asymmetries may well be severe and U.S. investors performed well precisely by investing in the types of firms (cross-listed or well governed) that have alleviated barriers to international investors.
References
Adler, M., and B. Dumas, 1983. International portfolio choice and corporation finance: A synthesis. Journal of Finance 38, 925-984.
Ahearne, A., W. Griever, and F. Warnock, 2004. Information costs and home bias: an analysis of U.S. holdings of foreign equities. Journal of International Economics 62:313-336.
Ammer, J., S. Holland, D. Smith, and F. Warnock, 2004. Look at me now: The role of cross-listing in attracting U.S. shareholders. International Finance Discussion Paper 815, Board of Governors of the Federal Reserve System.
Ang, A., and G. Bekaert, 2002. Stock return predictability: Is it there? Working Paper, Columbia Business School
Badrinath, S.G., and S. Wahal, 2002. Momentum trading by institutions. Journal of Finance 57, 2449-2478.
Bansal R., and M. Dahlquist, 2000. The forward premium puzzle: Different tales from developed and emerging economies, Journal of International Economics 51, 115-144.
Bange M., K. Khang, and T. Miller, 2003. The performance of global portfolio recommendation. Working Paper University of South Carolina.
Barber, B., and T. Odean, 2000. Trading is hazardous to your wealth: The common stock investment performance of individual investors. Journal of Finance 55(2): 773-806.
Becker, C., W. Ferson, D. Myers, and M. Schill, 1999. Conditional market timing with benchmark investors. Journal of Financial Economics 52: 119-148.
Bekaert, G., and C.R. Harvey, 1997. Emerging equity market volatility. Journal of Financial Economics 43, 29-77.
Bekaert, G. , C.R. Harvey and C. Lundblad, 2003. Liquidity and expected returns: Lessons from emerging markets. Working Paper, Fuqua School of Business, Duke University.
Black, F., 1971. Yes, Virginia, there is hope: Test of the value line ranking system. University of Chicago.
Black, F., 1974. International capital market equilibrium with investment barriers. Journal of Financial Economics, 1.
Blake, D., and A. Timmermann, 2004. Returns from active management in international equity markets: Evidence from a panel of UK pension funds. Journal of Business (forthcoming).
Bohn, H., and L. Tesar, 1996. U.S. equity investment in foreign markets: Portfolio rebalancing or returns chasing? American Economic Review 86(2): 77-81.
Brennan, M., and H. Cao, 1997. International portfolio flows. Journal of Finance 52: 1851-1880.
Campbell, J.Y., and M. Yogo, 2003. Efficient tests of stock return predictability. NBER Working Paper 10026.
Cheung, Y., M. Chinn, and A. Pascual, 2004. Empirical exchange rate models of the nineties: Are any fit to survive? Journal of International Money and Finance (forthcoming).
Choe, H., C. Kho, and R. Stulz, 2001. Do domestic investors have more valuable information about individual stocks than foreign investors? NBER Working Paper 8073.
Choe, H., C. Kho, and R. Stulz, 2004. Do domestic investors have an edge? The trading experience of foreign investors in Korea. NBER Working Paper 10502.
Claessens, S., S. Djankov, and L.H.P. Lang, 2000. The separation of ownership and control in East Asian corporations. Journal of Financial Economics 58, 81-112.
Claessens, S., and J.P.H. Fan, 2002. Corporate governance in Asia: A survey. International Review of Finance 3, 71-103.
Connor, G. ,and R.A. Korajczyk, 1995. The arbitrage pricing theory and multifactor models of asset returns, in Robert Jarrow, Vojislav Maksimovic, and William T. Ziemba, eds: Finance, vol. 9 of Handbooks in Operations Reserach and Management Science (North-Holland, Amsterdam).
Copeland, T., and D. Mayers, 1982. The Value Line enigma (1965-1978): A case study of performance evaluation issues. Journal of Financial Economics 10: 289-321.
Cumby, R., and J. Glen 1990. Evaluating the performance of international mutual funds, Journal of Finance 45, 497-521.
Dahlquist, M., L. Pinkowitz, R. Stulz, and R. Williamson, 2003. Corporate governance and the home bias. Journal of Financial and Quantitative Analysis 38: 87-110.
Denis, D., and J. McConnell, 2003. International corporate governance.Journal of Financial and Quantitative Analysis 38, 1-36.
De Santis, G., and B. Gerard 1998. How big is the premium for currency risk?, Journal of Financial Economics 49, 375-412.
Doidge, C., G.A. Karolyi, and R. Stulz, 2004. Why are foreign firms listed in the U.S. worth more? Journal of Financial Economics 71, 205-238.
Domowitz, I., J. Glen, and A. Madhaven, 2001. Liquidity, volatility, and equity trading costs across countries and over time. International Finance, 4 (2), 221-255.
Dumas B., and B. Solnik 1995. The world price of foreign exchange risk,. Journal of Finance 50, 445-479
Dvorak, T., 2004. Do domestic investors have an information advantage? Evidence from Indonesia. Journal of Finance (forthcoming).
Eckbo, E., and D. Smith, 1998. The conditional performance of insider trades. Journal of Finance 53(2): 467-498.
Edison, H. J., and F. Warnock, 2004. U.S. investors' emerging market equity portfolios: A security-level analysis, Review of Economics and Statistics 84(3): 691-704.
Faccio, M., and L.H.P. Lang, 2002. The ultimate ownership of western European corporations. Journal of Financial Economics, 65, 365-395.
Fama, E. and K. French, 1998. Value versus growth: The international evidence. Journal of Finance 53, 1975-1999.
Faust, J., J. Rogers, and J. Wright, 2003. Exchange rate forecasting: The errors we've really made. Journal of International Economics 60: 35-60.
Ferson, W., and R.A. Korajczyk, 1995. Do arbitrage pricing models explain the predictability of stock returns?, Journal of Business 68, 309-349.
Ferson, W., and C. R. Harvey 1993, The risk and predictability of international equity returns. Review of Financial Studies 6, 527-566.
Ferson, W., and K. Khang, 2002. Conditional performance measurement using portfolio weights: evidence for pension funds. Journal of Financial Economics 65: 249-282.
Ferson, W., S. Sarkissian, and T. Simin, 2003. Spurious regressions in financial economics? Journal of Finance 58, 1393-1413.
Ferson, W., and R. Schadt, 1996. Measuring fund strategy and performance in changing economic conditions. Journal of Finance 51(2): 425-461.
Freund, C., and F. Warnock, 2004. Demystifying current account adjustments: A focus on the US. World Bank and FRB mimeo.
Froot, K., P. O'Connell, and M. Seasholes, 2001. The portfolio flows of international investors. Journal of Financial Economics 59: 151-193.
Gehrig, T., 1993. An information based explanation of the domestic bias in international equity investment. Scandinavian Journal of Economics 95: 97-109.
Glassman, D., and L. Riddick 2003. Market timing by global fund managers, Working Paper, American University.
Graham, J., and C. Harvey, 1996. Market timing ability and volatility implied in investment newsletters' asset allocation recommendations. Journal of Financial Economics 42: 397-422.
Griever, W., G. Lee, and F. Warnock, 2001. The U.S. system for measuring cross-border investment in securities: A primer with a discussion of recent developments. Federal Reserve Bulletin 87(10): 633-650.
Griffin, J., F. Nardari, and R. Stulz, 2004. Are daily cross-border flows pushed or pulled? Review of Economics and Statistics 86(3): 641-657.
Grinblatt, M., S. Titman, 1989. Portfolio performance evaluation: Old issues and new insights. Review of Financial Studies 2: 393-421
Grinblatt, M., S. Titman, 1993. Performance measurement without benchmarks: An examination of mutual fund returns. Journal of Business 60: 97-112.
Grinblatt, M., S. Titman, and R. Wermers, 1995. Momentum investment strategies, portfolio performance, and herding: a study of mutual fund behavior. American Economic Review 85(5): 1088-1105.
Hansen, L. 1982. Large Sample Properties of the Generalized Method of Moments Estimators, Econometrica 50, 1029-1054.
Harvey, C., 1991. The World Price of Covariance Risk, Journal of Finance 46, 111-157.
Harvey, C., 1995. Predictable Risk and Returns in Emerging Markets. Review of Financial Studies 8(3): 773-816.
Hau, H., 2001. Location matters: An examination of trading profits, Journal of Finance 56(5): 1951-1983.
International Monetary Fund, 2003. Coordinated Portfolio Investment Survey.
Jensen, M., 1969. Risk, the pricing of capital assets, and the evaluation of investment portfolios. Journal of Business 42: 167-247.
Kaminsky, G., R. Lyons, and S. Schmukler, 2004. Managers, investors, and crises: mutual fund strategies in emerging markets. Journal of International Economics (forthcoming).
Kang, J., and R. Stulz, 1997. Why is there a home bias? An analysis of foreign portfolio equity ownership in Japan. Journal of Financial Economics, 46:3-28.
Lakonishok, J., A. Shleifer, and R. Vishny, 1992. The impact of institutional trading on stock prices. Journal of Financial Economics 32(1): 23-43.
Lesmond, D., 2002. Liquidity of emerging markets. Journal of Financial Economics, forthcoming.
Lins, K., 2003. Equity ownership and firm value in emerging markets. Journal of Financial and Quantitative Analysis 38, 159-184.
Lins, K., and F. Warnock, 2004. Corporate governance and the shareholder base. International Finance Discussion Paper 816, Board of Governors of the Federal Reserve System.
Meese, R., and K. Rogoff, 1983. Empirical exchange rate models of the Seventies: Do they fit out of sample? Journal of International Economics 14: 3-24.
Merton, R., 1987. A simple model of capital market equilibrium with incomplete information. Journal of Finance 42: 483-510.
Metrick, A., 1999. Performance evaluation with transactions data: The stock selection of investment newsletters. Journal of Finance 54(5): 1743-1775.
Newey, W. K., and K. D. West, 1987. A simple, positive semi-definite, heteroskedasticity and autocorrelation consistent covariance matrix, Econometrica 55, 703-708.
Nguyen, E., 2002. The international investment position of the United States at year end 2002. Survey of Current Business 83(7): 12-21.
Rogers, J., and F. Warnock, 2004. Exchange rates and external asset positions. mimeo, Federal Reserve Board.
Rubinstein, M. 1973. A comparative statics analysis of risk premiums, Journal of Business 46, 605-615.
Sharpe, W., 1966. Mutual fund performance. Journal of Business 39: 119-138.
Shukla, R., and G. van Inwegen, 1995. Do locals perform better than foreigners? An analysis of UK and US mutual fund managers. Journal of Economics and Business 47: 241-254.
Solnik, B. 1974. An equilibrium model of the international capital market. Journal of Economic Theory 8, 500-524.
Stulz, R., 1981. On the effects of barriers to international investment. Journal of Finance, 36: 923-934.
Thomas, C., F. Warnock, and J. Wongswan, 2004. The performance of international portfolios. International Finance Discussion Paper 817, Board of Governors of the Federal Reserve System.
Treasury Department, Federal Reserve Bank of New York, and Federal Reserve Board, 2003. Report on United States Holdings of Foreign Securities as of December 31, 2001.
Treynor, J., and K. Mazuy, 1966. Can mutual funds outguess the market? Harvard Business Review 44: 131-136.
Warnock, F., and C. Cleaver, 2003. Financial centers and the geography of capital flows. International Finance 6(1): 27-59.
Willoughby, J., 1998. Executions song. Institutional Investor, 31(11), 51-56.
Appendix A. Creating Monthly Bilateral Equity Positions
Data Requirements
To create monthly estimates of U.S. investors' holdings of equities, we use data from infrequent benchmark surveys as well as data on capital flows, valuation adjustments, transaction costs, and merger-related stock swaps.
Bilateral capital flows. U.S. residents' foreign securities transactions have been reported monthly since January 1977 to the Treasury International Capital Reporting System (TIC), mainly by brokers and dealers. For foreign long-term securities, these mandatory reports contain information on gross purchases and gross sales (at market value); the country of the foreign counterparty to the transaction; and that the foreign security was an equity. For the purposes of estimating bilateral positions, there is geographic bias in the TIC data because the data indicate the countries through which U.S. residents purchase foreign securities, but not the residence of the issuer of the foreign security. It is commonly assumed that the transactor country is the same as the country in which the security's issuer is resident, but trades conducted through intermediaries in third countries, such as the financial centers of the United Kingdom and the Caribbean, violate this assumption. The TIC data are available at www.treas.gov/tic.
Benchmark asset surveys. Data on U.S. holdings of foreign securities, available at www.treas.gov/fpis, are collected in detailed but infrequent security-level benchmark asset surveys conducted in March 1994, December 1997, and December 2001.22 Reporting to the surveys is mandatory, with penalties for noncompliance, and the data received are subjected to extensive analysis and editing. For asset surveys (of U.S. holdings of foreign securities), the reporters consist mainly of large custodians and large institutional investors. Holdings of U.S. private investors are included to the extent they were through U.S. mutual funds or entrusted to U.S.-resident custodians for safekeeping. For our purposes, it is important to note that there is no geographical bias in the asset survey data; security-level identifiers (e.g, ISIN or SEDOL) provide information on the issuer's country of residence and ensure that the country attribution of the data is accurate.23
Valuation adjustments. Data availability for foreign equity indexes are depicted in Figure A1. We use country-level MSCI price return indexes, which are composed of large and liquid equities, the type of equities typically held by international investors (Kang and Stulz, 1997; Edison and Warnock, 2004; Ammer, Holland, Smith, and Warnock, 2004). For most emerging markets, MSCI equity data begin in December 1987; for these countries, prior to the MSCI starting date we rely on S&P/IFC Global returns.24
Transaction costs. The TIC data are reported gross at cost including commissions and taxes, so to compute the value of securities bought or sold, an adjustment for transaction costs must be made. For one-way transaction costs in equities, we use Elkins-McSherry estimates of commissions and fees charged institutional investors.25
Stock swaps. The TIC data do not include equities acquired through merger-related stock swaps. For example, when a foreign company acquires a U.S. firm, one form of financing the deal is an exchange of equity in which shareholders of the target (U.S.) firm are given stocks in the acquiring (foreign) firm. To continue with this example, if the acquisition of foreign stocks through swaps results in a greater-than-desired weighting on foreign stocks in U.S. equity portfolios, U.S. residents will sell foreign stocks to rebalance their portfolios, and such sales are reported to the TIC system. Since the TIC system does not capture the initial acquisition, but should capture associated sales, measures of stock swaps must be included in any analysis of asset holdings.26 Stock swaps swelled in importance in 1998 and 1999, when U.S. residents acquired over $100 billion each year in foreign stocks through swaps, due largely to the megamergers of Daimler Chrysler, BP Amoco, and Airtouch Vodafone.
Methodology
Naive baseline estimates
To form naive baseline estimates, we start from one benchmark
survey amount and use the Warnock and Cleaver (2003) methodology to
form monthly estimates through the date of the next benchmark
survey. End-of-month holdings are formed by adjusting the previous
month's holdings for estimated price and exchange rate changes and
adding the current month's (transaction cost-adjusted) net
purchases and equities acquired through stock swaps. Specifically,
we use the following formula to form naive estimates of U.S.
investors' holdings of country ![]() 's equities at the end of period
's equities at the end of period ![]() :
:
![]() (A1)
(A1)
where
nh
![]() naive
estimates of U.S. holdings of country
naive
estimates of U.S. holdings of country ![]() 's equities at the end of month
's equities at the end of month
![]()
r![]() returns
from period
returns
from period ![]() to
to
![]() , computed from
appropriate price indices
, computed from
appropriate price indices
gp
![]() gross
purchases of country
gross
purchases of country ![]() 's
equities by U.S. residents during month
's
equities by U.S. residents during month ![]()
gs
![]() gross sales
of country
gross sales
of country ![]() 's equities by
U.S. residents during month
's equities by
U.S. residents during month ![]()
tc
![]() a constant
adjustment factor for transaction costs in country
a constant
adjustment factor for transaction costs in country ![]()
ss
![]() country
country
![]() 's equities acquired by
U.S. residents through stock swaps during month
's equities acquired by
U.S. residents through stock swaps during month ![]()
The initial values of each nh![]() ,
holdings in country
,
holdings in country ![]() as of
December 1976, predate benchmark surveys and must be estimated. To
do so we assume that the country distribution of holdings from the
first asset survey (1994) is the same as the country distribution
in December 1976, and apply those shares to aggregate end-1976
holdings as published by the BEA.27
as of
December 1976, predate benchmark surveys and must be estimated. To
do so we assume that the country distribution of holdings from the
first asset survey (1994) is the same as the country distribution
in December 1976, and apply those shares to aggregate end-1976
holdings as published by the BEA.27
Benchmark-consistent estimates
Our benchmark-consistent estimates combine the naive baseline
estimates with holdings from the infrequent benchmark surveys. For
example, to form estimates for the April 1994 - November 1997
inter-survey period, we start from the March 1994 benchmark survey
amount and apply equation (A1) to form estimates to December 1997.
Doing so results in a naive estimate of holdings as of December
1997 (nh![]() that differs from holdings as given by the
benchmark survey (bh
that differs from holdings as given by the
benchmark survey (bh![]() by an amount, gap
by an amount, gap![]() :
:
![]() (A2)
(A2)
One candidate cause for the gap is errors in the capital flow
data. Assuming that such errors are larger in months with greater
trading activity, we add to each inter-survey month an amount that
is a function of the gap and the proportion of inter-survey trading
activity that occurred in that month.28 That is, we add to month ![]() 's net purchases of country
's net purchases of country
![]() 's securities an
adjustment given by:
's securities an
adjustment given by:
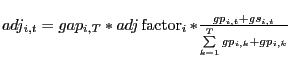 (A3)
(A3)
where periods ![]() and
and
![]() span the entire
inter-survey period. For each country (and each inter-survey
period), everything on the right side of (A3) is given except
adjfactor
span the entire
inter-survey period. For each country (and each inter-survey
period), everything on the right side of (A3) is given except
adjfactor![]() , which we choose to minimize the distance at time
, which we choose to minimize the distance at time
![]() between benchmark
holdings and our adjusted holdings estimates:
between benchmark
holdings and our adjusted holdings estimates:
![]() (A4)
(A4)
where our adjusted holdings estimates, ![]() , evolve according to
, evolve according to
![]() (A5)
(A5)
and, for all ![]() , we
impose a non-negativity constraint on our holdings estimates:
, we
impose a non-negativity constraint on our holdings estimates:
![]() (A6)
(A6)
Because the adjustment for any period ![]() must be part of the revaluation that
produces period
must be part of the revaluation that
produces period ![]() holdings (and so on), this is not a simple linear problem and,
accordingly, we employ a grid-search method to solve for the
adjustment factor.
holdings (and so on), this is not a simple linear problem and,
accordingly, we employ a grid-search method to solve for the
adjustment factor.
It is worthwhile to note three features of our adjustment
factor. First, it is both country-specific and
inter-survey-period-specific, so a country's adjustment factor is
independent of any other country's estimate and can differ across
inter-survey periods. Second, adjfactor![]() is constant for a given country and inter-survey
period, but the adjustment itself, adj
is constant for a given country and inter-survey
period, but the adjustment itself, adj![]() ,
is time-varying. Third, for the period after the last survey we
cannot form adjustment factors and so apply adjfactor
,
is time-varying. Third, for the period after the last survey we
cannot form adjustment factors and so apply adjfactor ![]() from the previous inter-survey period; to the
extent that the relationship of global financial centers and
capital flows changed after the last benchmark, our estimates that
post-date the most recent survey will incorporate the wrong
adjustment factors.
from the previous inter-survey period; to the
extent that the relationship of global financial centers and
capital flows changed after the last benchmark, our estimates that
post-date the most recent survey will incorporate the wrong
adjustment factors.
We form estimates for each country starting in December 1976. For some countries, however, we do not have complete source data. For example, the equity price data for the Philippines starts in 1985. Where we have no source data, we assume zero (e.g., flat returns). For returns, all such cases are indicated by white space in Figure A1. For our performance analysis, missing data are irrelevant because we bring each country into the analysis at the start date of its returns data.
Appendix B. Measures of Momentum Trading
To identify momentum trading strategies, we use the Ferson and
Khang (2002) and Badrinath and Wahal (2002) refinement of the
methodology of Grinblatt, Titman, and Wermers (1995). Define
![]() as the active
change in the weight of country
as the active
change in the weight of country ![]() in U.S. investors' foreign portfolio at time
in U.S. investors' foreign portfolio at time
![]() :
:
![]() (B1)
(B1)
where ![]() is the
return on country
is the
return on country ![]() equities from period
equities from period ![]() to
to
![]() ;
; ![]() is the return on U.S.
investors' foreign portfolio, defined as
is the return on U.S.
investors' foreign portfolio, defined as
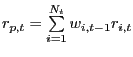 ; and
; and
![]() is the weight
of country
is the weight
of country ![]() at time
at time
![]() in U.S. investors'
portfolio. Note that for a buy-and-hold strategy
in U.S. investors'
portfolio. Note that for a buy-and-hold strategy ![]() equals zero. We compute the
following momentum or contrarian measure, LM, for lags of
equals zero. We compute the
following momentum or contrarian measure, LM, for lags of
![]() = 1, 2, and 3:
= 1, 2, and 3:
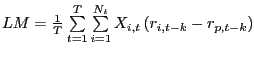 (B2)
(B2)
where ![]() is the
number of countries held in the portfolio at time
is the
number of countries held in the portfolio at time ![]() . A significantly positive (negative)
value of LM would constitute evidence of a momentum (contrarian)
trading strategy.
. A significantly positive (negative)
value of LM would constitute evidence of a momentum (contrarian)
trading strategy.
U.S. investors may follow momentum strategies only when buying or selling. To test this, we follow Grinblatt et al. (1995) and Badrinath and Wahal (2002) and jointly compute separate momentum statistics for buy and sell:
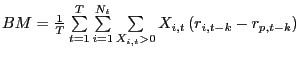 (B3)
(B3)
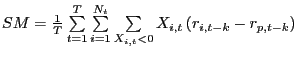 (B4)
(B4)
where BM (SM) is a measure of momentum when investors buy (sell) securities. In order to ensure that the buy and sell momentum statistics converge to zero under the null hypothesis of no momentum trading, we subtract total foreign portfolio returns from country returns.29 We estimate the momentum measures via generalized method of moments (GMM) for both the overall measure (B2) and jointly for BM (B3) and SM (B4).
Appendix C. Conditional Performance Measures
Conditional returns-based measure
Our implementation of a conditional returns-based performance
measure closely follows Eckbo and Smith (1998). We assume that the
conditional expected excess returns follow a ![]() -factor equilibrium model (see, for
example, Connor and Korajczyk (1995)),
-factor equilibrium model (see, for
example, Connor and Korajczyk (1995)),
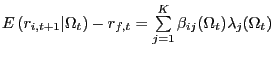 (C1)
(C1)
where
![]() denotes the mathematical expectation given
denotes the mathematical expectation given
![]() , the set of
all publicly available information at time
, the set of
all publicly available information at time ![]() ;
; ![]() is risk-free interest rate from holding period
is risk-free interest rate from holding period
![]() to
to ![]() ,which is known at time
,which is known at time
![]() ; and
; and
![]() and
and
![]() are, respectively, the systematic risk exposure of asset
are, respectively, the systematic risk exposure of asset
![]() to risk factor
to risk factor
![]() and the risk premium
of factor
and the risk premium
of factor ![]() , which are
both functions of
, which are
both functions of
![]() . We further
assume that the time variation of systematic risk exposure to the
factor (beta) and the factor risk premium follow linear functions
of a smaller set of public information variables,
. We further
assume that the time variation of systematic risk exposure to the
factor (beta) and the factor risk premium follow linear functions
of a smaller set of public information variables, ![]() , that is a subset of
, that is a subset of
![]() .
.
Following Ferson and Harvey (1993), Ferson and Korajczyk (1995),
and Eckbo and Smith (1998), equation (C1) can be estimated for U.S.
investors' portfolio, ![]() ,
with an intercept term,
,
with an intercept term,
![]() . The
performance measure,
. The
performance measure,
![]() , can be
estimated via GMM with the following moment conditions:
, can be
estimated via GMM with the following moment conditions:
![]() (C2)
(C2)
![]() (C3)
(C3)
![]() . (C4)
. (C4)
The parameters of the model are ![]() ,
, ![]() ,
and
,
and
![]() ,where
,where
![]() is vector of K factor
returns and
is vector of K factor
returns and ![]() is the
return of portfolio
is the
return of portfolio ![]() Equation (C2) is a K vector of errors from estimating a linear
function of factor risk premiums on information variables. Equation
(C3) is a K vector which can be viewed as errors from estimates of
conditional betas that are linear functions of information
variables
Equation (C2) is a K vector of errors from estimating a linear
function of factor risk premiums on information variables. Equation
(C3) is a K vector which can be viewed as errors from estimates of
conditional betas that are linear functions of information
variables
![]() ,
where
,
where
![]() . L is the number of information variables. Equation (C4) is
the error from estimating a conditional Jensen's alpha, an average
difference between the return from the portfolio and returns
implied from the K-factor model.
. L is the number of information variables. Equation (C4) is
the error from estimating a conditional Jensen's alpha, an average
difference between the return from the portfolio and returns
implied from the K-factor model.
We set up the following system of moment conditions
![\begin{displaymath}g_{t}=\left[ \begin{array}[c]{c} e_{\lambda}\cdot Z_{t}\ e_{\beta}\cdot Z_{t}\ e_{CRW} \end{array}\right] \end{displaymath}](img66.gif) (C5)
(C5)
and
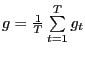 (C6)
(C6)
The sample moment conditions ![]() are a 2*K*L + 1 vector, and the GMM estimates are
obtained by minimizing the function
are a 2*K*L + 1 vector, and the GMM estimates are
obtained by minimizing the function
![]() , where W
is a positive-definite matrix (Hansen (1982)). We perform a
two-step iterative GMM estimation and use the Newey-West (1987)
covariance matrix for W.
, where W
is a positive-definite matrix (Hansen (1982)). We perform a
two-step iterative GMM estimation and use the Newey-West (1987)
covariance matrix for W.
In the conditional returns-based measure, a significantly positive intercept term (the conditional Jensen's alpha) would be evidence of superior performance that owed to private information about future returns beyond what can be exploited from public information.
Conditional portfolio weight-based measure
Our implementation of a conditional weight-based performance measure closely follows Eckbo and Smith (1998) and Ferson and Khang (2002), who extended the Grinblatt and Titman (1989, 1993) framework to allow for time-varying expected returns. Under time-varying expected returns, an investor would move into (out of) the market when private information indicates a positive (negative) abnormal return-above an expected return predicted from using public information. The estimate of the sum of the conditional covariances is defined as
![$ \sum\limits_{i=1}^{N_{t}}Cov\left( w_{i,t},r_{i,t+1}\vert\Omega_{t}\right) =\s... ...{i,t+1}-E\left( r_{i,t+1}\vert\Omega_{t}\right) \right) \vert\Omega_{t}\right] $](img70.gif) (C7)
(C7)
where
![]() is the
benchmark weight of country
is the
benchmark weight of country ![]() at time
at time ![]() .
The benchmark could be any portfolio weight which we want to
measure the performance against; in this paper we use a
buy-and-hold strategy. The buy-and-hold strategy weight of lag
.
The benchmark could be any portfolio weight which we want to
measure the performance against; in this paper we use a
buy-and-hold strategy. The buy-and-hold strategy weight of lag
![]() is defined as
is defined as
![]() (C8)
(C8)
This is a general form of a buy-and-hold strategy from the
second-term of equation (B1) in the case of ![]() = 1. We estimate the conditional
portfolio weight-based measure via GMM:
= 1. We estimate the conditional
portfolio weight-based measure via GMM:
![]() (C9)
(C9)
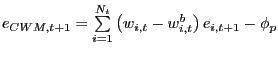 (C10)
(C10)
Equation (C9) is an ![]() vector of errors from estimating a linear function of future excess
returns on information variables when
vector of errors from estimating a linear function of future excess
returns on information variables when ![]() is the maximum value of
is the maximum value of ![]() for the full sample. The date at
which each country enters our U.S. portfolio evaluation is depicted
in Figure A1. Each error in equation (C9) has an interpretation of
an abnormal return. Equation (C10) is the error from estimating an
average of the conditional covariances between changes in portfolio
weights and future abnormal returns.
for the full sample. The date at
which each country enters our U.S. portfolio evaluation is depicted
in Figure A1. Each error in equation (C9) has an interpretation of
an abnormal return. Equation (C10) is the error from estimating an
average of the conditional covariances between changes in portfolio
weights and future abnormal returns. ![]() is the average of conditional weight measure
across the full sample. We set up the following system of moment
conditions
is the average of conditional weight measure
across the full sample. We set up the following system of moment
conditions
![\begin{displaymath}g_{t}=\left[ \begin{array}[c]{c} e_{i}\cdot Z\ e_{CWM}\cdot Z \end{array}\right] \end{displaymath}](img76.gif) (C11)
(C11)
The vector of sample moment conditions ![]() is a NL+L vector, and the parameters
are N vectors of L by 1 (
is a NL+L vector, and the parameters
are N vectors of L by 1 (![]() and a scalar
and a scalar ![]() .
.
The starting date in our large panel of international data
varies by country. MSCI total return data are available for the
full sample for developed markets and from the early 1990's for
emerging markets. We could estimate the model starting from the
date at which we have all country returns data. Instead, we exploit
all available information by using the whole sample and including
an indicator variable to control for missing values. Following
Bansal and Dahlquist (2000), we define ![]() , which indicates variable
denoting data availability for a country
, which indicates variable
denoting data availability for a country ![]() at time
at time ![]() , as
, as
![\begin{displaymath}I_{i,t}= \begin{array}[c]{c} \text{1, if data is observed at ... ... is not observed at }t\text{ for country }i\text{ } \end{array}\end{displaymath}](img79.gif) (C12)
(C12)
The key assumption is that ![]() is independent of the error terms from
equations (C9) and (C10), which implies that data are missing
randomly. This assumption would be violated if, for example,
missing data were all in periods with abnormally high excess
returns, which is not likely the case. The indicator variable will
in effect fill in missing values with zeros. We modify the error
term in equation (C9) by multiplying it with this indicator
variable, which in turn will affect equation (C10) through the
modified error term. Our augmented set of moments conditions
are
is independent of the error terms from
equations (C9) and (C10), which implies that data are missing
randomly. This assumption would be violated if, for example,
missing data were all in periods with abnormally high excess
returns, which is not likely the case. The indicator variable will
in effect fill in missing values with zeros. We modify the error
term in equation (C9) by multiplying it with this indicator
variable, which in turn will affect equation (C10) through the
modified error term. Our augmented set of moments conditions
are
![\begin{displaymath}g_{t}=\left[ \begin{array}[c]{c} e_{i}\cdot Z\cdot I_{i,t}\ e_{CWM}\cdot Z \end{array}\right] \end{displaymath}](img80.gif) (C13)
(C13)
Evidence of private information would be a positive estimate of the sum of the conditional covariances between changes in portfolio weight and future abnormal returns.
Notes for Figures
Figures 1a - 1g: Naive estimates are the thin lines; our benchmark-consistent estimates are the thick lines.
Figure 2: The share of foreign equities in world in U.S.
portfolios is derived from data taken from the S&P/IFC Emerging
Stock Markets Factbook (various issues) and the World Federation of
Exchanges (www.fibv.com). The share of
foreign equities in U.S. portfolios,
![]() , is
computed as U.S. holdings of foreign equities,
, is
computed as U.S. holdings of foreign equities,
![]() , divided by
U.S. holdings all equities. U.S. holdings of U.S. equities is
formed by subtracting the Rogers and Warnock (2004) estimate of
foreign holdings of U.S. equities,
, divided by
U.S. holdings all equities. U.S. holdings of U.S. equities is
formed by subtracting the Rogers and Warnock (2004) estimate of
foreign holdings of U.S. equities,
![]() , from U.S.
market capitalization. Specifically,
, from U.S.
market capitalization. Specifically,
![]()
Figure 3a: The asset-weighted dollar index is formed as
![]() ,
where
,
where ![]() is currency
is currency
![]() per dollar and
per dollar and
![]() is the time
is the time
![]() weight of country
weight of country
![]() 's equities in U.S.
investors' foreign equity portfolio. Cumulative returns
differential is a measure of the difference, since end-December
1976, between the total excess return on the total excess return on
U.S. residents' holdings of U.S. equities and U.S. residents'
holdings of foreign equities (expressed as a percent of the total
excess return to holding U.S. equities). Specifically, it is
computed as follows:
's equities in U.S.
investors' foreign equity portfolio. Cumulative returns
differential is a measure of the difference, since end-December
1976, between the total excess return on the total excess return on
U.S. residents' holdings of U.S. equities and U.S. residents'
holdings of foreign equities (expressed as a percent of the total
excess return to holding U.S. equities). Specifically, it is
computed as follows:
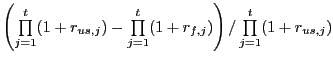
where ![]() =1
corresponds to January 1977;
=1
corresponds to January 1977; ![]() corresponds to the point plotted on the graph; and
corresponds to the point plotted on the graph; and
![]() and
and ![]() refer to U.S. and foreign
(dollar) returns, respectively.
refer to U.S. and foreign
(dollar) returns, respectively.
Figure 3b: The figure shows the Sharpe ratio for portfolios comprised of U.S. and foreign equities. The foreign component is formed by aggregating country returns using two different (time-varying) weighting schemes: a benchmark scheme that uses market weights (dotted line) and a scheme using the country weights in U.S. investors' foreign equity portfolios (solid line). The maximum point on each line, indicated by a triangle, represents the maximal Sharpe ratio attainable by mixing U.S. equities with the benchmark (market-cap-weighted) and U.S. investors' foreign portfolios.
Figure A1: The figure shows the availability (and our use) of data on equity returns. White space corresponds to periods for which we do not have returns data. Note that we use MSCI World for the Caribbean, which we exclude from our performance analysis. We also use the following indices for other areas excluded from our performance analysis: African Countries (MSCI EM Europe and Middle East), Other Latin America (MSCI Latin America Free index), and Other (MSCI EM Free).
Figure 1. U.S. Holdings of Foreign Equities
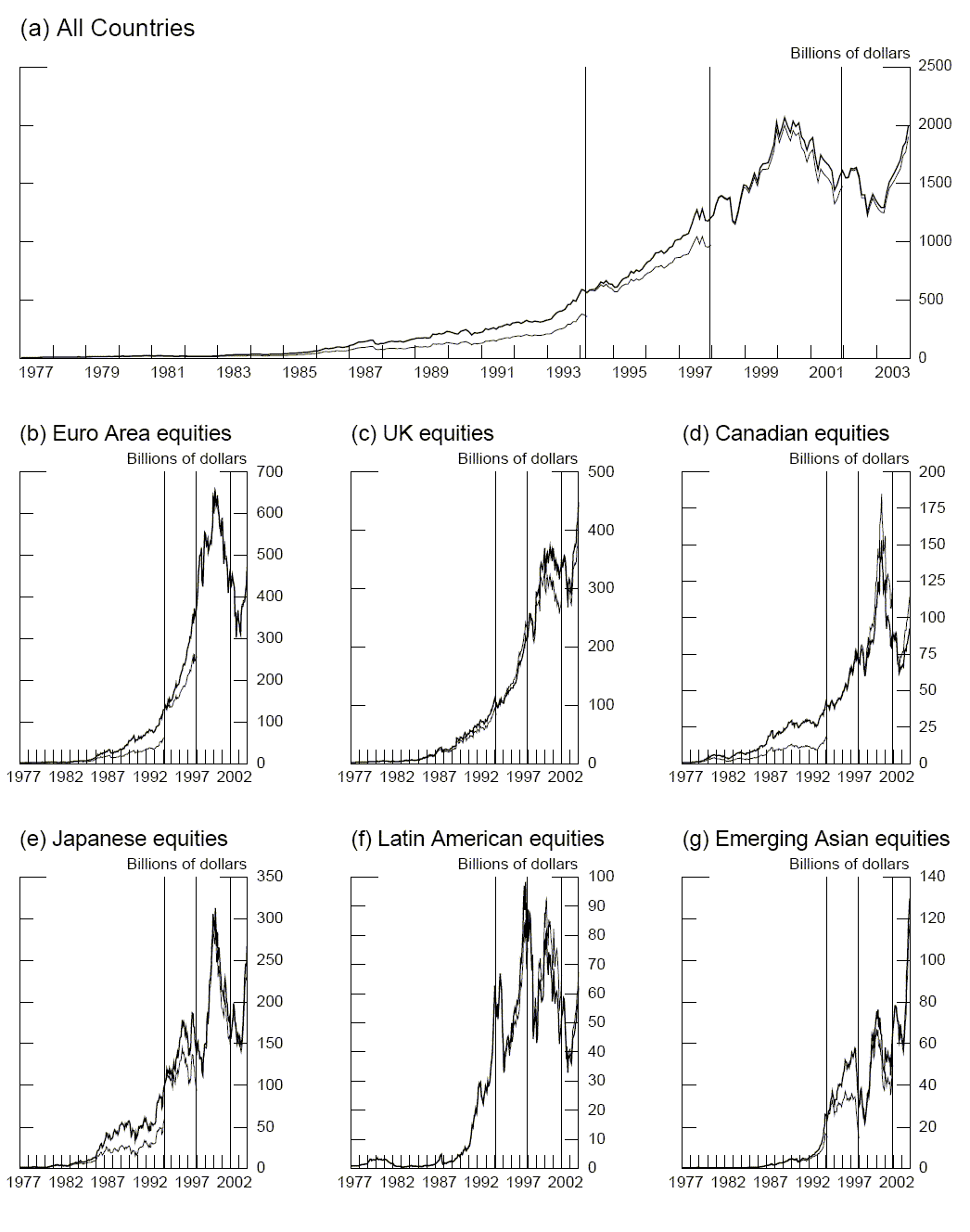
Figure 2. Share of Foreign Equities in World and U.S. Portfolios
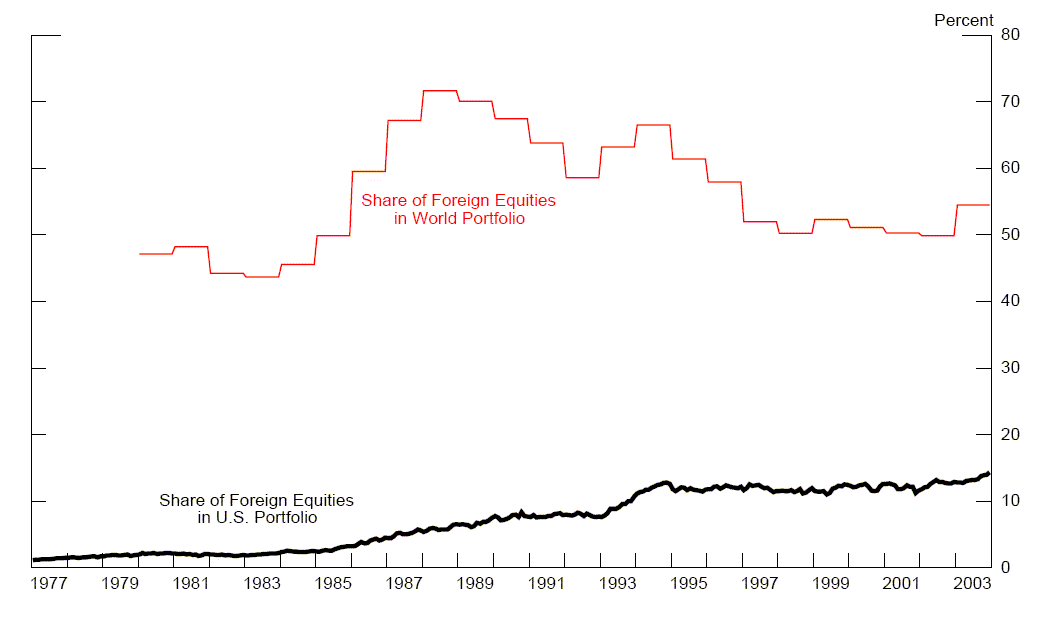
Figure 3a. Cumulative Return Differentials for Equities and Asset-Weighted Dollar Index
![Figure 3a plots the asset-weighted dollar index and the total return differential (U.S. - Foreign), both over 1977-2003. The dollar index ranges over [60,160]; the differential ranges over [-140%,+40%].
The two series move similarly over time. This figure shows that total U.S. investors' global portfolio depends largely on the movement of the dollar exchange rate.](Figure3a.gif)
Figure 3b. Reward-to-Risk Ratios, Equities (1977-2001)
Figure A1. Data Availability: Equity Returns
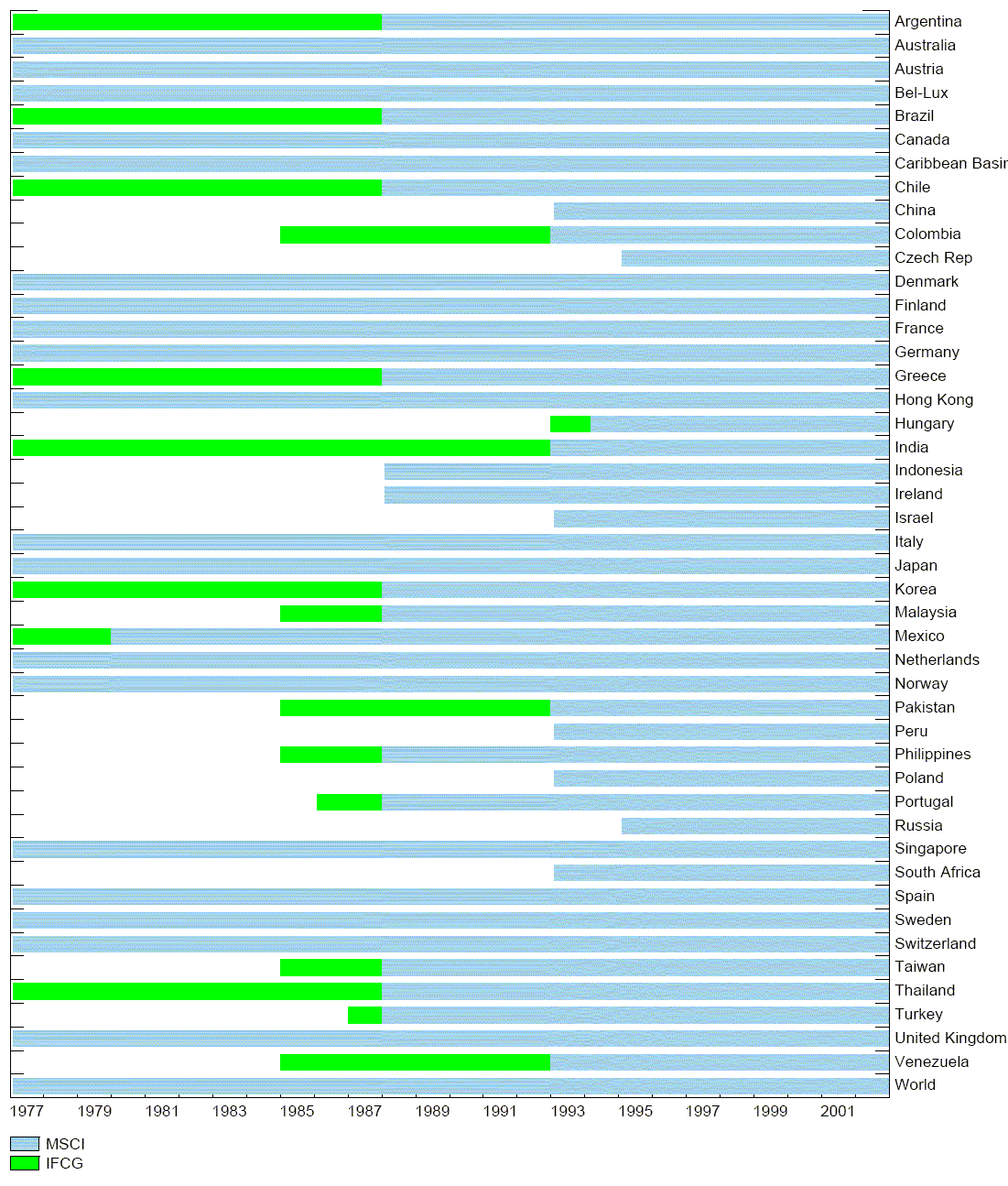
Table 1. Foreign Equity Weights in MSCI and U.S. Investors' Portfolios
This tables compares the evolution of country weights (expressed in percent) in U.S.investors' foreign with benchmark weights from the MSCI World ex US index. Data are as of year-end. Ellipses (�) indicate areas included in our holdings estimates that, owing to data limitations, are not in our performance analysis.
| 1989: MSCI | 1989: US | 1996: MSCI | 1996: US | 2001: MSCI | 2001: US | |
| Developed Countries | 92.7 | 92.9 | 82.0 | 82.0 | 88.2 | 86.0 |
| Euro Area | 17.0 | 24.4 | 20.2 | 26.3 | 31.7 | 32.4 |
| Austria | 0.3 | 0.3 | 0.4 | 0.3 | 0.1 | 0.1 |
| Belgium/Lux | 0.9 | 1.3 | 1.0 | 1.1 | 1.0 | 0.8 |
| Finland | 0.3 | 0.3 | 0.4 | 0.9 | 2.0 | 3.6 |
| France | 4.3 | 6.9 | 5.3 | 5.4 | 9.1 | 7.9 |
| Germany | 5.1 | 4.2 | 5.8 | 4.8 | 6.8 | 5.1 |
| Greece | 0.1 | 0.0 | 0.1 | 0.1 | 0.3 | 0.2 |
| Ireland | 0.2 | 0.3 | 0.2 | 0.8 | 0.7 | 2.0 |
| Italy | 2.1 | 1.6 | 1.9 | 2.4 | 3.5 | 2.4 |
| Netherlands | 2.1 | 7.3 | 3.4 | 8.0 | 5.0 | 7.9 |
| Portugal | 0.1 | 0.1 | 0.2 | 0.2 | 0.4 | 0.3 |
| Spain | 1.4 | 2.0 | 1.5 | 2.2 | 2.8 | 2.3 |
| Other Europe | 16.0 | 27.2 | 22.0 | 26.2 | 31.5 | 32.7 |
| Denmark | 0.5 | 0.4 | 0.7 | 0.6 | 0.7 | 0.5 |
| Norway | 0.3 | 0.7 | 0.4 | 0.8 | 0.4 | 0.6 |
| Sweden | 1.5 | 2.2 | 1.7 | 3.3 | 2.1 | 1.7 |
| Switzerland | 2.4 | 3.7 | 5.0 | 4.6 | 6.4 | 5.3 |
| Great Britain | 11.3 | 20.2 | 14.2 | 16.9 | 21.8 | 24.6 |
| Other Developed | 59.8 | 41.3 | 39.8 | 29.4 | 25.0 | 20.9 |
| Australia | 1.9 | 2.7 | 2.2 | 3.2 | 3.2 | 2.6 |
| Canada | 3.7 | 13.1 | 3.2 | 6.1 | 4.5 | 6.3 |
| Caribbean | ... | ... | ... | ... | ... | ... |
| Japan | 54.2 | 25.4 | 34.5 | 20.1 | 17.3 | 12.0 |
| Emerging Markets | 7.3 | 7.1 | 18.0 | 18.0 | 11.8 | 14.0 |
| Latin America | 0.8 | 2.1 | 3.4 | 5.8 | 2.2 | 3.6 |
| Argentina | 0.0 | 0.2 | 0.4 | 1.1 | 0.1 | 0.1 |
| Brazil | 0.4 | 0.3 | 1.2 | 1.4 | 0.9 | 1.5 |
| Chile | 0.1 | 0.2 | 0.6 | 0.6 | 0.2 | 0.1 |
| Colombia | 0.0 | 0.0 | 0.1 | 0.0 | 0.0 | 0.0 |
| Mexico | 0.3 | 1.4 | 0.9 | 2.5 | 0.9 | 1.8 |
| Peru | 0.0 | 0.0 | 0.1 | 0.1 | 0.0 | 0.0 |
| Venezuela | 0.0 | 0.0 | 0.0 | 0.1 | 0.0 | 0.0 |
| Other LA | ... | ... | ... | ... | ... | ... |
| Emerging Asia | 6.3 | 3.6 | 12.0 | 10.2 | 7.5 | 8.2 |
| China | 0.0 | 0.2 | 0.0 | 0.2 | 0.6 | 0.2 |
| Hong Kong | 0.9 | 0.9 | 2.6 | 3.2 | 1.7 | 2.1 |
| India | 0.0 | 0.0 | 0.6 | 0.3 | 0.5 | 0.5 |
| Indonesia | 0.0 | 0.1 | 0.6 | 0.6 | 0.1 | 0.1 |
| Korea | 1.4 | 0.5 | 1.8 | 1.4 | 1.6 | 2.1 |
| Malaysia | 0.5 | 0.3 | 1.8 | 1.4 | 0.6 | 0.2 |
| Pakistan | 0.0 | 0.0 | 0.1 | 0.0 | 0.0 | 0.0 |
| Phillippines | 0.1 | 0.3 | 0.3 | 0.5 | 0.1 | 0.1 |
| Singapore | 0.6 | 0.6 | 1.3 | 1.4 | 0.8 | 1.5 |
| Taiwan | 2.5 | 0.1 | 1.7 | 0.2 | 1.4 | 1.4 |
| Thailand | 0.3 | 0.5 | 1.1 | 1.0 | 0.1 | 0.1 |
| Emerging Europe | 0.1 | 0.1 | 0.5 | 0.3 | 0.7 | 0.7 |
| Czech | 0.0 | 0.0 | 0.2 | 0.0 | 0.1 | 0.0 |
| Hungary | 0.0 | 0.0 | 0.0 | 0.0 | 0.1 | 0.1 |
| Poland | 0.0 | 0.0 | 0.0 | 0.0 | 0.1 | 0.1 |
| Russia | 0.0 | 0.0 | 0.1 | 0.0 | 0.3 | 0.3 |
| Turkey | 0.1 | 0.1 | 0.1 | 0.2 | 0.2 | 0.2 |
| Other Emerging | 0.0 | 1.3 | 2.1 | 1.7 | 1.3 | 1.4 |
| Israel | 0.0 | 0.4 | 0.3 | 0.5 | 0.4 | 0.9 |
| South Africa | 0.0 | 0.9 | 1.8 | 1.2 | 0.9 | 0.5 |
| Other Africa | ... | ... | ... | ... | ... | ... |
| Other | ... | ... | ... | ... | ... | ... |
| Memo: U.S. Investors' Foreign Equity Portfolio ($ billions) | 235 | 804 | 1,613 |
Table 2. The Performance of U.S. Investors' Foreign Equity Portfolios
This table reports means, standard deviations, and Sharpe ratios
(mean divided by standard deviation) for portfolios of foreign
equities. Returns are in excess of a one-month Eurodollar interest
rate and are expressed in monthly percentage points. Value-weighted benchmarks are portfolios based on
MSCI market capitalization weights. U.S.
investors' portfolios are based on U.S. investors' holdings.
The Chi-squared: Sharpe Ratio is a test
statistic for the null hypothesis that Sharpe ratios in the two
columns are equal. Panels A-C report statistics for the following
samples: the full sample (January 1977 through December 2001), two
subsample periods (January 1977 through December 1989 and January
1990 through December 2001), and two groups of countries (Developed
and Emerging markets). Asymptotic ![]() -values computed from Newey and West (1987) standard
errors are in brackets.* Statistically
significant at the 5 percent level.
-values computed from Newey and West (1987) standard
errors are in brackets.* Statistically
significant at the 5 percent level.
Panel A: Full Sample (1977 - 2001)
| 1977 - 2001 | Value-Weighted Benchmark | U.S. Investors' Foreign Portfolio |
| Mean | 0.437 | 0.482 |
| Std Dev | 4.803 | 4.275 |
| Sharpe Ratio (%) | 9.093 | 11.273 |
| Chi-squared: Sharpe Ratio | 1.386
[0.239] |
Panel B: Pre- and post-1990
| 1977 - 1989 | Value-Weighted Benchmark | U.S. Investors' Foreign Portfolio |
| Mean | 0.922 | 0.807 |
| Std Dev | 4.720 | 4.117 |
| Sharpe Ratio (%) | 19.532 | 19.608 |
| Chi-squared: Sharpe Ratio | 0.001
[0.976] |
|
| 1990 - 2001 | Value-Weighted Benchmark | U.S. Investors' Foreign Portfolio |
|---|---|---|
| Mean | -0.108 | 0.132 |
| Std Dev | 4.815 | 4.412 |
| Sharpe Ratio (%) | -2.241 | 2.983 |
| Chi-squared: Sharpe Ratio | 4.952*
[0.026] |
|
Panel C: Developed and Emerging Equity Markets, 1990 - 2001
| Developed Markets | Value-Weighted Benchmark | U.S. Investors' Foreign Portfolio |
| Mean | -0.077 | 0.137 |
| Std Dev | 4.846 | 4.347 |
| Sharpe Ratio (%) | -1.589 | 3.154 |
| Chi-squared: Sharpe Ratio | 4.572*
[0.033] |
|
| Emerging Markets | Value-Weighted Benchmark | U.S. Investors' Foreign Portfolio |
| Mean | -0.120 | 0.473 |
| Std Dev | 6.786 | 6.940 |
| Sharpe Ratio (%) | -1.761 | 6.808 |
| Chi-squared: Sharpe Ratio | 4.094*
[0.043] |
Table 3. Momentum Measures for the Foreign Equity Portfolio
The LM statistic is a measure of momentum based on deviations of portfolio weights from a passive buy-and-hold strategy (equation (B1)). The BM statistic is a measure of momentum based on the positive portfolio weight deviations from a passive buy-and-hold strategy (equation (B3)). The SM statistic is a measure of momentum based on the negative portfolio weight deviations from a passive buy-and-hold strategy (equation (B4)). Lag 1, Lag 2, and Lag 3 correspond to the measure of momentum based on returns lagged 1, 2, and 3 months, respectively. Panel A reports statistics for all countries for the full sample (January 1977 through December 2001) and two subsample periods (January 1977 through December 1989 and January 1990 through December 2001). Panel B reports estimates for two groups of countries (Developed and Emerging Markets) for 1990 - 2001. Newey and West (1987) standard errors are in parentheses. * Statistically significant at the 5 percent level.
Panel A: All Countries
| Momentum Measure | 1977-2001: Lag 1 | 1977-2001: Lag 2 | 1977-2001: Lag 3 | 1977-1989: Lag 1 | 1977-1989: Lag 2 | 1977-1989: Lag 3 | 1990-2001: Lag 1 | 1990-2001: Lag 2 | 1990-2001: Lag 3 |
| LM (Buy and Sell) | 0.074
(0.152) |
-0.353*
(0.158) |
-0.218
(0.169) |
0.105
(0.209) |
-0.496*
(0.220) |
-0.046
(0.245) |
0.047
(0.225) |
-0.259
(0.222) |
-0.479*
(0.230) |
| BM (Buy Only) | 0.244*
(0.116) |
0.080
(0.114) |
0.089
(0.107) |
0.269
(0.144) |
0.051
(0.139) |
0.201
(0.156) |
0.214
(0.189) |
0.058
(0.175) |
-0.075
(0.145) |
| SM (Sell Only) | -0.170*
(0.073) |
-0.434*
(0.081) |
-0.307*
(0.086) |
-0.164
(0.112) |
-0.547*
(0.132) |
-0.248
(0.133) |
-0.167*
(0.084) |
-0.317*
(0.091) |
-0.404*
(0.109) |
Panel B: Country Splits, 1990 - 2001
| Momentum Measure | Developed Countries: Lag 1 | Developed Countries: Lag 2 | Developed Countries: Lag 3 | Emerging Markets: Lag 1 | Emerging Markets: Lag 2 | Emerging Markets: Lag 3 |
| LM (Buy and Sell) | -0.095
(0.225) |
-0.391
(0.211) |
-0.545*
(0.228) |
0.640
(0.467) |
-0.255
(0.590) |
-0.505
(0.519) |
| BM (Buy Only) | 0.026
(0.185) |
-0.154
(0.152) |
-0.263
(0.150) |
0.964*
(0.333) |
0.584
(0.446) |
0.269
(0.349) |
| SM (Sell Only) | -0.121*
(0.089) |
-0.237*
(0.083) |
-0.283*
(0.104) |
-0.324
(0.213) |
-0.838*
(0.263) |
-0.774*
(0.256) |
Table 4. Conditional Jensen's Alpha for U.S. Investors' Foreign Equity Portfolio
This table reports GMM estimates of conditional Jensen's alpha,
![]() , using
the following system of equations:
, using
the following system of equations:
![]()
![]()
![]() .
.
.
where ![]() is
the excess return in month
is
the excess return in month ![]() of U.S. investors' foreign equity portfolio,
of U.S. investors' foreign equity portfolio,
![]() is the set of
information variables (including a constant), and
is the set of
information variables (including a constant), and ![]() is the set of risk factors.
Three different factor pricing models are used. CAPM represents a one-factor model that includes
the excess return on the world market portfolio. CAPM and HML represents a two-factor model that
includes the excess return on the world market portfolio and the
difference between returns on global portfolio of high
book-to-market and low book-to-market (HML). CAPM and FX represents a four-factor model that
includes the excess return on the world market portfolio and
foreign exchange (FX) risks proxied by excess returns from
investing in euro, yen, and sterling interest rates. Chi-sq: Constant
is the set of risk factors.
Three different factor pricing models are used. CAPM represents a one-factor model that includes
the excess return on the world market portfolio. CAPM and HML represents a two-factor model that
includes the excess return on the world market portfolio and the
difference between returns on global portfolio of high
book-to-market and low book-to-market (HML). CAPM and FX represents a four-factor model that
includes the excess return on the world market portfolio and
foreign exchange (FX) risks proxied by excess returns from
investing in euro, yen, and sterling interest rates. Chi-sq: Constant
![]() is a
test statistic for the null hypothesis that estimates in vector
is a
test statistic for the null hypothesis that estimates in vector
![]() in equation (C3), except the
intercept, are jointly insignificant. Chi-sq:
Constant Risk Premium is a test statistic for the null
hypothesis that estimates in vector
in equation (C3), except the
intercept, are jointly insignificant. Chi-sq:
Constant Risk Premium is a test statistic for the null
hypothesis that estimates in vector
![]() in
equation (C2), except the intercept, are jointly insignificant. In
Panel A, estimates are from the full sample. Panel B shows
estimates for two subsamples: January 1977 through December 1989
and January 1990 through December 2001. Test
Equal
in
equation (C2), except the intercept, are jointly insignificant. In
Panel A, estimates are from the full sample. Panel B shows
estimates for two subsamples: January 1977 through December 1989
and January 1990 through December 2001. Test
Equal
![]() is a
Chi-squared test statistic for the null of hypothesis that alpha is
equal for the two subsample periods. Panel C reports estimates from
the sample from January 1990 through December 2001 for two group of
countries: Developed and Emerging markets. Newey and West (1987)
standard errors are in parentheses. Asymptotic
is a
Chi-squared test statistic for the null of hypothesis that alpha is
equal for the two subsample periods. Panel C reports estimates from
the sample from January 1990 through December 2001 for two group of
countries: Developed and Emerging markets. Newey and West (1987)
standard errors are in parentheses. Asymptotic ![]() -values computing from Newey and West
(1987) standard errors are in brackets. * Statistically significant at the 5 percent
level.
-values computing from Newey and West
(1987) standard errors are in brackets. * Statistically significant at the 5 percent
level.
Panel A: Full Sample (1977 - 2001)
| Time-Varying Beta: CAPM | Time-Varying Beta: CAPM and HML | Time-Varying Beta: CAPM and FX | Constant Beta: CAPM | Constant Beta: CAPM and HML | Constant Beta: CAPM and FX | |
|
|
0.105
(0.106) |
0.072
(0.104) |
0.095
(0.114) |
0.042
(0.084) |
0.024
(0.086) |
0.037
(0.079) |
| Chi-sq: Constant
|
2.942
[0.401] |
4.925
[0.553] |
18.442
[0.103] |
|||
| Chi-sq: Constant Risk Premium | 21.469*
[0.000] |
18.783*
[0.005] |
56.385*
[0.000] |
19.245*
[0.000] |
20.723*
[0.002] |
50.459*
[0.000] |
Panel B: Pre- and post-1990
| Time-Varying Beta: CAPM | Time-Varying Beta: CAPM and HML | Time-Varying Beta: CAPM and FX | Constant Beta: CAPM | Constant Beta: CAPM and HML | Constant Beta: CAPM and FX | |
|
|
0.537
(0.341) |
0.472
(0.242) |
0.456
(0.316) |
0.407
(0.237) |
0.387
(0.236) |
0.404
(0.230) |
|
|
-0.369
(0.267) |
-0.314
(0.269) |
-0.315
(0.283) |
-0.349
(0.262) |
-0.368
(0.263) |
-0.360
(0.269) |
| Chi-sq: Constant
|
2.842
[0.417] |
7.671
[0.263] |
15.957
[0.193] |
|||
| Chi-sq: Constant Risk Premium | 21.616*
[0.000] |
25.443*
[0.000] |
48.796*
[0.000] |
19.225*
[0.000] |
20.699*
[0.002] |
50.459*
[0.000] |
| Test Equal
|
3.248
[0.072] |
2.933
[0.087] |
2.856
[0.091] |
2.618
[0.106] |
2.619
[0.106] |
2.619
[0.106] |
Panel C: Post-1990 (1990 - 2001)
| Developed Markets | Time-Varying Beta: CAPM | Time-Varying Beta: CAPM and HML | Time-Varying Beta: CAPM and FX | Constant Beta: CAPM | Constant Beta: CAPM and HML | Constant Beta: CAPM and FX |
|
|
-0.084
(0.195) |
-0.115
(0.218) |
0.376
(0.862) |
-0.077
(0.196) |
-0.089
(0.200) |
-0.075
(0.182) |
| Chi-sq: Constant
|
0.129
[0.988] |
0.781
[0.993] |
3.351
[0.993] |
|||
| Chi-sq: Constant Risk Premium | 0.228
[0.973] |
1.061
[0.983] |
12.970
[0.371] |
0.395
[0.941] |
0.688
[0.995] |
5.697
[0.931] |
| Emerging Markets | Time-Varying Beta: CAPM | Time-Varying Beta: CAPM and HML | Time-Varying Beta: CAPM and FX | Constant Beta: CAPM | Constant Beta: CAPM and HML | Constant Beta: CAPM and FX |
|
|
0.933
(1.430) |
-0.011
(1.329) |
0.088
(1.363) |
0.219
(1.135) |
0.221
(1.153) |
0.220
(1.072) |
| Chi-sq: Constant
|
2.311
[0.510] |
3.430
[0.753] |
4.509
[0.972] |
|||
| Chi-sq: Constant Risk Premium | 5.855
[0.119] |
6.085
[0.414] |
9.440
[0.665] |
0.357
[0.949] |
0.711
[0.994] |
5.689
[0.931] |
Table 5. Conditional Portfolio Weight Performance Measure for U.S. Investors' Foreign Equity Portfolio
This table reports GMM estimates of
![]() for the following system:
for the following system:
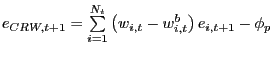
where ![]() is
the vector of portfolio excess returns in month
is
the vector of portfolio excess returns in month ![]() , b
, b![]() is the matrix of coefficients from regressing
is the matrix of coefficients from regressing
![]() on the
instruments,
on the
instruments, ![]() (including
a constant), and the parameter
(including
a constant), and the parameter
![]() is the
average conditional covariance. In Panel A, estimates are from the
full sample. Panel B shows estimates for two subsamples: January
1977 through December 1989 and January 1990 through December 2001.
Test Equal
is the
average conditional covariance. In Panel A, estimates are from the
full sample. Panel B shows estimates for two subsamples: January
1977 through December 1989 and January 1990 through December 2001.
Test Equal
![]() is a
Chi-squared test statistic for the null of hypothesis that
is a
Chi-squared test statistic for the null of hypothesis that
![]() is
equal in the two subsample periods. Panel C reports estimates from
the sample from January 1990 through December 2001 for two group of
countries: Developed and Emerging. Asymptotic
is
equal in the two subsample periods. Panel C reports estimates from
the sample from January 1990 through December 2001 for two group of
countries: Developed and Emerging. Asymptotic ![]() -values computed from Newey and West
(1987) standard errors are in parentheses. * Statistically significant at the 5 percent
level.
-values computed from Newey and West
(1987) standard errors are in parentheses. * Statistically significant at the 5 percent
level.
Panel A: Full Sample (1976 - 2001)
| k=1 | k=2 | k=3 | |
|
|
0.229
(0.167) |
0.246
(0.273) |
0.009
(0.370) |
Panel B: Pre- and post-1990
|
|
0.128
(0.277) |
0.163
(0.449) |
-0.048
(0.629) |
|
|
0.343
(0.216) |
0.352
(0.299) |
0.114
(0.361) |
| Test Equal
|
0.425
(0.515) |
0.126
(0.722) |
0.051
(0.822) |
Panel C: Post-1990 (1990 - 2001)
|
Developed Markets |
0.302
(0.268) |
0.452
(0.551) |
0.388
(0.562) |
|
Emerging Markets |
1.245
(0.898) |
1.253
(1.405) |
1.558
(2.117) |
Table 6. Governance, Cross-listings, and U.S. Portfolio Performance
This table reports means, standard deviations, and Sharpe ratios
(mean divided by standard deviation) for the period January 1990
through December 2001. Returns are in excess of a one-month
Eurodollar interest rate and are expressed in monthly percentage
points. U.S. investors' foreign equity portfolios are based on U.S.
investors' holdings. Weights in the value-weighted foreign
benchmark portfolios are based on MSCI market capitalizations
(column b); the dollar value of each country's market
capitalization that was dispersely held as of end-1997 (column c);
or the dollar value of each country's market capitalization that
was cross-listed on a U.S. exchange as of end-1997. The
Chi-squared: Sharpe Ratio is a test
statistic for the null hypothesis that Sharpe ratio in that column
is equal to the Sharpe ratio in column (a). Asymptotic ![]() -values computed from Newey and West
(1987) standard errors are in brackets. * Statistically significant at the 5 percent
level.
-values computed from Newey and West
(1987) standard errors are in brackets. * Statistically significant at the 5 percent
level.
| 1990 - 2001 | U.S. Investors' Foreign Equity Portfolio: (a) | Value-Weighted Foreign Benchmarks: MSCI (b) | Value-Weighted Foreign Benchmarks: Governance (c) | Value-Weighted Foreign Benchmarks: Cross-listed (d) |
| Mean | 0.132 | -0.108 | 0.236 | 0.281 |
| Std Dev | 4.412 | 4.815 | 4.342 | 4.326 |
| Sharpe Ratio (%) | 2.983 | -2.241 | 5.439 | 6.501 |
| Chi-squared: Sharpe Ratio | 4.952*
[0.026] |
3.363
[0.067] |
7.127*
[0.001] |
Table 7. The Performance of U.S. Investors' Global Equity Portfolios
This table reports means, standard deviations, and Sharpe ratios
(mean divided by standard deviation). Returns are in excess of a
one-month Eurodollar interest rate and are expressed in monthly
percentage points. Value-weighted benchmarks are portfolios based
on MSCI market capitalization weights. U.S. investors' portfolios
are based on U.S. investors' holdings. Global includes U.S. and foreign markets;
US is only the U.S. market; and
Foreign consists of non-U.S. markets.
Prior to 1990, MSCI value weights exclude emerging markets. The
Chi-squared: Sharpe Ratio is a test
statistic for the null hypothesis that Sharpe ratios in columns (c)
and (d) are equal. Panels A and B report statistics for equities
for the full sample (January 1977 through December 2001) and two
subsample periods (January 1977 through December 1989 and January
1990 through December 2001). Asymptotic ![]() -values computed from Newey and West
(1987) standard errors are in brackets. * Statistically significant at the 5 percent
level.
-values computed from Newey and West
(1987) standard errors are in brackets. * Statistically significant at the 5 percent
level.
Panel A: Full Sample (1977 - 2001)
| 1977 - 2001 | Value-Weighted
Benchmarks: US (a) |
Value-Weighted
Benchmarks: Foreign (b) |
Value-Weighted
Benchmarks: Global (c) |
U.S. Investors' Global Portfolio: (d) |
| Mean | 0.543 | 0.437 | 0.447 | 0.519 |
| Std Dev | 4.361 | 4.803 | 4.108 | 4.288 |
| Sharpe Ratio (%) | 12.450 | 9.093 | 10.885 | 12.099 |
| Chi-squared: Sharpe Ratio | 0.151
[0.698] |
Panel B: Pre- and post-1990
| 1977 - 1989 | Value-Weighted
Benchmarks: US (a) |
Value-Weighted
Benchmarks: Foreign (b) |
Value-Weighted
Benchmarks: Global (c) |
U.S. Investors' Global Portfolio: (d) |
| Mean | 0.413 | 0.922 | 0.662 | 0.423 |
| Std Dev | 4.510 | 4.720 | 4.001 | 4.450 |
| Sharpe Ratio (%) | 9.161 | 19.532 | 16.540 | 9.507 |
| Chi-squared: Sharpe Ratio | 2.345
[0.126] |
|||
| 1990 - 2001 | Value-Weighted
Benchmarks: US (a) |
Value-Weighted
Benchmarks: Foreign (b) |
Value-Weighted
Benchmarks: Global (c) |
U.S. Investors' Global Portfolio: (d) |
|---|---|---|---|---|
| Mean | 0.683 | -0.108 | 0.187 | 0.622 |
| Std Dev | 4.191 | 4.815 | 4.231 | 4.103 |
| Sharpe Ratio (%) | 16.291 | -2.241 | 4.425 | 15.152 |
| Chi-squared: Sharpe Ratio | 7.311*
[0.007] |
Footnotes
1. For example, Griever, Lee, and Warnock (2001, Table 3) show that U.S. investors' annual trading in foreign equities increased over forty-fold from the 1980s to 2000. Return to text
2. Unpublished international performance studies include Bange, Khang, and Miller (2003), which finds little evidence of conditional skill in investment houses' portfolio recommendations across 6 markets, and Glassman and Riddick (2003), which examines fund performance in 4 markets in the late 1980s. The related (and larger) literature on the relationship between international flows and returns-- for recent examples, see Froot, O'Connell, and Seasholes (2001), Griffin, Nadari, and Stulz (2004), and references therein--does not speak to portfolio performance. For example, if flows into all markets are positive and identical and all markets subsequently have positive returns, one would find a positive relationship between flows and returns in each market. This would not necessarily be superior performance if returns in some markets are stronger. Return to text
3. In an international context, evidence that firms with good governance have higher value is surveyed in Claessens and Fan (2002) and Denis and McConnell (2003). Doidge, Karolyi, and Stulz (2004) show that cross-listed firms have higher value. Return to text
4. The assumption that U.S. investors hold the ``market'' within foreign countries is borne out of necessity but reasonable. Using the U.S. benchmark survey from March 1994 and December 1997, Edison and Warnock (2004) and Ammer et al. (2004) find that U.S. investors tend to hold foreign equities that are large and liquid, the same types of stocks that are included in the MSCI index. The assumption implies that we evaluate country-picking ability, not stock selection within markets. Return to text
5. Our focus is on unhedged returns on foreign portfolios. These returns are comprised of two components, equity returns and changes in currency cross-rates. Thus, strictly speaking our study concerns the ability to pick equity markets and foreign currencies. However, to the extent that one-month currency movements are largely unforecastable--Meese and Rogoff (1983) and more recently Faust, Rogers, and Wright (2003) and Cheung, Chinn, and Pascual (2004)--this in effect reduces to the selection of equity markets. Return to text
6. Our holdings data will be posted at www.federalreserve.gov/pubs/ifdp/2004/817/default.htm. Return to text
7. The benchmark survey data collection procedure-the large custodians who report the majority of the data do not always distinguish between types of U.S. investors-makes it impossible to identify the ultimate U.S. investor with precision. That said, the typical U.S. investor who invests in foreign securities is likely an institution. In the 1997 survey, the type of U.S. investor was denoted for $667 billion of the reported $1208 billion in U.S. holdings of foreign equities. Of the $667 billion in holdings, 93 percent was held by mutual funds or pension funds. Return to text
8. We do not show performance against equally weighted portfolios because this allocation is not feasible. In December 1997, U.S. investors' foreign equity position was about $1.15 trillion, so equal allocations across 44 countries would imply an investment of $26 billion in each country, or more than 100 percent of the (MSCI) market capitalization of 16 countries in our sample. Return to text
9. A momentum investor buys past winners and sells past losers; a contrarian investor does the opposite. Return to text
10. At first glance, our contrarian when selling results appear to contrast with Kaminsky, Lyons, and Schmukler (2004), who find that 13 Latin American mutual funds exhibit momentum trading over the period from 1993 - 1999. However, most of their evidence pertains to LM (Buy and Sell) at a zero lag; we do not analyze contemporaneous momentum statistics because it is impossible to disentangle truly momentum trading (flows following price) from price pressure (price reacting to flows). Moreover, they do not compute BM and SM statistics, so our studies are not directly comparable. Return to text
11. To capture the predictability of future returns, we use information variables that have been found to have robust predictive power for aggregate country-level expected returns (Harvey, 1991; Ferson and Harvey, 1993; and Bekaert and Harvey, 1997). These information variables include lagged changes in the short-term interest rate (U.S. Treasury three-month yield), lagged changes in term structure spread (U.S. Treasury 10-year yield minus U.S. Treasury 3-month yield), and lagged world excess returns. Consistent with the findings in Ang and Bekaert (2002) and Campbell and Yogo (2003), our (untabulated) country-by-country regressions indicate that the interest rate variables have the most power for predicting future returns. We also experimented with a lagged default spread (Moody's Baa minus Aaa bond yields) and lagged local excess returns, but found that these variables have little predictive power in most countries; including these two variables do not change our results. We do not use the local or global dividend yield. Ferson, Sarkisssian, and Simin (2003) illustrate that returns prediction regressions with persistent variables such as the dividend yield tend to over-reject the null hypothesis of no predictability. Moreover, Campbell and Yogo (2003), who account for this bias in a study of the U.S. market, and Ang and Bekaert (2002) and Bekaert, Harvey, and Lundblad (2003), who use Monte Carlo simulations for a range of emerging and developed markets, find no predictive power for the dividend yield. Return to text
12. In our asset pricing models, aggregate country-level equity indices of total (price and dividend) returns and market capitalization in U.S. dollars are from MSCI. The starting date for each country is shown in Figure A1. The MSCI World index consists of 23 developed markets with the sample starting from December 1969. Total returns indices for most developed markets are available from the beginning of our sample, January 1977, while most emerging markets indices are available from the early 1990s. All excess returns are computed over the one-month Eurodollar interest rate. Results using the MSCI All Country World index (not reported), which consists of 49 developed and emerging markets and starts in December 1987 are qualitatively similar; the sample correlation of returns computed from MSCI World and MSCI All Country World indices is 0.998. Return to text
13. Our two-factor model utilizes MSCI World growth and value indices, which include 29 developed markets and are available from January 1975. MSCI All Country World growth and values indices include both developed and emerging markets and are available from December 1987. Results based on the All Country indices (not reported) are very similar to the ones using only developed markets; the sample correlation of the difference between global portfolio returns of value and growth stocks based on the MSCI World and All Country World indices is 0.999. Return to text
14. Table 3 of Thomas et al. (2004) presents results, including conditional Jensen's alpha, of the conditional pricing models for each country in our sample. There is little if any evidence that our conditional asset pricing models are not valid for this set of countries. Return to text
15. We estimate the model jointly but allow an estimate of Jensen's alpha for each subsample. Return to text
16. This approach also mitigates interim trading bias, which refers to a bias when trading is carried out at a higher frequency than holdings observations. Return to text
17. See Claessens and Fan (2002) and Denis and McConnell (2003) for recent surveys on the international corporate governance literature, and Doidge, Karolyi, and Stulz (2004) on the value impact of cross-listing. Return to text
18. When we also constrain U.S. and MSCI portfolios to have fixed end-1997 weights (not shown), the same conclusion is reached. Return to text
19. Relative returns differentials in Figure 3a are a measure of the difference, since end-December 1976, between the total excess return on U.S. residents' holdings of U.S. equities and the total excess return on U.S. residents' holdings of foreign equities (expressed as a percent of the total excess return to holding U.S. equities). Specifically, it is computed as follows:
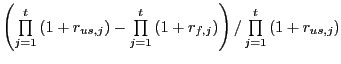
where ![]() =1
corresponds to January 1977;
=1
corresponds to January 1977; ![]() corresponds to the point plotted on the graph; and
corresponds to the point plotted on the graph; and
![]() and
and ![]() refer to U.S. and foreign
(dollar) returns, respectively. Return
to text
refer to U.S. and foreign
(dollar) returns, respectively. Return
to text
20. The index is formed as
![]() ,
,
where ![]() is
currency
is
currency ![]() per dollar
and
per dollar
and ![]() is the time
is the time
![]() weight of country
weight of country
![]() 's equities in U.S.
investors' foreign equity portfolio. Further details and dollar
indices formed using other weighting schemes are in Rogers and
Warnock (2004). Return to text
's equities in U.S.
investors' foreign equity portfolio. Further details and dollar
indices formed using other weighting schemes are in Rogers and
Warnock (2004). Return to text
21. For models with barriers to international investment, see Black (1974), Stulz (1981), Merton (1987), and Gehrig (1993). Return to text
22. Details of the 2001 asset survey, including findings and methodology, are discussed in Treasury Department et al. (2003). Griever, Lee, and Warnock (2001) is a primer on the surveys. Asset surveys are now more frequent; starting in December 2003 annual ``mini'' surveys supplement the quinquennial full benchmarks. Return to text
23. ISIN (International Security Identification Number) and SEDOL (Stock Exchange Daily Official List) are the two primary security identification systems for non-U.S. securities. Return to text
24. S& P/IFC Global or Investable indices are both reasonable choices for equity returns, but these are less readily available for the current period so we use the more accessible MSCI indices. Return to text
25. See www.elkins-mcsherry.com, Willoughby (1998), and Domowitz, Glen, and Madhaven (2001) for discussions of the Elkins-McSherry data. Lesmond (2002) studies transaction costs in emerging equity markets, but to have one source for both emerging markets and devoloped countries, we use Elkins-McSherry data. Return to text
26. In their presentation of U.S. capital flows data, the Bureau of Economic Analysis (BEA) includes estimates of stock swaps. Aggregate stock swaps data are now posted on the TIC web site. Our data on bilateral stock swaps are from Security Data Corporation. Return to text
27. Our results are robust to different starting values, such as zero or 1994 positions scaled by the distribution of 1977 trading. We cannot use BEA data on bilateral positions because it is limited to selected countries for a limited number of years. Annual BEA estimates of U.S. positions in foreign securities, without country detail, is provided in Table 2 of Nguyen (2002). In general, our aggregate estimates are similar in spirit to BEA's but will differ in all cases except when a benchmark survey was conducted at the end of a year (1997 and 2001). Return to text
28. Another way to form estimates is to assume that there are errors in valuation adjustments. For example, investors might beat the market by x percentage points per month or earn returns that are more volatile than the market. Return to text
29. This adjustment is similar to
that in the security-level analysis of Grinblatt et al. (1995) and
Badrinath and Wahal (2002), who subtract security ![]() 's return from an expected return for
security
's return from an expected return for
security ![]() which is
proxied by a 12-month ahead return. We also compute buy and sell
momentum measures by replacing the return on the total foreign
portfolio with a 12-month ahead country return. The results (not
shown) are qualitatively similar. Return
to text
which is
proxied by a 12-month ahead return. We also compute buy and sell
momentum measures by replacing the return on the total foreign
portfolio with a 12-month ahead country return. The results (not
shown) are qualitatively similar. Return
to text
This version is optimized for use by screen readers. A printable pdf version is available.