The Federal Reserve Board eagle logo links to home page

Cash Flows and Discount Rates, Industry and Country Effects, and Co-Movement in Stock Returns

John Ammer and Jon Wongswan*

NOTE: International Finance Discussion Papers are preliminary materials circulated to stimulate discussion and critical comment. References in publications to International Finance Discussion Papers (other than an acknowledgment that the writer has had access to unpublished material) should be cleared with the author or authors. Recent IFDPs are available on the Web at http://www.federalreserve.gov/pubs/ifdp/. This paper can be downloaded without charge from the Social Science Research Network electronic library at http://www.ssrn.com/

Abstract:

This paper examines the relative importance of global, country-specific, and industry-specific factors in both the cash flow and discount rate components of equity returns between 1995 and 2003. Our framework draws upon previously separate literatures on country versus industry effects and (forward-looking) cash flow versus discount rate components of equity return innovations. We apply the Campbell (1991) decomposition for industry-by-country, all-country, global industry, and world market index returns so we can produce a richer characterization of same-industry and same-country effects in stock returns. Unlike previous equity return decomposition papers, we exploit information in equity analysts' earnings forecasts when projecting future variables from our reduced-form equation systems. Our findings confirm previous research that finds patterns of correlation that suggest a richer underlying structure than just a single common global factor. Furthermore, our results suggest that global, within-country, and same-industry effects are all important for both of the two key components of stock returns: news about future dividends and news about future discount rates. In particular, within-industry covariation in news about future discount rates appears to be just as important as within-country covariation in news about future discount rates. We also find that the idiosyncratic component of cash flow news is more important than the global component, while the reverse is true for news about future discount rates. Our results are broadly consistent with co-movement in future discount rates arising from perceptions of common elements of risk, rather than national market segmentation.

Keywords: International stock markets, Globalization.

JEL Classification: F36, G15

*  Senior Economist and Economist, respectively, in the Division of International Finance of the Federal Reserve Board. Email addresses for the authors are [email protected] and [email protected]. We thank Nate Clinton for research assistance and Mark Carey and Dale Henderson for helpful comments. The views in this paper are solely the responsibility of the authors and should not be interpreted as reflecting the views of the Board of Governors of the Federal Reserve System or of any other person associated with the Federal Reserve System. Return to text

1   Introduction

Although standard theories of portfolio choice dictate that risk-averse investors diversify their positions broadly, one complicating factor is that asset returns tend to be significantly correlated - a particularly well-documented stylized fact for traded equity securities. But what are the key forces driving this co-movement? Traditional asset pricing models, such as the CAPM and its multi-factor variants, offer little hint, because they take the second moments of asset returns as given, and then concern themselves with equilibrium values for the first moments. More recent studies have considered the possibility of co-movements driven by the behavior of investors, who thus participate in shaping their own investment opportunity set. For example, Kodres and Pritsker (2002) explore the consequences for price movements of hedging strategies undertaken by an optimizing risk-averse investor in a stylized setting, in some cases finding positive correlation between returns on unrelated assets. Time-varying risk premiums, as in Ferson and Harvey (1991), offer another means by which return covariation can arise essentially out of investors' choices.

In this general vein, Campbell (1991) uses the Campbell-Shiller (1988) log-linearization of the dividend yield to derive a time series framework for decomposing the variance of equity returns into several components: innovations in expected future excess returns (i.e., risk premiums), in future risk-free real interest rates (the other component of forward-going discount rates), and in future dividend cash flows. Interestingly, Campbell's derivation is independent of investors' preferences, with the only implicit behavioral assumption being a weak form of rational expectations. For aggregate U.S. stock returns, Campbell finds that news about future excess returns is the most important component. On the other hand, Vuolteenaho (2002) undertakes a similar decomposition of firm-level stock returns, and finds that they are driven primarily by cash-flow news.

Campbell and Ammer (1993) and Ammer and Mei (1996) extend this approach to multiple assets, so that the covariances between national stock returns can be characterized in terms of elements like time-varying discount rates and the value of future cash flows. Campbell and Hamao (1992) argue that if asset returns in different countries are generated by an international multivariate linear factor model, the conditional means of these excess returns must move in tandem, as linear combinations of some common risk premiums. In the extreme case of a one-factor model with fixed factor loadings (betas), any variation over time in mean returns would have to be perfectly correlated across assets.1 Thus, if national financial markets are highly integrated, we should find high correlations between future expected return innovations in different countries. As it turns out, Ammer and Mei find increased U.S.-U.K. financial integration after fixed exchanged rates were abandoned in the early 1970s. In this paper, the distinction between co-movements in discount rates on the one hand, and common movements in expected future cash flows on the other, will also be a key feature of our empirical characterization of international asset return covariation.

Our work is also related to another branch of the empirical literature on covariation in stock returns. A number of previous studies that have addressed co-movements in an international context have been concerned with the relative importance of country and industry effects as common factors, distinguishing between cross-border industry correlations and within-country inter-industry correlations. For example, Stockman (1988) examines contemporaneous co-movements in industrial production indices. However, because effects of a common shock may be asynchronous, contemporary correlations may tend to understate the degree of commonality, which complicates the exercise. As in our paper, Heston and Rouwenhorst (1994), Griffin and Karolyi (1998), and Brooks and Del Negro (2002) examine stock returns, which have the advantage of tending to reflect any common shocks without any delay. Both Heston and Rouwenhorst (1994) and Griffin and Karolyi (1998) conclude that common national factors are a substantially more important feature of stock returns than are common industry effects. However, Brooks and Del Negro (2002) argue that relaxing these earlier authors' restriction to unit factor loadings leads to estimates of an important role for both industry and country effects. In another recent paper, Catao and Timmerman (2003) extend Heston and Rouwenhorst's method for decomposing returns into country and industry effects to permit regime switching in factor volatility. They find that both industry factors and a global factor have increased their importance relative to country factors since the late 1990s. Using a different methodology to decompose volatility components, Ferreira and Gama (2004) also find evidence that industry factors have become more important relative a global and country factors since the late 1990s.

The patterns of correlation found in these industry-versus-country factor frameworks can be challenging to interpret, although some studies have linked return correlations to proxy measures of real-side economic integration. For example, Griffin and Karolyi (1998) argue that on average, industry effects explain more variation in industry index returns within the more tradable sectors of the economy. Brooks and Del Negro (2003) use firm-level data and find a positive association between a firm's global market factor exposure and the ratio of the firm's foreign sales to total sales. Similarly, Forbes and Chinn (2003) find that bilateral co-movements in national stock and bond returns between the world's five largest economies and other markets are positively associated with the corresponding direct trade flows. In contrast, their results do not offer much support for a link between asset return co-movement and several proxy measures of financial-side integration, based, for example, on flow measures of bank lending and foreign direct investment.

Our work here will also allow for country and industry factors, as well as a global factor that affects all asset returns. In particular, we will assess within a common framework the relative importance of global, country, and industry factors and the relative importance of cash flow and discount rate effects in equity return co-movements, thus bringing together two sets of distinctions. Furthermore, through an interaction of these two decompositions, our estimates lead to a detailed allocation of the covariances between pairs of return innovations. Specifically, we can attribute variance shares of the discount rate revisions and the cash flow effects to global, national, industry sector, and idiosyncratic factors. Thus, for example, we can gauge the importance of country-specific risk premiums or industry-specific innovations to cash flows. Accordingly, we offer insight into the extent of both international financial barriers and real-side industry globalization. For example, cross-border same-industry co-movement in expected cash flows could arise from exposure to common shocks to long-term profitability, whereas any within-country inter-industry co-movement in expected cash flows likely reflects common exposure to national macro-economy. On the financial side, a finding that the within-country inter-industry co-movement in expected returns is relatively important (e.g., compared to the cross-border inter-industry co-movement) would tend to suggest that discount rates include country-specific factors, possibly as a result of barriers to global integration of national financial markets. On the other hand, cross-border same-industry co-movement in expected returns could result from common exposures to changes in risk.

The next section of the paper lays out our empirical methodology, and the following section describes the variables we included in our specification and the data sources from which we drew them. Section 4 contains our main results, while Section 5 reports the outcome of a robustness check using an alternative identifying assumption, and Section 6 summarizes our conclusions.

2   Methodology

Campbell (1991) shows that rearranging the Campbell-Shiller (1988) log-linear approximation to the dividend discount model, and then applying expectation operators yields:

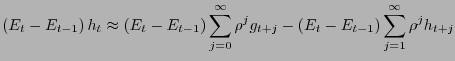
$\displaystyle \left( {E_{t} -E_{t-1} } \right) h_{t} \approx\left( {E_{t} -E_{t... ...left( {E_{t} -E_{t-1} } \right) \sum\limits_{j=1}^{\infty}{\rho^{j}h_{t+j} } $
or
$\displaystyle \left( {E_{t} -E_{t-1} } \right) h_{t} \approx G-H $
where
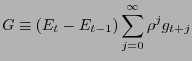
$\displaystyle G\equiv\left( {E_{t} -E_{t-1} } \right) \sum\limits_{j=0}^{\infty}{\rho ^{j}g_{t+j} } $
and
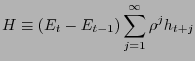
$\displaystyle H\equiv\left( {E_{t} -E_{t-1} } \right) \sum\limits_{j=1}^{\infty}{\rho ^{j}h_{t+j} } $
Here, h is the log of the one-period return (including dividends), g is the log dividend growth rate and $ \rho$ (a number slightly less than one) is a by-product of the log-linear approximation. The underlying intuition is that a higher-than-expected current stock return must reflect either an increase in the expected stream of future dividends (G $ >$ 0) or a decrease in future expected (or required) stock returns (H $ <$ 0).

We apply the relation to the return on the stock index (measured in dollars) for each industry (i) in each country (k).2 We do this by including the country-sector return (h$ _{ik})$ in a vector auto-regression (VAR), which we estimate by GMM.3 We estimate a separate system for each country-sector return (h$ _{ik})$. We deduce the properties of the unexpected part of the return from the estimated VAR parameters, as well as the discount rate component (H), which can be generated via forecasts of future returns (h$ _{ik})$. Properties of the cash flow component (G) are then inferred from the expression given above. For simplicity, unlike Campbell and Ammer (1993) and Ammer and Mei (1996), we do not include interest rate or exchange rate components in our decomposition. Those authors did not find innovations about either future interest rates or future exchange rates to be important for stock return variation.

We also include the corresponding industry return (h$ _{i})$, the corresponding country return (h$ _{k})$, and the world index return (h$ _{w})$ in the VAR, along with variables that are likely to forecast returns. These three other returns are similarly decomposed into their discount rate component (H) and cash flow component (G) using the full set of coefficient estimates from the same VAR system. We can then characterize the importance of global, national, and sectoral co-movements in G and H for the given country-sector return (h$ _{ik})$. Because the statistics of interest, such as coefficients of correlation among the derived return components, are functions of the VAR parameters, we can generate standard errors using the ``delta'' method. In particular, we take numerical derivatives of a statistic with respect to the VAR parameters and we interact this estimated gradient with the variance-covariance matrix of the estimated VAR parameters.

3   Data

Our country-sector returns pertain to the Morgan Stanley Capital International indexes, which in recent years have used the GICS industry classification system (also adopted recently by the S&P 500). Data have been reconstructed back to the beginning of 1995. Our proximate data source, the I/B/E/S Industry and Sector Aggregates (ISA) reports mid-month prices (the Tuesday before the third Friday) and dividend yields from which we construct monthly return series. The ISA also reports information about equity analysts' earnings forecasts that has been aggregated to the index level. We use the ratio of 12-month-ahead expected index earnings to the index price for the national and sectoral indexes as additional variables in the VAR. This use of survey expectations from I/B/E/S to help measure innovations in expected (or required) future stock returns in a Vector Auto-Regression framework is something of a departure from prior research on return variance decompositions, although similar variables have been used in literature that focuses on the mean of the equity premium, such as Claus and Thomas (2001). We also incorporate two more common variables for forecasting returns into the framework: the World Index dividend-price ratio and the yield spread of Baa bonds over 10-year U.S. Treasuries. Including the four returns, we have eight variables in each of the VAR systems that we estimate.

The ISA also includes figures on analysts' long-term earnings growth forecasts, which in theory ought to convey information about future returns. Nevertheless, in practice, we found that instruments based on the long-term estimates did not improve the power of our VARs to forecast returns.

Table 1 shows summary statistics (the individual correlation coefficients are listed in appendix Table A1) for the raw correlations of country-sector returns with the corresponding industry return (h$ _{i})$, the corresponding country return (h$ _{k})$, and the world index return (h$ _{w})$. Our sample period is March 1995 to August 2003. On average, the national and sectoral return correlations are equal at 66 percent, distinctly higher than the 44 percent average correlation of our 71 country-sector returns with the MSCI World Index return. There is some variation in these correlations. Our Japanese country-sectors, for example, tend to have the highest national correlations but the weakest global and sectoral correlations. Meanwhile, all three correlations tend to be high for the Financial and the Discretionary Consumer Sectors, but low for Utilities. The next section examines when variation in these correlations is driven by the discount-rate component of returns, and when it arises from the pattern of co-movements in expected cash flows.

4   Results

Tables 2 and 3 contain a similar set of summary results for correlations among our derived cash-flow (G) and discount-rate (H) components of index returns.4 The full results in appendix tables A2 and A3 include standard errors in parentheses. The weak sectoral and global correlations for Japanese country-sectors appear to be associated more with the discount-rate component than the cash-flow component of returns. However, most other country-sectors tend to be more closely linked through their discount-rate components than through their cash-flow components, suggesting a higher degree of financial integration than of common forces driving long-term dividend growth. The fact that the average sectoral correlation of discount-rate components is slightly higher than the average national correlation is further evidence against national segmentation, suggesting instead that a pattern of co-movements in perceived risks underlies the results. Interestingly, we find a larger role for industry effects than do Heston and Rouwenhorst (1994) and Griffin and Karolyi (1998) despite our using a relatively coarse classification that divides the firms underlying our sample into only 10 groupings. However, the importance of the role we infer for sectoral co-movements is consistent with the findings by Ferreira and Gama (2004) and Carrier, Errunza, and Sarkissian (2003) that common industry components were a much more important source of stock return variation from the mid-1990s than they had been before.

Tables 4, 5, 6, and 7 show these results in a different way. Table 4 (with full results and standard errors in appendix table A5) is based on a Cholesky-style orthogonalization of the cash-flow components (G). The global cash-flow component appears first in the Cholesky ``ordering'', meaning that common movements between the global G and the country, sector, or country-sector G are taken to be part of the global G. The country and sector G come next, and the country-sector return is listed last. Thus, movements in the country-sector G are taken to be ``idiosyncratic'' only to the extent that they are orthogonal to the global, country, and sector G components. Given this ordering, we can infer a variance decomposition of the country-sector G (into global, country, sector, and idiosyncratic pieces) from the estimated VAR coefficients.

The results show that on average, global, national, and sectoral co-movements each account for roughly 20 percent of the volatility of a country-sector's G component, with idiosyncratic variation of about 40 percent constituting the balance. Global co-movement in G is particularly weak for Italy, Consumer Staples, and Utilities, and strongest for Information Technology. The results here appear to be broadly consistent with the argument by Griffin and Karolyi (1998) that tradable sectors are more sensitive to global product market conditions than non-tradable sectors (Consumer Staples includes supermarkets and there is little international trade in electric power, for example).

Table 5 (with full results and standard errors in appendix table A6) shows results for an alternative ordering in which the sector G precedes the country G. The estimates are broadly similar to Table 4. On average, however, the earlier position in the Cholesky ordering shifts a few percentage points of the variance share to the sectoral G at the expense of the country G.

Tables 6 and 7 (with full results and standard errors in appendix tables A7 and A8) show analogous orthogonalized decompositions of the of the country-sector H component. Compared to the decompositions of G in Tables 4 and 5, the global component of H is higher (averaging 40 percent) and the idiosyncratic part of H is less important, typically accounting for 20 percent of the time variation in future expected returns for a country-sector index. Again, these results are broadly consistent with a high degree of equity market integration. However, the relative importance of sectoral and national components is more sensitive to the Cholesky ordering in this case. Nevertheless, we find a sizable variance share assigned to sectoral co-movements for country-sectors in the materials sector, and to a lesser extent in the energy sector. This may be a manifestation of a globalized commodity cycle and its implications for common changing perceptions of the riskiness of participating firms.

Table 8 shows estimates obtained one more way - it puts these orthogonalized decompositions of G and H together with the basic Campbell (1991) decomposition of return variance into the variance of G, the variance of H, and a covariance term. Individual results and standard errors for the three-part Campbell decomposition of country sector returns are in appendix table A4. Consistent with the results of Malliaropulos (1998) for a similar group of countries over a longer sample period, as well as Vuolteenaho's (2002) findings for individual U.S. stocks, cash flow news typically accounts for a large share of the return variance. Many of the estimates for the covariance of G and -H are negative (although in most cases not statistically distinguishable from zero with 95 percent confidence), suggesting a weak positive association between revisions to projected growth and revisions to required returns. At least on a global basis, this seems consistent with the likely effect from shocks to the investment opportunity set.

The combination results in a grand nine-part decomposition for which we show summary statistics in Table 8. In allocating among the G components, we use the mean of the Table 4 and Table 5 results, rather than choosing between the two orderings. Similarly, H shares are based on the mean of the Table 6 and Table 7 results. On average, idiosyncratic cash flow news appears to be the most important driver of country-sector returns (comprising 36 percent of the variance), with global, sector, and country-specific cash flow news and global revisions to future required returns (the world discount-rate factor) also accounting for double-digit shares.

5   Robustness Check: An Alternative Identifying Assumption

Here, we will check the robustness of our main results by taking an entirely different approach to identifying the G and H components in the Campbell relation that makes use of the I/B/E/S earnings estimates data. Specifically, analyst's revisions in earnings expectations generally should reflect changes in views about future earnings and dividends, and not changes in future risk premiums. Accordingly, we regress each stock return on current and next period's (to allow for analysts' delays in incorporating new information, which have been elsewhere documented) revisions to earnings expectations. Our specification includes their revisions to expected earnings for the next fiscal year for the corresponding industry and country indexes and the World index, as well as for the country-sector index in question. G$ _{k,i}$ here is the fitted value from the regression. In this case, G$ _{k,i}$ is orthogonal to H$ _{k,j}$ by construction. We estimate these regressions to get G and H for each of the 71 country-sector indexes, and for the world, country, and industry indexes.

Correlations of the constructed country-sector cash-flow (G) components with the corresponding country, sector, and global G components are summarized in Table 9. Reassuringly, the results here are broadly similar to the corresponding figures in Table 2 that were based on less direct estimates of the cash-flow component of returns. In particular, the national and sectoral correlations are distinctly higher than the global correlations, typically. The sectoral correlations run a little higher in Table 9, with the strongest sector effects showing up for Information Technology, Telecom, Financials, and Materials country-sectors.

The correlations in the constructed discount-rate (H) components (summarized in Table 10) are also broadly similar to their more directly derived counterparts reported in Table 3. Notably, we still see weak sectoral and global correlations for the discount-rate components of Japanese country-sector returns, hinting at a relative lack of global integration.

6   Conclusions

Our findings confirm previous research that finds patterns of correlation that suggest a richer underlying structure than just a single common global factor. Furthermore, our results suggest that global, within-country, and same-industry effects are all important for both of the two key components of stock returns - news about future dividends and news about future discount rates. In particular, within-industry covariation in news about future discount rates appears to be just as important as within-country covariation in the same. This result is consistent with co-movement (other than global co-movement) in future discount rates arising from perceptions of common elements of risk, rather than national market segmentation. Our results also suggest that international stock prices are driven at least in part by multiple time-varying risk factors, and their associated risk premiums. We also find that the idiosyncratic component of cash flow news is more important than the global component, while the reverse is true for news about future discount rates. This fact is also consistent with a significant degree of global equity market integration.

References

Ammer, John and Jianping Mei (1996), ``Measuring International Economic Linkages with Stock Market Data,'' Journal of Finance 51.

Brooks, Robin and Marco Del Negro (2002), ``International Diversification Strategies,'' Federal Reserve Bank of Atlanta Working Paper 2002-23.

Brooks, Robin and Marco Del Negro (2003), ``Firm-Level Evidence on International Stock Market Comovement,'' Federal Reserve Bank of Atlanta Working Paper 2003-8.

Campbell, John Y. (1991), ``A Variance Decomposition for Stock Returns,'' the H.G. Johnson Lecture to the Royal Economic Society, Economic Journal, 101, 157-179.

Campbell, John Y. and John Ammer (1993), ``What moves the stock and bond markets? A Variance Decomposition for Long-term Asset Returns,'' Journal of Finance, 48, 3-37.

Campbell, John Y. and Yasushi Hamao (1992), ``Predictable returns in the United States and Japan: A Study of Long Term Capital Markets Integration,'' Journal of Finance, 47, 43-70.

Campbell, John Y. and Robert J. Shiller (1988), ``The dividend-price ratio and expectations of future dividends and discount factors,'' Review of Financial Studies, 1, 195-228.

Carrieri, Francesca, Vihang Errunza, and Sergei Sarkissian (2003), ``The Dynamics of Geographic versus Sectoral Diversification: A Casual Explanation,'' manuscript, McGill University (October).

Catao, Luis, and Timmerman, Allan, 2003, ``Country and Industry Dynamics in Stock Returns,'' IMF working paper WP/03/52.

Claus, James and Jacob Thomas (2001), ``Equity Premiums as Low as Three Percent? Evidence from Analysts' Earnings Forecasts for Domestic and International Stock Markets,'' Journal of Finance 56, 1629-1666.

Connor, Greg and Robert Korajczyk (1988), ``Risk and Return in an Equilibrium APT: Application of a New Test Methodology,'' Journal of Financial Economics, 21, 255-289.

Ferreira, Miguel A. and Paulo M. Gama (2004), ``Have World, Country, and Industry Risks Changed Over Time? An Investigation of the Developed Stock Markets Volatility,'' Journal of Financial and Quantitative Analysis, forthcoming.

Ferson, Wayne and Campbell Harvey (1991), ``The Variation of Economic Risk Premiums,'' Journal of Political Economy 99, 385-415.

Forbes, Kristin, and Chinn, Menzie, 2003, ``A Decomposition of Global linkages in Financial Markets over Time,'' NBER working paper 9555.

Griffin, John M. and G. Andrew Karolyi (1998), ``Another Look at the Role of the Industrial Structure of Markets for International Diversification Strategies,'' Journal of Financial Economics 50(3):351-373.

Heston, Steven L. and K. Geert Rouwenhorst (1994), ``Does Industrial Structure Explain the Benefits of International Diversification?'' Journal of Financial Economics 36, 3-27.

Kodres, Laura E., and Matt Pritsker (2002), ``A Rational Expectations Model of Financial Contagion,'' Journal of Finance 57, 769-799.

Malliaropulos, Dmitrios (1998), ``Excess Stock Returns and News: Evidence form European Markets,'' European Financial Management 4(1), 29-46.

Page, Sebastien and Anne-Sophie Van Royen (2001), ``The Multiple Dimensions of Asset Allocation: Countries, Sectors, or Factors?'' State Street Associates working paper.

Stockman, Alan (1988) ``Sectoral and national aggregate disturbances to industrial output in seven European countries,'' Journal of Money and Economics 21, 387-409.

Vuolteenaho, Tuomo, 2002, ``What Drives Firm-Level Stock Returns?'' Journal of Finance 57, No.1, p.233-264

 

Table 1: Correlations of Dollar-Denominated Returns - Summary Results

Industry-Sector versus Corresponding Broader Aggregates

average for: N global national sectoral
Germany 9 0.45 0.64 0.60
France 9 0.51 0.66 0.66
Italy 8 0.40 0.74 0.54
Netherlands 7 0.48 0.65 0.71
U.K. 10 0.40 0.60 0.71
Japan 9 0.32 0.76 0.51
Canada 9 0.42 0.54 0.66
U.S. 10 0.53 0.67 0.89
Energy 7 0.30 0.56 0.71
Materials 8 0.41 0.61 0.71
Industrials 8 0.54 0.77 0.65
Discretionary 8 0.61 0.77 0.73
Staples 8 0.33 0.56 0.58
Healthcare 6 0.39 0.61 0.59
Financials 8 0.54 0.82 0.74
InfoTech 7 0.54 0.69 0.71
Telecom 4 0.45 0.69 0.72
Utilities 7 0.24 0.48 0.52
all of above 71 0.44 0.66 0.66

 

Table 2: Correlations of Cash-Flow-Revision Components (G) of Returns - Summary Results

Industry-Sector versus Corresponding Broader Aggregates

average for: N global national sectoral
Germany 9 0.38 0.55 0.52
France 9 0.45 0.64 0.61
Italy 8 0.12 0.56 0.31
Netherlands 7 0.52 0.63 0.63
U.K. 10 0.41 0.51 0.66
Japan 9 0.34 0.77 0.49
Canada 9 0.29 0.45 0.47
U.S. 10 0.51 0.60 0.86
Energy 7 0.28 0.51 0.66
Materials 8 0.41 0.61 0.65
Industrials 8 0.46 0.76 0.53
Discretionary 8 0.54 0.67 0.60
Staples 8 0.23 0.44 0.47
Healthcare 6 0.31 0.49 0.47
Financials 8 0.45 0.75 0.64
InfoTech 7 0.58 0.69 0.73
Telecom 4 0.32 0.54 0.69
Utilities 7 0.15 0.33 0.36
all of above 71 0.38 0.59 0.58

Table 3: Correlations of Discount-Rate Components (H) of Returns - Summary Results

Industry-Sector versus Corresponding Broader Aggregates

average for: N global national sectoral
Germany 9 0.79 0.85 0.75
France 9 0.65 0.74 0.73
Italy 8 0.60 0.66 0.75
Netherlands 7 0.56 0.77 0.51
U.K. 10 0.68 0.74 0.91
Japan 9 0.15 0.58 0.36
Canada 9 0.31 0.50 0.59
U.S. 10 0.58 0.70 0.92
Energy 7 0.29 0.43 0.58
Materials 8 0.21 0.34 0.63
Industrials 8 0.63 0.85 0.68
Discretionary 8 0.74 0.81 0.76
Staples 8 0.56 0.66 0.67
Healthcare 6 0.51 0.79 0.54
Financials 8 0.74 0.89 0.84
InfoTech 7 0.63 0.86 0.83
Telecom 4 0.83 0.90 0.87
Utilities 7 0.36 0.46 0.66
all of above 71 0.54 0.69 0.70

Table 4: Orthogonalized Decomposition of Cash Flow News Component (G) - Summary Results

Cholesky Ordering: Global, National, Sectoral, Idiosyncratic (shown as contribution to variance)

average for: N global national sectoral idiosyncratic
Germany 9 0.16 0.19 0.11 0.53
France 9 0.21 0.22 0.16 0.40
Italy 8 0.07 0.34 0.07 0.52
Netherlands 7 0.29 0.14 0.14 0.42
U.K. 10 0.20 0.13 0.23 0.43
Japan 9 0.18 0.51 0.06 0.25
Canada 9 0.18 0.17 0.16 0.49
U.S. 10 0.29 0.11 0.41 0.19
Energy 7 0.16 0.20 0.33 0.32
Materials 8 0.20 0.21 0.22 0.36
Industrials 8 0.28 0.33 0.07 0.32
Discretionary 8 0.32 0.19 0.06 0.43
Staples 8 0.08 0.17 0.21 0.55
Healthcare 6 0.14 0.20 0.21 0.45
Financials 8 0.21 0.39 0.15 0.25
InfoTech 7 0.35 0.20 0.14 0.31
Telecom 4 0.11 0.21 0.33 0.35
Utilities 7 0.08 0.13 0.13 0.65
all of above 71 0.20 0.23 0.18 0.40

 

Table 5: Orthogonalized Decomposition of Cash Flow News Component (G) - Summary Results
Cholesky Ordering: Global, Sectoral, National, Idiosyncratic (shown as contribution to variance)

average for: N global national sectoral idiosyncratic
Germany 9 0.16 0.15 0.15 0.53
France 9 0.21 0.18 0.20 0.40
Italy 8 0.07 0.29 0.13 0.52
Netherlands 7 0.29 0.09 0.19 0.42
U.K. 10 0.20 0.09 0.27 0.43
Japan 9 0.18 0.41 0.16 0.25
Canada 9 0.18 0.11 0.22 0.49
U.S. 10 0.29 0.06 0.46 0.19
Energy 7 0.16 0.12 0.40 0.32
Materials 8 0.20 0.15 0.29 0.36
Industrials 8 0.28 0.27 0.13 0.32
Discretionary 8 0.32 0.15 0.09 0.43
Staples 8 0.08 0.14 0.23 0.55
Healthcare 6 0.14 0.15 0.26 0.45
Financials 8 0.21 0.32 0.22 0.25
InfoTech 7 0.35 0.12 0.21 0.31
Telecom 4 0.11 0.13 0.41 0.35
Utilities 7 0.08 0.12 0.15 0.65
all of above 71 0.20 0.17 0.23 0.40

 


Table 6: Orthogonalized Decomposition of Discount-Rate Component (H) - Summary of Results

Cholesky Ordering: Global, National, Sectoral, Idiosyncratic (shown as contribution to variance)

average for: N global national sectoral idiosyncratic
Germany 9 0.63 0.14 0.04 0.18
France 9 0.47 0.27 0.11 0.15
Italy 8 0.44 0.20 0.13 0.23
Netherlands 7 0.45 0.33 0.05 0.16
U.K. 10 0.51 0.11 0.28 0.10
Japan 9 0.09 0.38 0.12 0.41
Canada 9 0.18 0.37 0.16 0.28
U.S. 10 0.41 0.19 0.30 0.10
Energy 7 0.18 0.27 0.19 0.35
Materials 8 0.21 0.22 0.38 0.20
Industrials 8 0.46 0.34 0.05 0.16
Discretionary 8 0.57 0.18 0.09 0.16
Staples 8 0.40 0.15 0.22 0.23
Healthcare 6 0.33 0.33 0.09 0.25
Financials 8 0.58 0.23 0.10 0.09
InfoTech 7 0.44 0.36 0.11 0.09
Telecom 4 0.69 0.16 0.02 0.12
Utilities 7 0.21 0.21 0.25 0.34
all of above 71 0.40 0.25 0.16 0.20

 

Table 7: Orthogonalized Decomposition of Discount-Rate Component (H) - Summary of Results

Cholesky Ordering: Global, Sectoral, National, Idiosyncratic (shown as contribution to variance)

average for: N global national sectoral idiosyncratic
Germany 9 0.63 0.09 0.10 0.18
France 9 0.47 0.15 0.24 0.15
Italy 8 0.44 0.14 0.19 0.23
Netherlands 7 0.45 0.22 0.17 0.16
U.K. 10 0.51 0.02 0.37 0.10
Japan 9 0.09 0.27 0.23 0.41
Canada 9 0.18 0.23 0.30 0.28
U.S. 10 0.41 0.03 0.46 0.10
Energy 7 0.18 0.06 0.40 0.35
Materials 8 0.21 0.10 0.49 0.20
Industrials 8 0.46 0.27 0.11 0.16
Discretionary 8 0.57 0.17 0.10 0.16
Staples 8 0.40 0.08 0.29 0.23
Healthcare 6 0.33 0.26 0.15 0.25
Financials 8 0.58 0.14 0.19 0.09
InfoTech 7 0.44 0.10 0.37 0.09
Telecom 4 0.69 0.10 0.09 0.12
Utilities 7 0.21 0.09 0.37 0.34
all of above 71 0.40 0.14 0.26 0.20

 


Table 8: average combination of GCSI and G vs. H breakdown by country and sector

(expressed as fractional contribution to stock return variance)

average for: N global cash flow news global discount rate news country cash flow news country discount rate news industry cash flow news industry discount rate news residual cash flow news residual discount rate news cross terms
Germany 9 0.15 0.23 0.16 0.04 0.14 0.03 0.46 0.04 -0.25
France 9 0.18 0.09 0.17 0.04 0.16 0.03 0.35 0.03 -0.05
Italy 8 0.06 0.15 0.27 0.07 0.08 0.04 0.41 0.06 -0.15
Netherlands 7 0.31 0.14 0.11 0.07 0.15 0.02 0.40 0.05 -0.24
U.K. 10 0.20 0.17 0.08 0.02 0.20 0.09 0.34 0.03 -0.14
Japan 9 0.22 0.01 0.55 0.04 0.13 0.03 0.29 0.05 -0.32
Canada 9 0.20 0.05 0.15 0.10 0.17 0.08 0.51 0.07 -0.34
U.S. 10 0.21 0.09 0.05 0.02 0.28 0.08 0.14 0.02 0.11
Energy 7 0.14 0.04 0.16 0.02 0.31 0.05 0.27 0.06 -0.05
Materials 8 0.17 0.05 0.17 0.03 0.19 0.08 0.31 0.03 -0.03
Industrials 8 0.31 0.10 0.30 0.09 0.11 0.02 0.34 0.04 -0.31
Discretionary 8 0.26 0.15 0.15 0.02 0.06 0.02 0.36 0.04 -0.06
Staples 8 0.07 0.10 0.13 0.03 0.12 0.07 0.42 0.03 0.01
Healthcare 6 0.12 0.09 0.18 0.10 0.14 0.04 0.38 0.07 -0.11
Financials 8 0.18 0.22 0.33 0.05 0.14 0.05 0.21 0.03 -0.21
InfoTech 7 0.40 0.12 0.19 0.06 0.19 0.06 0.34 0.02 -0.39
Telecom 4 0.12 0.25 0.16 0.04 0.38 0.02 0.34 0.04 -0.35
Utilities 7 0.08 0.08 0.12 0.04 0.15 0.09 0.63 0.08 -0.27
all of above 71 0.19 0.11 0.19 0.05 0.17 0.05 0.36 0.04 -0.17

 

 

Table 9: Correlations of Cash-Flow-Revision Components (G) of Returns - Summary Results

Industry-Sector versus Corresponding Broader Aggregates

average for: N global national sectoral
Germany 9 0.52 0.58 0.60
France 9 0.45 0.59 0.62
Italy 8 0.29 0.58 0.45
Netherlands 7 0.49 0.61 0.61
U.K. 10 0.31 0.38 0.72
Japan 9 0.34 0.54 0.55
Canada 9 0.39 0.47 0.60
U.S. 10 0.36 0.57 0.71
Energy 7 0.35 0.47 0.71
Materials 8 0.41 0.59 0.75
Industrials 8 0.59 0.81 0.58
Discretionary 8 0.65 0.71 0.71
Staples 8 0.16 0.30 0.14
Healthcare 6 0.28 0.44 0.46
Financials 8 0.49 0.67 0.77
InfoTech 7 0.64 0.70 0.80
Telecom 4 0.30 0.46 0.79
Utilities 7 -0.05 0.11 0.46
all of above 71 0.39 0.54 0.61
Note: Results (unlike Table 2) are based on decomposition which treats the part of the return that is explained by I/B/E/S earnings forecast revisions as the cash-flow news component. The two components (G and H) are in this case orthogonal by construction.

 

Table 10: Correlations of Discount-Rate Components (H) of Returns - Summary Results

Industry-Sector versus Corresponding Broader Aggregates

average for: N global national sectoral
Germany 9 0.38 0.60 0.54
France 9 0.46 0.63 0.62
Italy 8 0.38 0.71 0.52
Netherlands 7 0.39 0.61 0.67
U.K. 10 0.33 0.56 0.66
Japan 9 0.27 0.73 0.48
Canada 9 0.38 0.52 0.63
U.S. 10 0.46 0.66 0.85
Energy 7 0.28 0.54 0.68
Materials 8 0.33 0.55 0.69
Industrials 8 0.48 0.73 0.59
Discretionary 8 0.55 0.74 0.68
Staples 8 0.33 0.57 0.57
Healthcare 6 0.38 0.60 0.58
Financials 8 0.42 0.73 0.69
InfoTech 7 0.40 0.60 0.61
Telecom 4 0.37 0.68 0.66
Utilities 7 0.26 0.49 0.51
all of above 71 0.38 0.63 0.63
Note: Results (unlike Table 3) are based on decomposition which treats the part of the return that is explained by I/B/E/S earnings forecast revisions as the cash-flow news component. The two components (G and H) are in this case orthogonal by construction.

 

Table A1 (2 pages): Correlations of Dollar-Denominated Returns (March 1995 to August 2003)

Industry-Sector versus Corresponding Broader Aggregates

country sector global national sectoral
Germany Materials 0.55 0.72 0.70
Germany Industrials 0.62 0.79 0.65
Germany Discretionary 0.57 0.75 0.67
Germany Staples 0.26 0.45 0.34
Germany Healthcare 0.37 0.49 0.51
Germany Financials 0.59 0.86 0.73
Germany InfoTech 0.40 0.60 0.55
Germany Telecom 0.41 0.64 0.73
Germany Utilities 0.25 0.44 0.52
France Energy 0.37 0.58 0.72
France Materials 0.46 0.52 0.76
France Industrials 0.56 0.76 0.58
France Discretionary 0.73 0.85 0.77
France Staples 0.55 0.66 0.51
France Healthcare 0.45 0.50 0.61
France Financials 0.60 0.73 0.79
France InfoTech 0.54 0.72 0.69
France Utilities 0.35 0.65 0.49
Italy Energy 0.21 0.56 0.51
Italy Materials 0.36 0.68 0.56
Italy Industrials 0.43 0.79 0.41
Italy Discretionary 0.59 0.85 0.69
Italy Staples 0.27 0.58 0.38
Italy Financials 0.58 0.87 0.65
Italy Telecom 0.49 0.86 0.68
Italy Utilities 0.28 0.72 0.44
Netherlands Energy 0.38 0.65 0.88
Netherlands Materials 0.32 0.52 0.59
Netherlands Industrials 0.56 0.70 0.64
Netherlands Discretionary 0.52 0.59 0.62
Netherlands Staples 0.40 0.64 0.82
Netherlands Financials 0.60 0.83 0.78
Netherlands InfoTech 0.57 0.64 0.65
U.K. Energy 0.30 0.54 0.80
U.K. Materials 0.41 0.48 0.83
U.K. Industrials 0.49 0.62 0.62
U.K. Discretionary 0.62 0.64 0.75
U.K. Staples 0.20 0.49 0.75
U.K. Healthcare 0.38 0.69 0.74
U.K. Financials 0.52 0.87 0.80
U.K. InfoTech 0.46 0.46 0.58
U.K. Telecom 0.42 0.66 0.72
U.K. Utilities 0.20 0.54 0.50
Japan Energy 0.23 0.66 0.40
Japan Materials 0.35 0.83 0.58
Japan Industrials 0.37 0.94 0.62
Japan Discretionary 0.51 0.88 0.68
Japan Staples 0.25 0.77 0.36
Japan Healthcare 0.34 0.74 0.41
Japan Financials 0.25 0.87 0.48
Japan InfoTech 0.52 0.77 0.73
Japan Utilities 0.06 0.43 0.35
Canada Energy 0.24 0.44 0.75
Canada Materials 0.34 0.49 0.83
Canada Industrials 0.57 0.67 0.75
Canada Discretionary 0.62 0.71 0.73
Canada Staples 0.36 0.36 0.52
Canada Healthcare 0.31 0.58 0.31
Canada Financials 0.53 0.63 0.78
Canada InfoTech 0.60 0.82 0.77
Canada Utilities 0.19 0.13 0.50
U.S. Energy 0.38 0.50 0.92
U.S. Materials 0.48 0.61 0.85
U.S. Industrials 0.74 0.89 0.91
U.S. Discretionary 0.74 0.86 0.92
U.S. Staples 0.37 0.52 0.93
U.S. Healthcare 0.47 0.68 0.95
U.S. Financials 0.64 0.87 0.88
U.S. InfoTech 0.69 0.80 0.97
U.S. Telecom 0.47 0.58 0.75
U.S. Utilities 0.35 0.42 0.82

 


Table A2 (2 pages): Correlations of Cash-Flow-Revision Components (G) of Returns

Industry-Sector versus Corresponding Broader Aggregates (standard errors in parentheses)

country sector global national sectoral
Germany Materials 0.54 ( 0.14) 0.68 ( 0.12) 0.66 ( 0.13)
Germany Industrials 0.47 ( 0.19) 0.74 ( 0.12) 0.50 ( 0.18)
Germany Discretionary 0.33 ( 0.16) 0.47 ( 0.21) 0.40 ( 0.21)
Germany Staples 0.11 ( 0.20) 0.28 ( 0.22) 0.24 ( 0.22)
Germany Healthcare 0.40 ( 0.23) 0.36 ( 0.27) 0.63 ( 0.16)
Germany Financials 0.60 ( 0.15) 0.89 ( 0.05) 0.60 ( 0.14)
Germany InfoTech 0.23 ( 0.22) 0.58 ( 0.17) 0.46 ( 0.21)
Germany Telecom 0.43 ( 0.19) 0.63 ( 0.16) 0.84 ( 0.07)
Germany Utilities 0.31 ( 0.24) 0.31 ( 0.22) 0.38 ( 0.22)
France Energy 0.44 ( 0.27) 0.61 ( 0.18) 0.81 ( 0.09)
France Materials 0.40 ( 0.20) 0.57 ( 0.20) 0.72 ( 0.12)
France Industrials 0.38 ( 0.20) 0.73 ( 0.10) 0.29 ( 0.26)
France Discretionary 0.63 ( 0.14) 0.80 ( 0.07) 0.68 ( 0.13)
France Staples 0.39 ( 0.20) 0.46 ( 0.27) 0.60 ( 0.18)
France Healthcare 0.34 ( 0.25) 0.37 ( 0.26) 0.56 ( 0.16)
France Financials 0.49 ( 0.17) 0.71 ( 0.13) 0.70 ( 0.12)
France InfoTech 0.60 ( 0.19) 0.82 ( 0.10) 0.75 ( 0.13)
France Utilities 0.39 ( 0.24) 0.69 ( 0.13) 0.39 ( 0.27)
Italy Energy -0.14 ( 0.23) 0.21 ( 0.19) 0.14 ( 0.23)
Italy Materials 0.14 ( 0.28) 0.65 ( 0.14) 0.40 ( 0.19)
Italy Industrials -0.09 ( 0.26) 0.63 ( 0.17) -0.13 ( 0.28)
Italy Discretionary 0.55 ( 0.19) 0.81 ( 0.09) 0.68 ( 0.17)
Italy Staples 0.05 ( 0.24) 0.39 ( 0.23) 0.09 ( 0.29)
Italy Financials 0.38 ( 0.26) 0.79 ( 0.13) 0.56 ( 0.19)
Italy Telecom 0.20 ( 0.25) 0.67 ( 0.13) 0.58 ( 0.12)
Italy Utilities -0.09 ( 0.21) 0.33 ( 0.25) 0.14 ( 0.21)
Netherlands Energy 0.57 ( 0.24) 0.64 ( 0.17) 0.92 ( 0.04)
Netherlands Materials 0.60 ( 0.18) 0.72 ( 0.12) 0.46 ( 0.21)
Netherlands Industrials 0.67 ( 0.16) 0.77 ( 0.11) 0.74 ( 0.13)
Netherlands Discretionary 0.25 ( 0.26) 0.29 ( 0.27) 0.19 ( 0.26)
Netherlands Staples 0.39 ( 0.26) 0.52 ( 0.25) 0.78 ( 0.08)
Netherlands Financials 0.64 ( 0.15) 0.83 ( 0.09) 0.78 ( 0.10)
Netherlands InfoTech 0.55 ( 0.19) 0.67 ( 0.15) 0.57 ( 0.19)
U.K. Energy 0.47 ( 0.27) 0.53 ( 0.21) 0.86 ( 0.08)
U.K. Materials 0.60 ( 0.15) 0.59 ( 0.13) 0.83 ( 0.07)
U.K. Industrials 0.65 ( 0.13) 0.77 ( 0.09) 0.67 ( 0.12)
U.K. Discretionary 0.52 ( 0.18) 0.47 ( 0.19) 0.65 ( 0.14)
U.K. Staples 0.05 ( 0.20) 0.37 ( 0.18) 0.53 ( 0.13)
U.K. Healthcare 0.32 ( 0.24) 0.42 ( 0.18) 0.64 ( 0.14)
U.K. Financials 0.35 ( 0.28) 0.72 ( 0.16) 0.64 ( 0.15)
U.K. InfoTech 0.63 ( 0.13) 0.37 ( 0.27) 0.71 ( 0.14)
U.K. Telecom 0.28 ( 0.18) 0.39 ( 0.14) 0.72 ( 0.11)
U.K. Utilities 0.24 ( 0.22) 0.45 ( 0.14) 0.33 ( 0.19)
Japan Energy 0.03 ( 0.35) 0.63 ( 0.23) 0.31 ( 0.29)
Japan Materials 0.28 ( 0.26) 0.85 ( 0.07) 0.64 ( 0.15)
Japan Industrials 0.26 ( 0.28) 0.93 ( 0.03) 0.52 ( 0.20)
Japan Discretionary 0.64 ( 0.15) 0.90 ( 0.05) 0.65 ( 0.15)
Japan Staples 0.36 ( 0.22) 0.77 ( 0.11) 0.42 ( 0.19)
Japan Healthcare 0.56 ( 0.24) 0.83 ( 0.08) 0.35 ( 0.26)
Japan Financials 0.39 ( 0.25) 0.90 ( 0.06) 0.52 ( 0.20)
Japan InfoTech 0.65 ( 0.17) 0.82 ( 0.09) 0.79 ( 0.12)
Japan Utilities -0.13 ( 0.31) 0.26 ( 0.28) 0.26 ( 0.32)
Canada Energy 0.05 ( 0.32) 0.34 ( 0.28) 0.64 ( 0.19)
Canada Materials 0.23 ( 0.23) 0.33 ( 0.20) 0.64 ( 0.15)
Canada Industrials 0.65 ( 0.17) 0.72 ( 0.14) 0.81 ( 0.11)
Canada Discretionary 0.64 ( 0.14) 0.72 ( 0.10) 0.67 ( 0.13)
Canada Staples 0.35 ( 0.20) 0.41 ( 0.20) 0.26 ( 0.30)
Canada Healthcare -0.15 ( 0.31) 0.45 ( 0.26) -0.31 ( 0.30)
Canada Financials 0.30 ( 0.24) 0.52 ( 0.18) 0.55 ( 0.21)
Canada InfoTech 0.65 ( 0.16) 0.80 ( 0.11) 0.85 ( 0.08)
Canada Utilities -0.16 ( 0.22) -0.23 ( 0.27) 0.16 ( 0.21)
U.S. Energy 0.57 ( 0.23) 0.62 ( 0.21) 0.93 ( 0.04)
U.S. Materials 0.51 ( 0.21) 0.48 ( 0.23) 0.87 ( 0.06)
U.S. Industrials 0.70 ( 0.12) 0.78 ( 0.10) 0.88 ( 0.07)
U.S. Discretionary 0.78 ( 0.09) 0.86 ( 0.08) 0.89 ( 0.05)
U.S. Staples 0.19 ( 0.29) 0.29 ( 0.26) 0.86 ( 0.08)
U.S. Healthcare 0.37 ( 0.27) 0.55 ( 0.22) 0.93 ( 0.03)
U.S. Financials 0.43 ( 0.21) 0.61 ( 0.17) 0.74 ( 0.10)
U.S. InfoTech 0.72 ( 0.13) 0.76 ( 0.13) 0.97 ( 0.01)
U.S. Telecom 0.37 ( 0.23) 0.48 ( 0.23) 0.64 ( 0.16)
U.S. Utilities 0.48 ( 0.26) 0.53 ( 0.26) 0.85 ( 0.10)

 


Table A3 (2 pages): Correlations of Discount-Rate Components (H) of Returns

Industry-Sector versus Corresponding Broader Aggregates (standard errors in parentheses)

country sector global national sectoral
Germany Materials 0.90 ( 0.10) 0.88 ( 0.12) 0.75 ( 0.23)
Germany Industrials 0.82 ( 0.28) 0.86 ( 0.19) 0.89 ( 0.26)
Germany Discretionary 0.92 ( 0.08) 0.84 ( 0.24) 0.85 ( 0.15)
Germany Staples 0.57 ( 0.40) 0.55 ( 0.38) 0.34 ( 0.47)
Germany Healthcare 0.64 ( 0.37) 0.82 ( 0.18) 0.39 ( 0.43)
Germany Financials 0.77 ( 0.30) 0.94 ( 0.08) 0.71 ( 0.30)
Germany InfoTech 0.79 ( 0.32) 0.96 ( 0.07) 0.91 ( 0.15)
Germany Telecom 0.92 ( 0.11) 0.89 ( 0.14) 0.96 ( 0.04)
Germany Utilities 0.77 ( 0.22) 0.90 ( 0.13) 0.98 ( 0.03)
France Energy 0.41 ( 0.61) 0.59 ( 0.53) 0.66 ( 0.38)
France Materials 0.65 ( 0.48) 0.39 ( 0.59) 0.86 ( 0.26)
France Industrials 0.85 ( 0.29) 0.91 ( 0.13) 0.87 ( 0.23)
France Discretionary 0.90 ( 0.09) 0.85 ( 0.14) 0.88 ( 0.14)
France Staples 0.90 ( 0.21) 0.97 ( 0.04) 0.39 ( 0.35)
France Healthcare 0.60 ( 0.52) 0.77 ( 0.38) 0.53 ( 0.37)
France Financials 0.78 ( 0.29) 0.78 ( 0.22) 0.96 ( 0.08)
France InfoTech 0.49 ( 0.46) 0.84 ( 0.19) 0.77 ( 0.22)
France Utilities 0.23 ( 0.47) 0.59 ( 0.29) 0.64 ( 0.44)
Italy Energy 0.56 ( 0.41) 0.50 ( 0.39) 0.75 ( 0.37)
Italy Materials -0.15 ( 1.31) -0.03 ( 1.19) 0.66 ( 0.84)
Italy Industrials 0.62 ( 0.34) 0.81 ( 0.14) 0.67 ( 0.36)
Italy Discretionary 0.61 ( 0.43) 0.80 ( 0.29) 0.63 ( 0.44)
Italy Staples 0.85 ( 0.22) 0.64 ( 0.36) 0.91 ( 0.17)
Italy Financials 0.78 ( 0.31) 0.85 ( 0.20) 0.91 ( 0.15)
Italy Telecom 0.76 ( 0.26) 0.95 ( 0.06) 0.84 ( 0.18)
Italy Utilities 0.71 ( 0.30) 0.80 ( 0.27) 0.61 ( 0.42)
Netherlands Energy 0.53 ( 0.51) 0.84 ( 0.18) 0.93 ( 0.08)
Netherlands Materials -0.18 ( 0.56) 0.43 ( 0.44) -0.58 ( 0.43)
Netherlands Industrials 0.25 ( 0.44) 0.63 ( 0.32) 0.15 ( 0.53)
Netherlands Discretionary 0.76 ( 0.24) 0.75 ( 0.31) 0.57 ( 0.32)
Netherlands Staples 0.76 ( 0.32) 0.90 ( 0.09) 0.85 ( 0.18)
Netherlands Financials 0.92 ( 0.11) 0.97 ( 0.04) 0.88 ( 0.12)
Netherlands InfoTech 0.89 ( 0.17) 0.90 ( 0.13) 0.77 ( 0.27)
U.K. Energy 0.65 ( 0.47) 0.89 ( 0.16) 0.90 ( 0.11)
U.K. Materials 0.38 ( 0.57) 0.41 ( 0.46) 0.98 ( 0.03)
U.K. Industrials 0.91 ( 0.13) 0.94 ( 0.07) 0.92 ( 0.14)
U.K. Discretionary 0.92 ( 0.09) 0.81 ( 0.17) 0.92 ( 0.09)
U.K. Staples 0.52 ( 0.43) 0.50 ( 0.28) 0.88 ( 0.12)
U.K. Healthcare 0.80 ( 0.24) 0.92 ( 0.12) 0.93 ( 0.04)
U.K. Financials 0.91 ( 0.15) 0.94 ( 0.06) 0.94 ( 0.05)
U.K. InfoTech 0.53 ( 0.59) 0.59 ( 0.63) 0.85 ( 0.27)
U.K. Telecom 0.84 ( 0.21) 0.86 ( 0.16) 0.85 ( 0.15)
U.K. Utilities 0.38 ( 0.43) 0.53 ( 0.27) 0.90 ( 0.15)
Japan Energy -0.13 ( 0.99) 0.14 ( 0.92) -0.42 ( 0.67)
Japan Materials -0.34 ( 0.91) 0.25 ( 0.95) 0.58 ( 0.65)
Japan Industrials 0.30 ( 0.79) 0.93 ( 0.11) 0.53 ( 0.59)
Japan Discretionary 0.49 ( 0.95) 0.88 ( 0.18) 0.60 ( 1.02)
Japan Staples 0.37 ( 0.91) 0.42 ( 1.11) 0.46 ( 1.06)
Japan Healthcare 0.20 ( 0.84) 0.65 ( 0.81) 0.02 ( 0.96)
Japan Financials 0.32 ( 0.76) 0.91 ( 0.16) 0.41 ( 0.80)
Japan InfoTech 0.22 ( 0.65) 0.90 ( 0.29) 0.71 ( 0.31)
Japan Utilities -0.03 ( 0.80) 0.10 ( 1.04) 0.34 ( 0.65)
Canada Energy -0.18 ( 0.83) -0.38 ( 0.60) 0.32 ( 0.77)
Canada Materials 0.17 ( 0.46) -0.13 ( 0.45) 0.92 ( 0.14)
Canada Industrials 0.47 ( 0.52) 0.83 ( 0.31) 0.46 ( 0.50)
Canada Discretionary 0.64 ( 0.40) 0.89 ( 0.13) 0.63 ( 0.40)
Canada Staples -0.08 ( 0.67) 0.69 ( 0.40) 0.54 ( 0.48)
Canada Healthcare 0.12 ( 0.64) 0.79 ( 0.27) 0.37 ( 0.34)
Canada Financials 0.62 ( 0.29) 0.81 ( 0.16) 0.93 ( 0.08)
Canada InfoTech 0.66 ( 0.32) 0.96 ( 0.07) 0.82 ( 0.15)
Canada Utilities 0.32 ( 0.49) 0.03 ( 0.55) 0.33 ( 0.63)
U.S. Energy 0.17 ( 0.69) 0.46 ( 0.50) 0.93 ( 0.10)
U.S. Materials 0.26 ( 0.51) 0.48 ( 0.33) 0.87 ( 0.10)
U.S. Industrials 0.81 ( 0.22) 0.91 ( 0.12) 0.91 ( 0.09)
U.S. Discretionary 0.67 ( 0.26) 0.70 ( 0.20) 0.96 ( 0.04)
U.S. Staples 0.60 ( 0.36) 0.64 ( 0.30) 0.99 ( 0.03)
U.S. Healthcare 0.74 ( 0.32) 0.82 ( 0.19) 0.99 ( 0.01)
U.S. Financials 0.81 ( 0.17) 0.92 ( 0.10) 0.96 ( 0.05)
U.S. InfoTech 0.83 ( 0.19) 0.91 ( 0.14) 0.95 ( 0.07)
U.S. Telecom 0.80 ( 0.25) 0.92 ( 0.15) 0.81 ( 0.31)
U.S. Utilities 0.16 ( 0.57) 0.30 ( 0.47) 0.84 ( 0.21)

 

Table A4 (2 pages): Variance Decompositions of Country-Sector Returns

Each decomposition based on separately estimated VAR system (standard errors in parentheses)

country sector cash flow news covariance term discount rate news
Germany Materials 0.83 ( 0.23) -0.22 ( 0.37) 0.38 ( 0.29)
Germany Industrials 0.78 ( 0.26) 0.08 ( 0.23) 0.14 ( 0.15)
Germany Discretionary 0.89 ( 0.21) -0.20 ( 0.37) 0.30 ( 0.25)
Germany Staples 0.69 ( 0.14) 0.17 ( 0.15) 0.14 ( 0.09)
Germany Healthcare 0.78 ( 0.30) 0.05 ( 0.39) 0.17 ( 0.15)
Germany Financials 1.02 ( 0.42) -0.39 ( 0.51) 0.37 ( 0.24)
Germany InfoTech 1.02 ( 0.38) -0.47 ( 0.62) 0.46 ( 0.32)
Germany Telecom 1.36 ( 0.46) -0.90 ( 0.76) 0.54 ( 0.42)
Germany Utilities 0.86 ( 0.30) -0.34 ( 0.58) 0.48 ( 0.33)
France Energy 0.79 ( 0.34) 0.11 ( 0.39) 0.10 ( 0.09)
France Materials 0.83 ( 0.28) 0.08 ( 0.26) 0.09 ( 0.14)
France Industrials 0.82 ( 0.23) 0.08 ( 0.21) 0.10 ( 0.09)
France Discretionary 0.55 ( 0.21) 0.26 ( 0.13) 0.19 ( 0.14)
France Staples 0.87 ( 0.37) -0.19 ( 0.54) 0.33 ( 0.22)
France Healthcare 0.95 ( 0.38) -0.16 ( 0.52) 0.21 ( 0.19)
France Financials 0.73 ( 0.26) 0.15 ( 0.24) 0.12 ( 0.11)
France InfoTech 1.36 ( 0.73) -0.64 ( 1.02) 0.28 ( 0.32)
France Utilities 0.87 ( 0.42) -0.12 ( 0.60) 0.25 ( 0.23)
Italy Energy 0.66 ( 0.20) -0.03 ( 0.43) 0.37 ( 0.28)
Italy Materials 0.93 ( 0.31) 0.02 ( 0.31) 0.04 ( 0.06)
Italy Industrials 1.01 ( 0.35) -0.75 ( 0.73) 0.74 ( 0.45)
Italy Discretionary 0.79 ( 0.36) 0.10 ( 0.36) 0.11 ( 0.08)
Italy Staples 0.64 ( 0.17) 0.12 ( 0.18) 0.24 ( 0.20)
Italy Financials 1.01 ( 0.43) -0.31 ( 0.50) 0.30 ( 0.23)
Italy Telecom 0.71 ( 0.32) -0.12 ( 0.36) 0.41 ( 0.24)
Italy Utilities 0.87 ( 0.22) -0.22 ( 0.44) 0.35 ( 0.30)
Netherlands Energy 1.03 ( 0.47) -0.21 ( 0.53) 0.17 ( 0.12)
Netherlands Materials 1.20 ( 0.60) -0.36 ( 0.84) 0.15 ( 0.26)
Netherlands Industrials 1.44 ( 0.58) -0.75 ( 0.92) 0.30 ( 0.37)
Netherlands Discretionary 0.80 ( 0.23) -0.22 ( 0.34) 0.42 ( 0.23)
Netherlands Staples 0.58 ( 0.21) 0.19 ( 0.20) 0.23 ( 0.17)
Netherlands Financials 0.84 ( 0.32) -0.24 ( 0.47) 0.40 ( 0.25)
Netherlands InfoTech 0.84 ( 0.35) -0.10 ( 0.40) 0.26 ( 0.16)
U.K. Energy 0.96 ( 0.44) -0.10 ( 0.48) 0.14 ( 0.11)
U.K. Materials 0.89 ( 0.41) -0.05 ( 0.45) 0.16 ( 0.20)
U.K. Industrials 1.10 ( 0.26) -0.27 ( 0.34) 0.17 ( 0.20)
U.K. Discretionary 0.90 ( 0.24) -0.27 ( 0.37) 0.37 ( 0.30)
U.K. Staples 0.50 ( 0.13) 0.17 ( 0.17) 0.33 ( 0.18)
U.K. Healthcare 0.43 ( 0.13) 0.19 ( 0.14) 0.38 ( 0.11)
U.K. Financials 0.57 ( 0.16) -0.21 ( 0.38) 0.64 ( 0.30)
U.K. InfoTech 1.27 ( 0.54) -0.49 ( 0.69) 0.22 ( 0.18)
U.K. Telecom 0.88 ( 0.21) -0.17 ( 0.25) 0.29 ( 0.16)
U.K. Utilities 0.77 ( 0.21) -0.17 ( 0.45) 0.39 ( 0.29)
Japan Energy 1.29 ( 0.58) -0.45 ( 0.82) 0.16 ( 0.26)
Japan Materials 0.98 ( 0.40) -0.03 ( 0.45) 0.05 ( 0.08)
Japan Industrials 1.13 ( 0.46) -0.29 ( 0.61) 0.15 ( 0.19)
Japan Discretionary 1.17 ( 0.52) -0.25 ( 0.60) 0.07 ( 0.10)
Japan Staples 1.11 ( 0.34) -0.15 ( 0.34) 0.04 ( 0.08)
Japan Healthcare 1.18 ( 0.59) -0.29 ( 0.78) 0.11 ( 0.22)
Japan Financials 1.32 ( 0.56) -0.42 ( 0.65) 0.11 ( 0.12)
Japan InfoTech 1.49 ( 0.78) -0.69 ( 1.01) 0.20 ( 0.25)
Japan Utilities 1.09 ( 0.30) -0.30 ( 0.50) 0.22 ( 0.24)
Canada Energy 0.66 ( 0.14) 0.23 ( 0.17) 0.11 ( 0.16)
Canada Materials 0.50 ( 0.09) 0.18 ( 0.19) 0.32 ( 0.18)
Canada Industrials 1.62 ( 0.91) -0.84 ( 1.18) 0.21 ( 0.29)
Canada Discretionary 0.87 ( 0.26) 0.01 ( 0.29) 0.13 ( 0.10)
Canada Staples 1.19 ( 0.37) -0.50 ( 0.55) 0.31 ( 0.28)
Canada Healthcare 1.11 ( 0.50) -0.78 ( 0.90) 0.67 ( 0.46)
Canada Financials 1.00 ( 0.31) -0.58 ( 0.61) 0.58 ( 0.39)
Canada InfoTech 1.10 ( 0.60) -0.32 ( 0.72) 0.22 ( 0.17)
Canada Utilities 1.26 ( 0.50) -0.46 ( 0.73) 0.20 ( 0.29)
U.S. Energy 0.79 ( 0.38) 0.08 ( 0.39) 0.13 ( 0.06)
U.S. Materials 0.60 ( 0.23) 0.11 ( 0.31) 0.29 ( 0.23)
U.S. Industrials 0.56 ( 0.23) 0.26 ( 0.16) 0.18 ( 0.12)
U.S. Discretionary 0.71 ( 0.26) 0.06 ( 0.28) 0.22 ( 0.17)
U.S. Staples 0.38 ( 0.19) 0.30 ( 0.08) 0.32 ( 0.19)
U.S. Healthcare 0.44 ( 0.17) 0.35 ( 0.10) 0.21 ( 0.10)
U.S. Financials 0.39 ( 0.13) 0.34 ( 0.09) 0.26 ( 0.13)
U.S. InfoTech 0.82 ( 0.36) 0.00 ( 0.36) 0.18 ( 0.09)
U.S. Telecom 1.08 ( 0.36) -0.22 ( 0.38) 0.13 ( 0.09)
U.S. Utilities 1.07 ( 0.63) -0.25 ( 0.80) 0.18 ( 0.22)
average for 71 country-sectors:   0.91 -0.17 0.26
Note: Figures expressed as fractional contribution to stock return variance


Table A5 (2 pages): Orthogonalized Decomposition of Cash-Flow-Revision Component (G) of Returns

Cholesky Ordering: Global, National, Sectoral, Idiosyncratic (standard errors in parentheses)

country sector global national sectoral idiosyncratic
Germany Materials 0.29 ( 0.16) 0.18 ( 0.14) 0.12 ( 0.09) 0.40 ( 0.13)
Germany Industrials 0.22 ( 0.18) 0.32 ( 0.16) 0.04 ( 0.06) 0.42 ( 0.16)
Germany Discretionary 0.11 ( 0.11) 0.12 ( 0.14) 0.01 ( 0.04) 0.76 ( 0.22)
Germany Staples 0.01 ( 0.04) 0.09 ( 0.11) 0.03 ( 0.07) 0.87 ( 0.15)
Germany Healthcare 0.16 ( 0.19) 0.01 ( 0.04) 0.25 ( 0.12) 0.58 ( 0.22)
Germany Financials 0.36 ( 0.18) 0.43 ( 0.15) 0.04 ( 0.03) 0.17 ( 0.08)
Germany InfoTech 0.05 ( 0.10) 0.34 ( 0.16) 0.07 ( 0.08) 0.54 ( 0.19)
Germany Telecom 0.18 ( 0.16) 0.21 ( 0.15) 0.38 ( 0.16) 0.23 ( 0.09)
Germany Utilities 0.10 ( 0.15) 0.02 ( 0.05) 0.05 ( 0.09) 0.83 ( 0.17)
France Energy 0.19 ( 0.23) 0.19 ( 0.14) 0.40 ( 0.16) 0.22 ( 0.09)
France Materials 0.16 ( 0.16) 0.16 ( 0.14) 0.30 ( 0.16) 0.38 ( 0.16)
France Industrials 0.14 ( 0.15) 0.39 ( 0.13) 0.00 ( 0.01) 0.47 ( 0.15)
France Discretionary 0.40 ( 0.18) 0.27 ( 0.12) 0.01 ( 0.02) 0.32 ( 0.12)
France Staples 0.15 ( 0.15) 0.08 ( 0.14) 0.24 ( 0.25) 0.53 ( 0.20)
France Healthcare 0.12 ( 0.17) 0.03 ( 0.08) 0.19 ( 0.12) 0.66 ( 0.19)
France Financials 0.24 ( 0.17) 0.27 ( 0.15) 0.20 ( 0.12) 0.29 ( 0.11)
France InfoTech 0.36 ( 0.23) 0.31 ( 0.13) 0.07 ( 0.06) 0.25 ( 0.13)
France Utilities 0.15 ( 0.18) 0.33 ( 0.14) 0.02 ( 0.04) 0.50 ( 0.18)
Italy Energy 0.02 ( 0.07) 0.11 ( 0.13) 0.08 ( 0.11) 0.80 ( 0.19)
Italy Materials 0.02 ( 0.08) 0.44 ( 0.16) 0.15 ( 0.10) 0.40 ( 0.14)
Italy Industrials 0.01 ( 0.05) 0.50 ( 0.19) 0.01 ( 0.03) 0.49 ( 0.18)
Italy Discretionary 0.30 ( 0.21) 0.40 ( 0.18) 0.04 ( 0.04) 0.26 ( 0.14)
Italy Staples 0.00 ( 0.03) 0.18 ( 0.15) 0.01 ( 0.05) 0.81 ( 0.17)
Italy Financials 0.15 ( 0.20) 0.48 ( 0.21) 0.12 ( 0.12) 0.25 ( 0.12)
Italy Telecom 0.04 ( 0.10) 0.44 ( 0.13) 0.16 ( 0.11) 0.36 ( 0.11)
Italy Utilities 0.01 ( 0.04) 0.17 ( 0.18) 0.03 ( 0.07) 0.79 ( 0.18)
Netherlands Energy 0.33 ( 0.28) 0.09 ( 0.11) 0.43 ( 0.18) 0.15 ( 0.07)
Netherlands Materials 0.36 ( 0.21) 0.16 ( 0.09) 0.00 ( 0.01) 0.49 ( 0.18)
Netherlands Industrials 0.45 ( 0.22) 0.16 ( 0.11) 0.05 ( 0.06) 0.35 ( 0.16)
Netherlands Discretionary 0.06 ( 0.13) 0.02 ( 0.05) 0.01 ( 0.03) 0.91 ( 0.16)
Netherlands Staples 0.15 ( 0.20) 0.13 ( 0.15) 0.37 ( 0.25) 0.35 ( 0.14)
Netherlands Financials 0.41 ( 0.19) 0.29 ( 0.10) 0.09 ( 0.06) 0.21 ( 0.10)
Netherlands InfoTech 0.30 ( 0.21) 0.14 ( 0.10) 0.05 ( 0.06) 0.51 ( 0.19)
U.K. Energy 0.22 ( 0.25) 0.06 ( 0.10) 0.50 ( 0.18) 0.22 ( 0.10)
U.K. Materials 0.36 ( 0.18) 0.03 ( 0.06) 0.31 ( 0.10) 0.30 ( 0.12)
U.K. Industrials 0.42 ( 0.17) 0.17 ( 0.13) 0.06 ( 0.07) 0.34 ( 0.10)
U.K. Discretionary 0.27 ( 0.18) 0.02 ( 0.05) 0.17 ( 0.12) 0.54 ( 0.16)
U.K. Staples 0.00 ( 0.02) 0.27 ( 0.12) 0.18 ( 0.11) 0.55 ( 0.17)
U.K. Healthcare 0.10 ( 0.15) 0.07 ( 0.07) 0.28 ( 0.15) 0.55 ( 0.17)
U.K. Financials 0.12 ( 0.20) 0.48 ( 0.18) 0.13 ( 0.11) 0.27 ( 0.11)
U.K. InfoTech 0.39 ( 0.17) 0.00 ( 0.03) 0.15 ( 0.09) 0.45 ( 0.17)
U.K. Telecom 0.08 ( 0.10) 0.07 ( 0.08) 0.51 ( 0.11) 0.34 ( 0.10)
U.K. Utilities 0.06 ( 0.11) 0.16 ( 0.13) 0.05 ( 0.07) 0.73 ( 0.12)
Japan Energy 0.00 ( 0.02) 0.62 ( 0.15) 0.01 ( 0.02) 0.37 ( 0.16)
Japan Materials 0.08 ( 0.15) 0.69 ( 0.18) 0.03 ( 0.03) 0.20 ( 0.08)
Japan Industrials 0.07 ( 0.15) 0.83 ( 0.17) 0.02 ( 0.02) 0.09 ( 0.04)
Japan Discretionary 0.41 ( 0.20) 0.41 ( 0.17) 0.04 ( 0.05) 0.14 ( 0.07)
Japan Staples 0.13 ( 0.16) 0.49 ( 0.15) 0.07 ( 0.06) 0.31 ( 0.13)
Japan Healthcare 0.31 ( 0.26) 0.38 ( 0.22) 0.02 ( 0.03) 0.28 ( 0.13)
Japan Financials 0.15 ( 0.20) 0.71 ( 0.19) 0.03 ( 0.03) 0.11 ( 0.05)
Japan InfoTech 0.43 ( 0.22) 0.28 ( 0.16) 0.15 ( 0.10) 0.14 ( 0.08)
Japan Utilities 0.02 ( 0.08) 0.18 ( 0.16) 0.20 ( 0.18) 0.60 ( 0.14)
Canada Energy 0.00 ( 0.03) 0.26 ( 0.17) 0.37 ( 0.16) 0.37 ( 0.11)
Canada Materials 0.05 ( 0.11) 0.05 ( 0.09) 0.35 ( 0.15) 0.54 ( 0.16)
Canada Industrials 0.42 ( 0.22) 0.14 ( 0.11) 0.15 ( 0.08) 0.29 ( 0.15)
Canada Discretionary 0.42 ( 0.18) 0.13 ( 0.12) 0.06 ( 0.05) 0.40 ( 0.14)
Canada Staples 0.12 ( 0.14) 0.06 ( 0.10) 0.06 ( 0.08) 0.76 ( 0.16)
Canada Healthcare 0.02 ( 0.09) 0.47 ( 0.20) 0.00 ( 0.01) 0.51 ( 0.19)
Canada Financials 0.09 ( 0.14) 0.19 ( 0.15) 0.25 ( 0.13) 0.47 ( 0.16)
Canada InfoTech 0.43 ( 0.21) 0.22 ( 0.11) 0.12 ( 0.09) 0.23 ( 0.12)
Canada Utilities 0.03 ( 0.07) 0.03 ( 0.08) 0.12 ( 0.09) 0.83 ( 0.15)
U.S. Energy 0.32 ( 0.27) 0.06 ( 0.06) 0.51 ( 0.21) 0.11 ( 0.06)
U.S. Materials 0.26 ( 0.21) 0.00 ( 0.02) 0.53 ( 0.17) 0.21 ( 0.09)
U.S. Industrials 0.50 ( 0.17) 0.12 ( 0.07) 0.25 ( 0.11) 0.14 ( 0.07)
U.S. Discretionary 0.60 ( 0.14) 0.13 ( 0.08) 0.13 ( 0.09) 0.13 ( 0.06)
U.S. Staples 0.04 ( 0.11) 0.08 ( 0.09) 0.67 ( 0.20) 0.22 ( 0.14)
U.S. Healthcare 0.13 ( 0.20) 0.23 ( 0.13) 0.54 ( 0.19) 0.09 ( 0.04)
U.S. Financials 0.18 ( 0.18) 0.24 ( 0.10) 0.36 ( 0.15) 0.22 ( 0.08)
U.S. InfoTech 0.52 ( 0.18) 0.08 ( 0.08) 0.36 ( 0.17) 0.05 ( 0.02)
U.S. Telecom 0.13 ( 0.17) 0.11 ( 0.11) 0.29 ( 0.17) 0.47 ( 0.19)
U.S. Utilities 0.23 ( 0.25) 0.05 ( 0.05) 0.47 ( 0.18) 0.25 ( 0.15)
Note: Figures expressed as fractional contribution to stock return variance

 

Table A6 (2 pages): Orthogonalized Decomposition of Cash-Flow-Revision Component (G) of Returns

Cholesky Ordering: Global, Sectoral, National, Idiosyncratic (standard errors in parentheses)

country sector global national sectoral idiosyncratic
Germany Materials 0.29 ( 0.16) 0.13 ( 0.11) 0.17 ( 0.12) 0.40 ( 0.13)
Germany Industrials 0.22 ( 0.18) 0.32 ( 0.15) 0.04 ( 0.09) 0.42 ( 0.16)
Germany Discretionary 0.11 ( 0.11) 0.08 ( 0.11) 0.05 ( 0.10) 0.76 ( 0.22)
Germany Staples 0.01 ( 0.04) 0.07 ( 0.10) 0.05 ( 0.08) 0.87 ( 0.15)
Germany Healthcare 0.16 ( 0.19) 0.02 ( 0.05) 0.24 ( 0.12) 0.58 ( 0.22)
Germany Financials 0.36 ( 0.18) 0.43 ( 0.15) 0.04 ( 0.07) 0.17 ( 0.08)
Germany InfoTech 0.05 ( 0.10) 0.23 ( 0.11) 0.18 ( 0.15) 0.54 ( 0.19)
Germany Telecom 0.18 ( 0.16) 0.07 ( 0.06) 0.52 ( 0.15) 0.23 ( 0.09)
Germany Utilities 0.10 ( 0.15) 0.01 ( 0.04) 0.06 ( 0.09) 0.83 ( 0.17)
France Energy 0.19 ( 0.23) 0.12 ( 0.08) 0.47 ( 0.15) 0.22 ( 0.09)
France Materials 0.16 ( 0.16) 0.09 ( 0.09) 0.36 ( 0.16) 0.38 ( 0.16)
France Industrials 0.14 ( 0.15) 0.39 ( 0.13) 0.00 ( 0.00) 0.47 ( 0.15)
France Discretionary 0.40 ( 0.18) 0.21 ( 0.10) 0.08 ( 0.07) 0.32 ( 0.12)
France Staples 0.15 ( 0.15) 0.10 ( 0.11) 0.22 ( 0.21) 0.53 ( 0.20)
France Healthcare 0.12 ( 0.17) 0.03 ( 0.06) 0.20 ( 0.13) 0.66 ( 0.19)
France Financials 0.24 ( 0.17) 0.22 ( 0.11) 0.25 ( 0.12) 0.29 ( 0.11)
France InfoTech 0.36 ( 0.23) 0.18 ( 0.09) 0.21 ( 0.12) 0.25 ( 0.13)
France Utilities 0.15 ( 0.18) 0.29 ( 0.15) 0.05 ( 0.09) 0.50 ( 0.18)
Italy Energy 0.02 ( 0.07) 0.11 ( 0.13) 0.07 ( 0.10) 0.80 ( 0.19)
Italy Materials 0.02 ( 0.08) 0.42 ( 0.17) 0.17 ( 0.17) 0.40 ( 0.14)
Italy Industrials 0.01 ( 0.05) 0.49 ( 0.19) 0.01 ( 0.05) 0.49 ( 0.18)
Italy Discretionary 0.30 ( 0.21) 0.28 ( 0.13) 0.17 ( 0.13) 0.26 ( 0.14)
Italy Staples 0.00 ( 0.03) 0.18 ( 0.15) 0.01 ( 0.04) 0.81 ( 0.17)
Italy Financials 0.15 ( 0.20) 0.42 ( 0.16) 0.18 ( 0.16) 0.25 ( 0.12)
Italy Telecom 0.04 ( 0.10) 0.26 ( 0.13) 0.34 ( 0.17) 0.36 ( 0.11)
Italy Utilities 0.01 ( 0.04) 0.12 ( 0.15) 0.08 ( 0.10) 0.79 ( 0.18)
Netherlands Energy 0.33 ( 0.28) 0.01 ( 0.01) 0.52 ( 0.22) 0.15 ( 0.07)
Netherlands Materials 0.36 ( 0.21) 0.14 ( 0.09) 0.02 ( 0.04) 0.49 ( 0.18)
Netherlands Industrials 0.45 ( 0.22) 0.09 ( 0.07) 0.11 ( 0.11) 0.35 ( 0.16)
Netherlands Discretionary 0.06 ( 0.13) 0.02 ( 0.05) 0.00 ( 0.02) 0.91 ( 0.16)
Netherlands Staples 0.15 ( 0.20) 0.05 ( 0.05) 0.45 ( 0.19) 0.35 ( 0.14)
Netherlands Financials 0.41 ( 0.19) 0.18 ( 0.10) 0.20 ( 0.11) 0.21 ( 0.10)
Netherlands InfoTech 0.30 ( 0.21) 0.15 ( 0.10) 0.04 ( 0.05) 0.51 ( 0.19)
U.K. Energy 0.22 ( 0.25) 0.04 ( 0.05) 0.52 ( 0.18) 0.22 ( 0.10)
U.K. Materials 0.36 ( 0.18) 0.01 ( 0.02) 0.34 ( 0.11) 0.30 ( 0.12)
U.K. Industrials 0.42 ( 0.17) 0.18 ( 0.12) 0.05 ( 0.07) 0.34 ( 0.10)
U.K. Discretionary 0.27 ( 0.18) 0.01 ( 0.04) 0.17 ( 0.12) 0.54 ( 0.16)
U.K. Staples 0.00 ( 0.02) 0.14 ( 0.10) 0.31 ( 0.13) 0.55 ( 0.17)
U.K. Healthcare 0.10 ( 0.15) 0.04 ( 0.04) 0.31 ( 0.16) 0.55 ( 0.17)
U.K. Financials 0.12 ( 0.20) 0.26 ( 0.12) 0.35 ( 0.18) 0.27 ( 0.11)
U.K. InfoTech 0.39 ( 0.17) 0.01 ( 0.03) 0.14 ( 0.11) 0.45 ( 0.17)
U.K. Telecom 0.08 ( 0.10) 0.07 ( 0.08) 0.51 ( 0.13) 0.34 ( 0.10)
U.K. Utilities 0.06 ( 0.11) 0.16 ( 0.14) 0.05 ( 0.08) 0.73 ( 0.12)
Japan Energy 0.00 ( 0.02) 0.49 ( 0.19) 0.14 ( 0.18) 0.37 ( 0.16)
Japan Materials 0.08 ( 0.15) 0.36 ( 0.15) 0.36 ( 0.22) 0.20 ( 0.08)
Japan Industrials 0.07 ( 0.15) 0.52 ( 0.17) 0.32 ( 0.21) 0.09 ( 0.04)
Japan Discretionary 0.41 ( 0.20) 0.42 ( 0.17) 0.03 ( 0.06) 0.14 ( 0.07)
Japan Staples 0.13 ( 0.16) 0.48 ( 0.16) 0.08 ( 0.08) 0.31 ( 0.13)
Japan Healthcare 0.31 ( 0.26) 0.40 ( 0.22) 0.01 ( 0.03) 0.28 ( 0.13)
Japan Financials 0.15 ( 0.20) 0.62 ( 0.19) 0.12 ( 0.13) 0.11 ( 0.05)
Japan InfoTech 0.43 ( 0.22) 0.23 ( 0.13) 0.20 ( 0.14) 0.14 ( 0.08)
Japan Utilities 0.02 ( 0.08) 0.21 ( 0.14) 0.18 ( 0.19) 0.60 ( 0.14)
Canada Energy 0.00 ( 0.03) 0.06 ( 0.06) 0.57 ( 0.11) 0.37 ( 0.11)
Canada Materials 0.05 ( 0.11) 0.01 ( 0.04) 0.39 ( 0.15) 0.54 ( 0.16)
Canada Industrials 0.42 ( 0.22) 0.05 ( 0.04) 0.24 ( 0.11) 0.29 ( 0.15)
Canada Discretionary 0.42 ( 0.18) 0.13 ( 0.11) 0.05 ( 0.04) 0.40 ( 0.14)
Canada Staples 0.12 ( 0.14) 0.11 ( 0.11) 0.01 ( 0.06) 0.76 ( 0.16)
Canada Healthcare 0.02 ( 0.09) 0.39 ( 0.18) 0.07 ( 0.14) 0.51 ( 0.19)
Canada Financials 0.09 ( 0.14) 0.19 ( 0.15) 0.25 ( 0.14) 0.47 ( 0.16)
Canada InfoTech 0.43 ( 0.21) 0.05 ( 0.04) 0.29 ( 0.13) 0.23 ( 0.12)
Canada Utilities 0.03 ( 0.07) 0.03 ( 0.07) 0.12 ( 0.09) 0.83 ( 0.15)
U.S. Energy 0.32 ( 0.27) 0.02 ( 0.02) 0.55 ( 0.22) 0.11 ( 0.06)
U.S. Materials 0.26 ( 0.21) 0.04 ( 0.03) 0.50 ( 0.17) 0.21 ( 0.09)
U.S. Industrials 0.50 ( 0.17) 0.09 ( 0.06) 0.27 ( 0.12) 0.14 ( 0.07)
U.S. Discretionary 0.60 ( 0.14) 0.07 ( 0.04) 0.20 ( 0.09) 0.13 ( 0.06)
U.S. Staples 0.04 ( 0.11) 0.01 ( 0.01) 0.74 ( 0.22) 0.22 ( 0.14)
U.S. Healthcare 0.13 ( 0.20) 0.03 ( 0.02) 0.75 ( 0.19) 0.09 ( 0.04)
U.S. Financials 0.18 ( 0.18) 0.20 ( 0.09) 0.40 ( 0.14) 0.22 ( 0.08)
U.S. InfoTech 0.52 ( 0.18) 0.01 ( 0.01) 0.43 ( 0.17) 0.05 ( 0.02)
U.S. Telecom 0.13 ( 0.17) 0.12 ( 0.10) 0.27 ( 0.17) 0.47 ( 0.19)
U.S. Utilities 0.23 ( 0.25) 0.03 ( 0.04) 0.48 ( 0.17) 0.25 ( 0.15)
Note: Figures expressed as fractional contribution to stock return variance

 

Table A7 (2 pages): Orthogonalized Decomposition of Discount-Rate Component (H) of Returns

Cholesky Ordering: Global, National, Sectoral, Idiosyncratic (standard errors in parentheses)

country sector global national sectoral idiosyncratic
Germany Materials 0.80 ( 0.18) 0.02 ( 0.07) 0.07 ( 0.11) 0.10 ( 0.10)
Germany Industrials 0.67 ( 0.46) 0.08 ( 0.22) 0.08 ( 0.22) 0.17 ( 0.31)
Germany Discretionary 0.84 ( 0.14) 0.02 ( 0.10) 0.01 ( 0.03) 0.13 ( 0.15)
Germany Staples 0.32 ( 0.45) 0.01 ( 0.11) 0.00 ( 0.06) 0.66 ( 0.42)
Germany Healthcare 0.40 ( 0.47) 0.28 ( 0.44) 0.01 ( 0.06) 0.31 ( 0.29)
Germany Financials 0.59 ( 0.46) 0.33 ( 0.44) 0.00 ( 0.02) 0.08 ( 0.10)
Germany InfoTech 0.63 ( 0.51) 0.29 ( 0.45) 0.00 ( 0.01) 0.08 ( 0.10)
Germany Telecom 0.85 ( 0.20) 0.04 ( 0.15) 0.05 ( 0.12) 0.06 ( 0.08)
Germany Utilities 0.60 ( 0.34) 0.21 ( 0.30) 0.14 ( 0.23) 0.04 ( 0.06)
France Energy 0.17 ( 0.51) 0.34 ( 0.60) 0.18 ( 0.54) 0.30 ( 0.25)
France Materials 0.43 ( 0.63) 0.14 ( 0.40) 0.23 ( 0.34) 0.20 ( 0.46)
France Industrials 0.72 ( 0.49) 0.10 ( 0.38) 0.06 ( 0.14) 0.11 ( 0.17)
France Discretionary 0.80 ( 0.16) 0.04 ( 0.12) 0.09 ( 0.08) 0.07 ( 0.08)
France Staples 0.81 ( 0.38) 0.15 ( 0.35) 0.00 ( 0.04) 0.04 ( 0.06)
France Healthcare 0.36 ( 0.62) 0.31 ( 0.53) 0.09 ( 0.28) 0.25 ( 0.29)
France Financials 0.60 ( 0.45) 0.04 ( 0.21) 0.30 ( 0.35) 0.07 ( 0.10)
France InfoTech 0.24 ( 0.45) 0.63 ( 0.50) 0.06 ( 0.12) 0.07 ( 0.13)
France Utilities 0.05 ( 0.22) 0.69 ( 0.39) 0.01 ( 0.11) 0.24 ( 0.22)
Italy Energy 0.31 ( 0.46) 0.02 ( 0.25) 0.24 ( 0.51) 0.42 ( 0.58)
Italy Materials 0.02 ( 0.38) 0.23 ( 0.98) 0.40 ( 1.31) 0.35 ( 0.50)
Italy Industrials 0.38 ( 0.42) 0.43 ( 0.53) 0.07 ( 0.21) 0.12 ( 0.41)
Italy Discretionary 0.38 ( 0.52) 0.31 ( 0.53) 0.07 ( 0.18) 0.24 ( 0.42)
Italy Staples 0.73 ( 0.37) 0.01 ( 0.11) 0.16 ( 0.31) 0.11 ( 0.19)
Italy Financials 0.61 ( 0.49) 0.13 ( 0.54) 0.09 ( 0.39) 0.18 ( 0.23)
Italy Telecom 0.59 ( 0.39) 0.33 ( 0.37) 0.03 ( 0.06) 0.06 ( 0.10)
Italy Utilities 0.51 ( 0.43) 0.13 ( 0.42) 0.00 ( 0.02) 0.36 ( 0.46)
Netherlands Energy 0.28 ( 0.55) 0.43 ( 0.44) 0.18 ( 0.23) 0.11 ( 0.09)
Netherlands Materials 0.03 ( 0.20) 0.80 ( 0.29) 0.03 ( 0.20) 0.13 ( 0.26)
Netherlands Industrials 0.06 ( 0.22) 0.60 ( 0.36) 0.09 ( 0.19) 0.26 ( 0.39)
Netherlands Discretionary 0.58 ( 0.36) 0.06 ( 0.21) 0.01 ( 0.06) 0.35 ( 0.36)
Netherlands Staples 0.58 ( 0.49) 0.24 ( 0.44) 0.06 ( 0.18) 0.12 ( 0.11)
Netherlands Financials 0.85 ( 0.21) 0.11 ( 0.21) 0.01 ( 0.03) 0.03 ( 0.03)
Netherlands InfoTech 0.78 ( 0.30) 0.10 ( 0.25) 0.00 ( 0.01) 0.12 ( 0.15)
U.K. Energy 0.42 ( 0.62) 0.38 ( 0.62) 0.10 ( 0.21) 0.10 ( 0.11)
U.K. Materials 0.14 ( 0.43) 0.03 ( 0.17) 0.80 ( 0.39) 0.03 ( 0.06)
U.K. Industrials 0.84 ( 0.24) 0.06 ( 0.11) 0.02 ( 0.10) 0.09 ( 0.16)
U.K. Discretionary 0.85 ( 0.17) 0.00 ( 0.02) 0.08 ( 0.11) 0.07 ( 0.09)
U.K. Staples 0.27 ( 0.44) 0.00 ( 0.04) 0.60 ( 0.53) 0.13 ( 0.24)
U.K. Healthcare 0.64 ( 0.38) 0.20 ( 0.22) 0.07 ( 0.21) 0.09 ( 0.06)
U.K. Financials 0.82 ( 0.28) 0.07 ( 0.18) 0.06 ( 0.07) 0.05 ( 0.07)
U.K. InfoTech 0.28 ( 0.62) 0.07 ( 0.35) 0.49 ( 0.71) 0.15 ( 0.22)
U.K. Telecom 0.71 ( 0.35) 0.05 ( 0.11) 0.01 ( 0.10) 0.23 ( 0.24)
U.K. Utilities 0.15 ( 0.33) 0.22 ( 0.61) 0.56 ( 0.45) 0.08 ( 0.11)
Japan Energy 0.02 ( 0.26) 0.04 ( 0.30) 0.17 ( 0.93) 0.78 ( 0.79)
Japan Materials 0.12 ( 0.62) 0.09 ( 0.47) 0.28 ( 0.67) 0.52 ( 0.84)
Japan Industrials 0.09 ( 0.47) 0.77 ( 0.52) 0.00 ( 0.01) 0.14 ( 0.20)
Japan Discretionary 0.24 ( 0.92) 0.56 ( 0.91) 0.04 ( 0.16) 0.16 ( 0.24)
Japan Staples 0.13 ( 0.67) 0.10 ( 0.74) 0.17 ( 0.59) 0.60 ( 0.65)
Japan Healthcare 0.04 ( 0.33) 0.38 ( 1.00) 0.01 ( 0.13) 0.56 ( 1.03)
Japan Financials 0.11 ( 0.49) 0.72 ( 0.61) 0.00 ( 0.03) 0.17 ( 0.29)
Japan InfoTech 0.05 ( 0.28) 0.79 ( 0.52) 0.08 ( 0.34) 0.08 ( 0.17)
Japan Utilities 0.00 ( 0.05) 0.01 ( 0.23) 0.33 ( 0.47) 0.65 ( 0.47)
Canada Energy 0.03 ( 0.29) 0.15 ( 0.34) 0.16 ( 0.49) 0.66 ( 0.48)
Canada Materials 0.03 ( 0.16) 0.14 ( 0.28) 0.73 ( 0.36) 0.10 ( 0.21)
Canada Industrials 0.22 ( 0.48) 0.49 ( 0.43) 0.02 ( 0.10) 0.28 ( 0.45)
Canada Discretionary 0.41 ( 0.51) 0.38 ( 0.39) 0.01 ( 0.03) 0.20 ( 0.22)
Canada Staples 0.01 ( 0.10) 0.68 ( 0.34) 0.18 ( 0.33) 0.13 ( 0.12)
Canada Healthcare 0.01 ( 0.15) 0.66 ( 0.31) 0.04 ( 0.15) 0.28 ( 0.24)
Canada Financials 0.39 ( 0.36) 0.26 ( 0.28) 0.24 ( 0.23) 0.11 ( 0.10)
Canada InfoTech 0.43 ( 0.43) 0.50 ( 0.43) 0.01 ( 0.05) 0.05 ( 0.07)
Canada Utilities 0.10 ( 0.31) 0.07 ( 0.28) 0.08 ( 0.34) 0.75 ( 0.56)
U.S. Energy 0.03 ( 0.23) 0.56 ( 0.28) 0.30 ( 0.39) 0.11 ( 0.29)
U.S. Materials 0.07 ( 0.27) 0.27 ( 0.41) 0.46 ( 0.33) 0.19 ( 0.14)
U.S. Industrials 0.66 ( 0.35) 0.19 ( 0.31) 0.03 ( 0.16) 0.12 ( 0.13)
U.S. Discretionary 0.45 ( 0.34) 0.05 ( 0.13) 0.44 ( 0.27) 0.06 ( 0.07)
U.S. Staples 0.36 ( 0.43) 0.06 ( 0.16) 0.56 ( 0.41) 0.03 ( 0.05)
U.S. Healthcare 0.54 ( 0.47) 0.13 ( 0.24) 0.30 ( 0.33) 0.03 ( 0.02)
U.S. Financials 0.66 ( 0.27) 0.20 ( 0.22) 0.08 ( 0.15) 0.06 ( 0.08)
U.S. InfoTech 0.69 ( 0.32) 0.14 ( 0.17) 0.14 ( 0.24) 0.03 ( 0.05)
U.S. Telecom 0.63 ( 0.40) 0.21 ( 0.26) 0.01 ( 0.12) 0.15 ( 0.30)
U.S. Utilities 0.02 ( 0.18) 0.10 ( 0.33) 0.64 ( 0.41) 0.23 ( 0.32)

Note: Figures expressed as fractional contribution to stock return variance


Table A8 (2 pages): Orthogonalized Decomposition of Discount-Rate Component (H) of Returns

Cholesky Ordering: Global, Sectoral, National, Idiosyncratic (standard errors in parentheses)

country sector global national sectoral idiosyncratic
Germany Materials 0.80 ( 0.18) 0.03 ( 0.08) 0.06 ( 0.11) 0.10 ( 0.10)
Germany Industrials 0.67 ( 0.46) 0.03 ( 0.21) 0.13 ( 0.26) 0.17 ( 0.31)
Germany Discretionary 0.84 ( 0.14) 0.02 ( 0.10) 0.00 ( 0.02) 0.13 ( 0.15)
Germany Staples 0.32 ( 0.45) 0.01 ( 0.11) 0.00 ( 0.03) 0.66 ( 0.42)
Germany Healthcare 0.40 ( 0.47) 0.28 ( 0.45) 0.01 ( 0.08) 0.31 ( 0.29)
Germany Financials 0.59 ( 0.46) 0.32 ( 0.44) 0.01 ( 0.05) 0.08 ( 0.10)
Germany InfoTech 0.63 ( 0.51) 0.08 ( 0.18) 0.21 ( 0.41) 0.08 ( 0.10)
Germany Telecom 0.85 ( 0.20) 0.00 ( 0.03) 0.09 ( 0.18) 0.06 ( 0.08)
Germany Utilities 0.60 ( 0.34) 0.00 ( 0.03) 0.35 ( 0.33) 0.04 ( 0.06)
France Energy 0.17 ( 0.51) 0.27 ( 0.46) 0.26 ( 0.53) 0.30 ( 0.25)
France Materials 0.43 ( 0.63) 0.01 ( 0.06) 0.36 ( 0.44) 0.20 ( 0.46)
France Industrials 0.72 ( 0.49) 0.10 ( 0.37) 0.07 ( 0.19) 0.11 ( 0.17)
France Discretionary 0.80 ( 0.16) 0.08 ( 0.15) 0.05 ( 0.08) 0.07 ( 0.08)
France Staples 0.81 ( 0.38) 0.13 ( 0.35) 0.02 ( 0.12) 0.04 ( 0.06)
France Healthcare 0.36 ( 0.62) 0.35 ( 0.54) 0.04 ( 0.21) 0.25 ( 0.29)
France Financials 0.60 ( 0.45) 0.01 ( 0.09) 0.32 ( 0.40) 0.07 ( 0.10)
France InfoTech 0.24 ( 0.45) 0.12 ( 0.23) 0.57 ( 0.57) 0.07 ( 0.13)
France Utilities 0.05 ( 0.22) 0.27 ( 0.55) 0.44 ( 0.46) 0.24 ( 0.22)
Italy Energy 0.31 ( 0.46) 0.00 ( 0.09) 0.26 ( 0.51) 0.42 ( 0.58)
Italy Materials 0.02 ( 0.38) 0.00 ( 0.02) 0.63 ( 0.71) 0.35 ( 0.50)
Italy Industrials 0.38 ( 0.42) 0.43 ( 0.74) 0.07 ( 0.22) 0.12 ( 0.41)
Italy Discretionary 0.38 ( 0.52) 0.35 ( 0.53) 0.03 ( 0.15) 0.24 ( 0.42)
Italy Staples 0.73 ( 0.37) 0.05 ( 0.17) 0.11 ( 0.35) 0.11 ( 0.19)
Italy Financials 0.61 ( 0.49) 0.00 ( 0.06) 0.21 ( 0.32) 0.18 ( 0.23)
Italy Telecom 0.59 ( 0.39) 0.21 ( 0.29) 0.15 ( 0.38) 0.06 ( 0.10)
Italy Utilities 0.51 ( 0.43) 0.10 ( 0.40) 0.04 ( 0.19) 0.36 ( 0.46)
Netherlands Energy 0.28 ( 0.55) 0.02 ( 0.06) 0.59 ( 0.53) 0.11 ( 0.09)
Netherlands Materials 0.03 ( 0.20) 0.53 ( 0.38) 0.31 ( 0.55) 0.13 ( 0.26)
Netherlands Industrials 0.06 ( 0.22) 0.66 ( 0.42) 0.02 ( 0.18) 0.26 ( 0.39)
Netherlands Discretionary 0.58 ( 0.36) 0.06 ( 0.19) 0.02 ( 0.07) 0.35 ( 0.36)
Netherlands Staples 0.58 ( 0.49) 0.11 ( 0.23) 0.18 ( 0.41) 0.12 ( 0.11)
Netherlands Financials 0.85 ( 0.21) 0.09 ( 0.20) 0.03 ( 0.07) 0.03 ( 0.03)
Netherlands InfoTech 0.78 ( 0.30) 0.06 ( 0.18) 0.04 ( 0.16) 0.12 ( 0.15)
U.K. Energy 0.42 ( 0.62) 0.04 ( 0.11) 0.44 ( 0.56) 0.10 ( 0.11)
U.K. Materials 0.14 ( 0.43) 0.00 ( 0.00) 0.83 ( 0.42) 0.03 ( 0.06)
U.K. Industrials 0.84 ( 0.24) 0.02 ( 0.07) 0.06 ( 0.16) 0.09 ( 0.16)
U.K. Discretionary 0.85 ( 0.17) 0.01 ( 0.04) 0.07 ( 0.11) 0.07 ( 0.09)
U.K. Staples 0.27 ( 0.44) 0.00 ( 0.01) 0.60 ( 0.57) 0.13 ( 0.24)
U.K. Healthcare 0.64 ( 0.38) 0.04 ( 0.07) 0.23 ( 0.40) 0.09 ( 0.06)
U.K. Financials 0.82 ( 0.28) 0.04 ( 0.07) 0.09 ( 0.18) 0.05 ( 0.07)
U.K. InfoTech 0.28 ( 0.62) 0.01 ( 0.04) 0.56 ( 0.57) 0.15 ( 0.22)
U.K. Telecom 0.71 ( 0.35) 0.02 ( 0.10) 0.04 ( 0.20) 0.23 ( 0.24)
U.K. Utilities 0.15 ( 0.33) 0.01 ( 0.04) 0.77 ( 0.32) 0.08 ( 0.11)
Japan Energy 0.02 ( 0.26) 0.00 ( 0.12) 0.20 ( 0.79) 0.78 ( 0.79)
Japan Materials 0.12 ( 0.62) 0.14 ( 0.51) 0.23 ( 0.63) 0.52 ( 0.84)
Japan Industrials 0.09 ( 0.47) 0.40 ( 0.44) 0.37 ( 0.54) 0.14 ( 0.20)
Japan Discretionary 0.24 ( 0.92) 0.47 ( 1.21) 0.14 ( 0.59) 0.16 ( 0.24)
Japan Staples 0.13 ( 0.67) 0.20 ( 0.91) 0.07 ( 0.63) 0.60 ( 0.65)
Japan Healthcare 0.04 ( 0.33) 0.36 ( 0.76) 0.03 ( 0.40) 0.56 ( 1.03)
Japan Financials 0.11 ( 0.49) 0.66 ( 0.83) 0.07 ( 0.42) 0.17 ( 0.29)
Japan InfoTech 0.05 ( 0.28) 0.16 ( 0.33) 0.71 ( 0.50) 0.08 ( 0.17)
Japan Utilities 0.00 ( 0.05) 0.09 ( 0.36) 0.26 ( 0.57) 0.65 ( 0.47)
Canada Energy 0.03 ( 0.29) 0.09 ( 0.28) 0.22 ( 0.57) 0.66 ( 0.48)
Canada Materials 0.03 ( 0.16) 0.05 ( 0.12) 0.82 ( 0.24) 0.10 ( 0.21)
Canada Industrials 0.22 ( 0.48) 0.49 ( 0.44) 0.01 ( 0.11) 0.28 ( 0.45)
Canada Discretionary 0.41 ( 0.51) 0.36 ( 0.40) 0.03 ( 0.12) 0.20 ( 0.22)
Canada Staples 0.01 ( 0.10) 0.18 ( 0.48) 0.68 ( 0.51) 0.13 ( 0.12)
Canada Healthcare 0.01 ( 0.15) 0.54 ( 0.37) 0.17 ( 0.35) 0.28 ( 0.24)
Canada Financials 0.39 ( 0.36) 0.00 ( 0.03) 0.50 ( 0.32) 0.11 ( 0.10)
Canada InfoTech 0.43 ( 0.43) 0.27 ( 0.23) 0.24 ( 0.42) 0.05 ( 0.07)
Canada Utilities 0.10 ( 0.31) 0.13 ( 0.36) 0.03 ( 0.21) 0.75 ( 0.56)
U.S. Energy 0.03 ( 0.23) 0.01 ( 0.13) 0.85 ( 0.26) 0.11 ( 0.29)
U.S. Materials 0.07 ( 0.27) 0.04 ( 0.07) 0.69 ( 0.21) 0.19 ( 0.14)
U.S. Industrials 0.66 ( 0.35) 0.04 ( 0.11) 0.18 ( 0.23) 0.12 ( 0.13)
U.S. Discretionary 0.45 ( 0.34) 0.01 ( 0.03) 0.48 ( 0.31) 0.06 ( 0.07)
U.S. Staples 0.36 ( 0.43) 0.00 ( 0.00) 0.61 ( 0.42) 0.03 ( 0.05)
U.S. Healthcare 0.54 ( 0.47) 0.00 ( 0.00) 0.43 ( 0.47) 0.03 ( 0.02)
U.S. Financials 0.66 ( 0.27) 0.02 ( 0.05) 0.26 ( 0.22) 0.06 ( 0.08)
U.S. InfoTech 0.69 ( 0.32) 0.01 ( 0.03) 0.27 ( 0.31) 0.03 ( 0.05)
U.S. Telecom 0.63 ( 0.40) 0.15 ( 0.41) 0.07 ( 0.26) 0.15 ( 0.30)
U.S. Utilities 0.02 ( 0.18) 0.02 ( 0.08) 0.72 ( 0.33) 0.23 ( 0.32)

Note: Figures expressed as fractional contribution to stock return variance

Footnotes

1   Tests for the number of factors in an APT model typically reject a single factor specification in favor of a multiple factor alternative, but usually a single factor can explain most of the common variations. More to the point, a statistically significant risk premium is often obtained for only one factor (for example, see Connor and Korajczyk (1988)). Even in a single factor model, if betas are time-varying, the conditional mean returns of two assets need not be perfectly correlated over time. However, Ferson and Harvey (1991) found that time variation in factor risk premiums accounted for more of the variation in conditional mean returns than did time variation in factor loadings. Return to text
2   We get very similar results when returns are measured in local currency and we would expect to also get similar results for excess dollar returns over the short dollar interest rate. Campbell and Ammer (1993) find that variation in both realized and expected future short-term interest rates are dwarfed by stock return volatility. Return to text
3   The GMM regression coefficients for lags of the state vector are identical to OLS. However, GMM produces standard errors for the estimated covariance matrix of the residuals, which is important for our application. Return to text
4   The results here are not sensitive to our choice of 0.95 for the log-linearization parameter ($ \rho)$, which corresponds to a Taylor approximation around a long-term dividend-price ratio of about 5 percent. Results reported in Tables 8 and A4 are, however, sensitive to ($ \rho)$. Return to text

This version is optimized for use by screen readers. A printable pdf version is available.

Home | Economic research and data | Publications and education resources
Accessibility | Contact us
Last update: October 24, 2006