The Federal Reserve Board eagle logo links to home page

Growth-Led Exports: Is Variety the Spice of Trade?

Joseph E. Gagnon1

NOTE: International Finance Discussion Papers are preliminary materials circulated to stimulate discussion and critical comment. References in publications to International Finance Discussion Papers (other than an acknowledgment that the writer has had access to unpublished material) should be cleared with the author or authors. Recent IFDPs are available on the Web at http://www.federalreserve.gov/pubs/ifdp/. This paper can be downloaded without charge from the Social Science Research Network electronic library at http://www.ssrn.com/.

Abstract:

Fast-growing countries tend to experience rapid export growth with little secular change in their terms of trade. This contradicts the standard Armington trade model, which predicts that fast-growing countries can experience rapid export growth only to the extent that they accept declining terms of trade. This paper generalizes the monopolistic competition trade model of Helpman and Krugman (1985), providing a basis for growth-led exports without declining terms of trade. The key mechanism behind this result is that fast-growing countries are able to develop new varieties of products that can be exported without pushing down the prices of existing products. There is strong support for the new model in long-run export growth of many countries in the post-war era.

Keywords: export demand, international trade, product differentiation

JEL Classification: F1, F4

I  Introduction

Few people would be surprised to learn that there is a strong positive correlation between the growth rate of a country's exports and the growth rate of its economy. Indeed, there is an extensive body of theoretical and empirical research on the phenomenon of ``export-led growth,'' which focuses on the benefits for long-run economic growth of encouraging exports and openness to trade.2 Curiously, however, the standard empirical model of trade flows implies that fast-growing countries with fast-growing exports should be experiencing secular declines in their terms of trade. But there is little evidence for such behavior in the terms of trade.

Figure 1 shows the positive correlation between long-run export growth and long-run economic growth in a sample of 64 countries over the period 1960-2000.3 Figure 2 shows essentially no correlation between changes in the terms of trade and long-run economic growth for these countries. Regression analysis presented in this paper demonstrates that this lack of correlation cannot be attributed to simultaneity bias and is unlikely to reflect omitted factors.

To explain these empirical findings, this paper develops a new model of export demand based on the theoretical work of Helpman and Krugman (1985). The new model significantly and robustly outperforms the standard model. Unlike the standard assumption of one good per country, the alternative model allows for multiple varieties of goods to be produced in each country. In this model, economic growth allows a country to produce more varieties, and demand for a country's exports is directly tied to the number of varieties it produces. Thus, fast-growing countries can have fast-growing exports without a decline in the terms of trade.

This finding carries important implications for empirical international macroeconomics. In most models of international macroeconomic linkages, permanently higher output tends to lower a country's trade balance through higher imports that are not matched by higher exports, at least not without a permanent decline in the terms of trade. For example, in the Fall 2004 Per Jacobsson Lecture, former Treasury Secretary Lawrence Summers claimed that the sustained increase in U.S. economic growth since the mid-1990s was at least partly responsible for the widening of the U.S. trade deficit.4 This research questions that conclusion.

The ``growth-led exports'' view of this paper is complementary to the traditional view of export-led growth. Deregulating, opening up the economy, and otherwise encouraging exports may indeed spur growth through technological transfer and more competitive producers. The model developed here helps to explain why such growth is all the more beneficial for a country's welfare because it is not offset by declining terms of trade. The evidence presented in this paper provides some support for a connection between changes in openness to foreign trade and economic growth. But even for countries with a relatively stable share of exports in GDP, faster economic growth tends to be associated with faster export growth.

The next section of the paper demonstrates that there is no significant link between long-run rates of economic growth and the terms of trade; in particular, exogenous forces driving growth do not have significantly adverse implications for a country's terms of trade. Section III develops a theoretical model to explain this empirical regularity. Section IV estimates the model and explores the robustness of the key parameters. Section V is a brief conclusion.

II  Terms of Trade and Economic Growth

Figures 1 and 2 display a strong link between export growth and economic growth in the long run and essentially no link between changes in the terms of trade and long-run economic growth. The latter finding is not consistent with the standard Armington (1969) model of export supply and demand under the assumption that economic growth is exogenous with respect to the terms of trade. As shown in Figure 3, faster economic growth shifts out the export supply curve and the economy moves down the export demand curve from point A to a new lower price of exports at point B.5With exogenous economic growth, export demand shocks may add noise to the empirical relationship, but they should not bias the coefficient in a regression of the terms of trade on economic growth. However, if long-run economic growth is not exogenous with respect to the terms of trade, then the (negative) coefficient is biased upward because positive demand shocks will tend to raise both the terms of trade and economic growth. This is conventional simultaneity bias.

It may be plausible to maintain that a country's long-run economic growth is determined by factors that are exogenous to the terms of trade, such as population growth and institutional characteristics that encourage or discourage the accumulation of human and physical capital. Nevertheless, the finding of no long-run correlation between changes in the terms of trade and economic growth is robust to the use of instrumental variables to isolate factors behind economic growth that clearly are exogenous with respect to changes in a country's terms of trade.

Table 1 presents cross-country instrumental-variables regressions of long-run changes in the terms of trade on long-run economic growth rates and other variables. (Data are described in the Data Appendix.) Following a recent paper by Acemoglu and Ventura (2002), long-run economic growth is instrumented by the levels of three variables that are observed at the beginning of the sample: real per capita income adjusted for purchasing power parity (PPP), the average years of schooling of the labor force, and the average life expectancy. All of these instruments are predetermined and thus exogenous with respect to subsequent changes in the terms of trade. Because the focus here is on total economic growth rather than per capita growth (as in Acemoglu and Ventura) population growth is added as a fourth instrument, under the assumption that population growth is exogenous to the terms of trade. However, the results are not sensitive to dropping the population growth rate.

Acemoglu and Ventura argue that the human capital variables (years of schooling and life expectancy) may have independent effects on the terms of trade, so they are included in the second-stage regression here, though the results are not sensitive to excluding them. The regressions also include a dummy variable for countries that produced more than twice as much oil as they consumed in 1985.6 From the point of view of many oil exporting countries, changes in the price of oil represent major exogenous shocks to the terms of trade that may have had lasting effects on economic growth.

Column (1) of Table 1 presents results of a regression of the change in the terms of trade between 1960 and 2000 on the growth of real GDP over the same period and on the oil exporter dummy. Neither coefficient is significant and the equation R$ ^{2}$ is very low, despite a respectable fit of the first-stage regression. Column (2) adds the human capital variables, which are statistically significant, though it is difficult to understand why schooling should have a negative effect on the terms of trade and life expectancy should have a positive effect.

Columns (3) and (4) break the sample into two 20-year sub-periods. The human capital variables are not significant in either sub-period. However, the oil dummy is significantly positive in the first sub-period, when oil prices were rising, and negative in the second period, when oil prices were falling in real terms. In neither sub-period is there a significant coefficient on GDP growth.

Column (5) replaces total GDP growth with per capita GDP growth and drops population growth from the instruments. This is the specification in Acemoglu and Ventura.7 Here the results are almost identical to those for total GDP growth in column (2). Column (6) focuses on countries whose exports are primarily composed of manufactured goods and services, in order to minimize the effect of volatile commodity prices on the regression.8 Column (7) restricts the sample to industrial countries, for which the data quality is generally highest. In neither column (6) nor column (7) is there a significant coefficient on GDP growth.

Altogether, the results shown in Table 1 are consistent with little or no effect of long-run economic growth on a country's terms of trade.

III  Theoretical Model

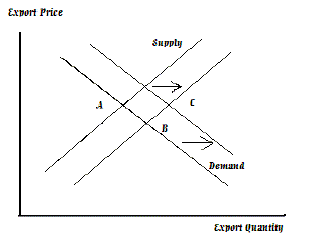
This section derives a two-country model of export demand and supply based on tastes, technology, and labor in a setting with endogenous varieties of goods.9 Under plausible assumptions, the number of varieties grows in proportion to a country's total output.10 A key contribution of this paper is to show that allowing for endogenous varieties leads to an export demand equation that can be approximated by augmenting the standard Armington demand equation with a term for the relative size of the exporting country in the world economy.11 In this model, as shown in Figure 4, long-run economic growth in output shifts both the export supply and export demand curves out simultaneously, moving from point A to point C with minimal effect on the price of exports.

Demand

The demand side of the model is taken from Helpman and Krugman (1985) who, in turn, based their work on the ``love of variety'' utility function proposed by Dixit and Stiglitz (1977).12 The utility of the representative household is displayed in equation (1). The budget constraint is equation (2). Here D represents domestic consumption of domestically produced goods and X* represents imports (exports from the rest of the world). Asterisks denote foreign variables. The subscripts denote individual varieties. There are N domestic varieties and N* varieties of imports. Prices of domestic goods are denoted by P$ ^{D}$. Import prices (in foreign currency) are denoted by P$ ^{X}$* and the exchange rate is R. Total expenditure is E. ``A'' is an exogenous variable that reflects taste for imports. Consumers are biased towards domestic goods if A is less than unity. The elasticity of substitution, $ \Sigma$, is assumed to be equal across all goods in order to obtain a closed-form solution for demand.

\begin{displaymath} \begin{array}[c]{l} (1)\,\,U\,=\,\left[ {\sum\limits_{i=1}^{... ...s_{i=1} ^{N\ast} {R\,P_{i}^{X\ast} \,X\ast_{i} }\ \end{array}\end{displaymath}

The representative household chooses consumption of each variety to maximize (1) subject to (2) and taking prices, available varieties, and total expenditure as given.13 All domestic firms face the same production technology, which leads to equal prices of all domestic varieties, P$ ^{D}$, and thus equal quantities sold, D. Similarly, all foreign varieties sell at the common price P$ ^{X}$* with equal quantities X*. Aggregate demand for each type of good equals the number of varieties times the quantity demanded of each variety. The resulting aggregate demand system is given by equations (3)-(4). As discussed in Anderson and van Wincoop (2003), the share of spending on domestic goods equals 1/(1+A) and the share spent on foreign goods is A/(1+A).

\begin{displaymath} \begin{array}[c]{l} (3)\,\,N\,D\,\,=\,\,\frac{N\,\left( {P^{... ...ce}\!\lower0.7ex\hbox{$A$}} \right) ^{1-\sigma}}\ \end{array}\end{displaymath}
Solving the analogous system for the rest of the world, yields equations (5)-(6).14

\begin{displaymath} \begin{array}[c]{l} (5)\,\,N\ast\,D\ast\,\,=\,\,\frac{N\ast\... ...ex\hbox{${R\,A\ast }$}} \right) ^{1-\sigma\ast}}\ \end{array}\end{displaymath}

Expenditure equals revenue from domestic production plus an exogenous transfer, T, from the rest of the world: equation (7). Foreign expenditure equals foreign production minus the transfer converted into foreign currency: equation (8). The transfer allows for unbalanced trade. T is assumed to be driven by macroeconomic factors such as fiscal and monetary policy that affect national saving and investment.

\begin{displaymath} \begin{array}[c]{l} (7)\,\,E\,=\,N\,\left( {P^{D}\,D\,+\,P^{... ...kern-\nulldelimiterspace}\!\lower0.7ex\hbox{$R$}\ \end{array}\end{displaymath}

Supply

Now turn to the firms' decisions and aggregate supply. There are a potentially unlimited number of varieties within each class of good, but a firm must pay a fixed cost for each new variety as well as a marginal cost for each unit of output. All costs and prices are expressed in terms of units of labor. Equations (9) and (10) are the total cost functions for each variety of domestic and foreign good, respectively.15 Note that each variety is both consumed at home (D) and exported (X). F is the fixed cost and G is the marginal cost. Technological progress tends to lower costs, and can thus be modeled as an exogenous decline in F and G.

\begin{displaymath} \begin{array}[c]{l} (9)\,\,C\,\,=\,\,F\,+\,G\,\left( {D\,+\,... ...ast\,+\,G\ast\,\left( {D\ast\,+\,X\ast} \right) \ \end{array}\end{displaymath}

The profit-maximizing prices depend on the elasticity of substitution and the marginal cost, as shown in equations (11)-(14).16 These are standard markup equations.

(11) $ P^{D}=(\frac{\sigma}{\sigma-1})G$

(12) $ P^{X}=P^{D}$

(13) $ P^{D^{\ast}}=(\frac{\sigma}{\sigma-1})G^{\ast}$

(14) $ P^{X^{\ast}}=P^{D^{\ast}}$

Total production in each country exhausts the available pool of labor, shown in equations (15)-(16), thereby determining the number of varieties of goods produced. Aggregate labor supply, L, is exogenous in each region. Free entry ensures that firm profits are zero, driving revenue equal to cost for each variety: equations (17)-(18). By Walras' Law, one of the last two equations or one of the two expenditure equations can be dropped.

\begin{displaymath} \begin{array}[c]{l} (15)\,\,L\,\,=\,\,N\,\left[ {F\,+\,G\,\l... ...\,F\ast\,+\,G\ast\,\left( {D\ast+X\ast} \right) \ \end{array}\end{displaymath}

Implications for Empirical Export Demand

This sub-section derives an estimable version of equation (6) for aggregate exports. The first step is to substitute the (unobserved) number of varieties produced by a country with the country's (observed) total output. Total output is defined as the number of varieties produced times the quantity of each variety, shown in equation (19). Inserting equations (11) and (12) into (17) yields equation (20) for domestic output of each variety. Substituting (20) into (19) and rearranging terms shows that the number of varieties is a function of total output and the ratio of marginal to fixed cost, equation (21).

\begin{displaymath} \begin{array}[c]{l} (19)\,\,Y\,\,=\,\,N\,\left( {D\,+\,X} \r... ...\ (21)\,\,N\,\,=\,\,\frac{Y\,G}{(\sigma-1)\,F}\ \end{array}\end{displaymath} The second step is to define the foreign expenditure price as the weighted average of foreign and domestic prices, shown in equation (22). Inserting (21) into (6), dividing the numerator and denominator by P$ ^{E}$*, and making use of (22) yields equation (23), where Z=G/[($ \Sigma$-1)F] for notational simplicity.

(22) $ P^{E^{\ast}}=(\frac{P^{D^{\ast}}D^{\ast}}{E^{\ast}})P^{D^{\ast} }+(\frac{P^{X}X}{RE^{\ast}})\frac{P^{X}}{R}$

(23) $ NX=\left( \frac{P^{X}}{RP^{E^{\ast}}}\right) ^{-\sigma}\left( \frac{E^{\ast}}{... ...st}}\left[ \frac{P^{X}}{RP^{D^{\ast}}A^{\ast}}\right] ^{1-\sigma }\right) ^{-1}$

To obtain a linear equation in growth rates, take the logarithm of equation (23) and totally differentiate. An appendix (available upon request) shows that the change in log exports can be expressed in terms of the log changes in other variables as shown in equation (24). The simple form of equation (24) derives from the assumed initial conditions that technology is the same across the two countries (F=F* and G=G*) and there is no home bias (A*=1). Equation (24) can be viewed as a linear approximation to the demand function in a neighborhood around these initial conditions.

\begin{displaymath} \begin{array}[c]{l} (24)\,\,\Delta\,\log\left( {N\,X} \right... ...Delta\,\log\,Z\,-\,\Delta\,\log\,Z\ast} \right) \ \end{array}\end{displaymath}
The first term on the right hand side of equation (24) is the change in the price of exports relative to the price of total foreign expenditures converted into domestic currency; the coefficient on this term is the negative of the elasticity of substitution. The second term is the change in real expenditure in the rest of the world, with a coefficient of unity. The first two terms together comprise the standard Armington demand equation. The third term is the change in the ratio of domestic output to world output, also with a coefficient of unity. This term represents the main contribution of this paper, and its coefficient is the parameter of interest. The fourth and fifth terms are functions of changes in unobservable tastes (A*) and technology (Z, Z*).

For identification, it is necessary that the unobservable disturbances (the last two terms) are not correlated with the regressors (the first three terms). Within the system developed here, taste shocks ($ \Delta$log A*) are not correlated with prices, output, or expenditures.17 The underlying technology variables (F, G, F*, G*) are correlated with prices, output, and expenditure. However, they enter the demand equation directly only through a function of their ratio (Z=G/[($ \Sigma$-1)F]). Thus, identification requires only the plausible assumption that technological progress lowers both fixed and marginal costs proportionally. Under this assumption, $ \Delta$log Z= $ \Delta$log Z*=0, and the fifth term of equation (24) drops out.

IV  Empirical Results

This section presents estimates of the coefficients of equation (24) using data on long-run growth rates of exports.18 A critical test of the growth-led exports model is that the coefficient on the change in the ratio of exporter GDP to world GDP should be significantly greater than zero and not significantly different from unity.

The equation is estimated across countries using one long-run growth rate for each country. Using long-run growth rates eliminates the need to model short-run adjustment dynamics. In addition, the relationship between output and the number of varieties is likely to be strongest over long time-horizons, as the number of varieties may not move in proportion with output over the business cycle.

Table 2 presents estimates of equation (24) with heteroskedasticity-robust standard errors (Huber/White).19 The first three columns of Table 2 display ordinary least squares (OLS) regressions. Column (1) is based on growth rates over the period from 1960 through 2000.20 Columns (2) and (3) are based on growth rates over the first half and second half, respectively, of these 40 years. In all three samples, the ratio of exporter GDP to world GDP is highly significant in explaining export growth, lending support to the importance of product varieties and growth-led exports. Column (4) shows that these results are not sensitive to outliers in the data, as estimates from minimum absolute deviation (MAD) regressions are very close to the OLS results. Similar results (not shown) obtain for the sub-sample periods.

The coefficient on the relative export price is the negative of the substitution elasticity ($ \Sigma)$). The estimate of this coefficient has the correct sign but is rather close to zero in these regressions, suggesting the possibility of simultaneity bias. Simultaneity bias could also be present if exporter GDP growth responds positively to shocks in the growth rate of exports in the long run. Columns (5) and (6) explore these issues. Column (5) presents results of an instrumental-variables regression in which the ratio of the domestic to the foreign GDP deflator is used as an instrument for the relative export price and the instruments of Table 1 (except the oil dummy) are used for the ratio of exporter GDP to world GDP. The first-stage fit is acceptable, but the instruments do not improve the estimated elasticity of substitution. Indeed, the estimated substitution elasticity now has the wrong sign; nevertheless, the coefficient on the ratio of exporter GDP is little changed.21 Column (6) presents instrumental variables results under the restriction that the coefficient on relative export prices is -2, representing a much larger substitution elasticity than is typically found in aggregate-level implementations of the Armington model.22 This restriction has only a small effect on the parameter of interest-the coefficient on growth of the ratio of exporter GDP to world GDP remains highly significant and close to unity.

Column (7) displays estimates over a sub-sample of countries for which manufactured goods and services comprised more than 50 percent of exports in 2000.23 This sample selection was made because the Helpman-Krugman model was designed for differentiated manufactures and services, and thus it may not be appropriate for trade in undifferentiated primary commodities. Small countries that specialize in the export of a particular primary commodity may experience growth in both GDP and exports with little change in relative prices if their production of the commodity is small relative to world consumption. This phenomenon would lead to a positive coefficient on the exporter GDP ratio for reasons other than those embodied in the Helpman-Krugman model. Table A1 indicates which countries in the dataset do not specialize in primary commodity exports. For the most part, these are the traditional industrialized countries, especially when one excludes countries for which data are not available in 1960. Thus, another benefit of this reduced sample is to focus on countries with relatively high-quality data that account for most of world trade in manufactures and services. As seen in column (7) of Table 2, the coefficient on the ratio of exporter to world GDP remains highly significant in this smaller sample.24

Columns (8) and (9) explore the interaction between export-led growth and growth-led exports. The sample of column (1) is split into two equal-sized groups: those for which the share of exports in GDP moved closely in line with the sample median between 1960 and 2000-column (8)-and those for which the share of exports in GDP rose either more or less quickly than the median-column (9).25 If export-led growth were entirely responsible for the results of this paper, one would expect that the coefficient on the ratio of exporter GDP to world GDP would be strongly affected by this sample split, as nearly all the identifying information would be in the sample of column (9)-these are the countries for which exports grew especially strongly or weakly. Indeed, the coefficient on the ratio of exporter GDP is larger in column (9) than in column (8), but the difference is not significant and the coefficient in column (8) remains highly statistically significant. Thus, it appears that economic growth spurs exports even in countries that are not aggressively pursuing a strategy of export-led growth.26

In all columns of Table 2, the estimated effect of growth in the ratio of exporter to world output is highly statistically significant and generally not significantly different from its predicted value of unity. These results provide strong support for the role of product varieties in trade and for growth-led exports.

V  Conclusion

This paper shows how the Helpman-Krugman (1985) trade model can be implemented empirically by augmenting the standard Armington export demand equation with a term for the ratio of the exporting country's output relative to world output. The augmented equation is estimated using cross-country data on average export growth rates between 1960 and 2000 for up to 89 countries. The effect of the exporter output ratio is highly significant and robust to alternative samples and specifications.

These results imply that fast-growing countries need not experience growing trade deficits or secular declines in their terms of trade, as is implied by the Armington model. This finding has important implications for international macroeconomic analysis, including analysis of the effects of productivity shocks, as most empirical macroeconomic models utilize Armington trade equations. These results also support public policies that pursue export-led growth by allaying concerns about immiserizing effects on a country's terms of trade.

Table 1. Terms of Trade and Economic Growth, 1960-2000 (instrumental-variables estimates, robust standard errors)


      1960-1980 1980-2000 Per Capita Growth$ ^{1}$ Manuf. & Services$ ^{2}$ Industrial Countries
  (1) (2) (3) (4) (5) (6) (7)
Real GDP Growth 0.023

(.207)

 -0.023

(.170)

 0.029

(.238)

 0.199

(.205)

 -0.010

(.168)

 0.069

(.169)

 -0.144

(.231)
Initial Years of Schooling   -0.002**

(.001)

 -0.000

(.002)

 0.001

(.001)

 -0.002**

(.001)

 -0.001

(.001)

 -0.001

(.001)
Initial Life Expectancy   0.041***

(.011)

 0.024

(.017)

 0.027

(.018)

 0.045***

(.012)

 0.036**

(.014)

 -0.007

(.030)
Oil Exporter 0.008

(.005)

 0.007

(.006)

 0.036***

(.013)

 -0.014*

(.008)

 0.007

(.005)

 n.a. n.a.
R$ ^{2}$ .07 .36 .23 .21 .36 .22 .22
No. Obs. 47 47 56 74 47 30 18
First-Stage R$ ^{2}$ .43 .43 .43 .30 .53 .68 .84

***, **, and * denote significance at the 1, 5, and 10 percent levels, respectively. First stage regression for real GDP growth includes initial years of schooling, initial life expectancy, oil exporter dummy, initial per capita PPP GDP, and population growth. Real GDP growth replaced by real per capita GDP growth. Population growth dropped from first stage regression. Sample includes countries for which manufactured goods and services comprised more than 50 percent of exports in 2000.

 

Table 2. Growth of Real Exports of Goods and Services, Equation (24), 1960-2000 (robust standard errors)



    1960-1980 1980-2000 MAD $ ^{1}$ IV

PY/RPY$ ^{2}$

 IV

$ \sigma=2^{3}$

 Manuf. & Services$ ^{4}$ (X/Y) Stable$ ^{5}$ (X/Y) Changing$ ^{6}$
  (1) (2) (3) (4) (5) (6) (7) (8) (9)
$ \Delta$ Rel. Price Exports -0.34

(.21)

 -0.55*

(.29)

 -0.56***

(.19)

 -0.16

(.34)

 0.60

(.67)

 -2.00

(n.a.)

 -1.27**

(.61)

 -0.60

(.39)

 -0.35

(.24)
$ \Delta$ Foreign Expenditure 1.43***

(.08)

 1.45***

(.11)

 1.17***

(.20)

 1.47***

(.18)

 1.71***

(.18)

 1.09***

(.12)

 1.51***

(.13)

 1.42***

(.13)

 1.36***

(.11)
$ \Delta$ Ratio of Exporter GDP 1.50***

(.26)

 1.22***

(.28)

 1.31***

(.21)

 1.34***

(.36)

 1.36**

(.52)

 1.11***

(.40)

 1.10***

(.25)

 1.25***

(.38)

 1.60***

(.33)
R$ ^{2}$ .58 .42 .53 .57 .41 .33 .61 .37 .67
No. Obs. 53 55 89 53 39 39 28 27 26
***, **, and * denote significance at the 1, 5, and 10 percent levels, respectively.

$ ^{1}$Minimum absolute deviation regression. Foreign expenditure term replaced by a constant equal to average growth of foreign expenditure over the sample.

$ ^{2}$Instruments are the same as in Table 1 (except oil dummy) plus the ratio of exporter to foreign GDP deflator. First-stage R$ ^{2}$ = .34 for the relative price of exports and .48 for the ratio of exporter GDP to world GDP.

$ ^{3}$Coefficient on relative prices constrained to equal -2. Instruments are the same as in Table 1 (except oil dummy), with first-stage R$ ^{2}$ = .44.

$ ^{4}$Sample includes countries for which manufactured goods and services comprised more than 50 percent of exports in 2000.

$ ^{5}$Sample includes countries for which the change in the share of exports in GDP lies between the 25$ ^{th}$ and 75$ ^{th}$ percentile of all available countries.

$ ^{6}$Sample includes countries for which the change in the share of exports in GDP is either less than the 25$ ^{th}$ percentile or greater than the 75$ ^{th}$ percentile.


Figure 1:  Export Growth and Output Growth, 1960-2000

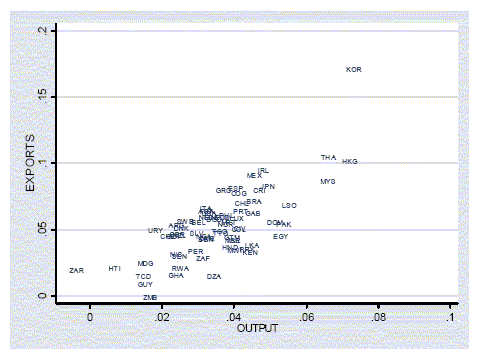
Figure 1 is a scatter plot outlining export growth against output growth for a variety of countries listed in Table 1 at the end of the paper. Both variables are calculated as average annual growth rates for the period spanning from to 1960 to 2000. The figure shows a clear positive correlation between the two variables, as the points seem to follow along a 45-degree line.

Figure 2:  Change in Terms of Trade and Output Growth, 1960-2000

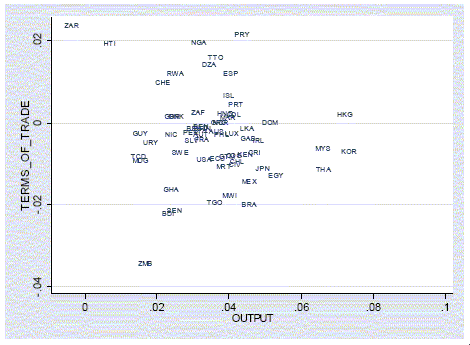
On the other hand, Figure 2 plots the change in terms of trade against output growth for those countries in Figure 1 during the same time period. Here the results are more obscure. The data points are widely scattered and at best a slight concentration of points exist in the center of the chart with a negligible negative correlation existing between the two variables.

Figure 3:  Economic Growth with Armington Export Demand

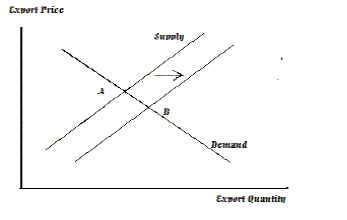
Figure 3 shows a standard upward-sloping supply and downward-sloping demand curve with the export quantity lying along the x-axis while the export price lies along the y-axis. In the graph, the intersection of the original demand and supply curves is at point A, but as faster economic growth shifts the export supply curve to the right, equilibrium moves down the demand curve to a new lower export price at point B.

Figure 4:  Economic Growth with Helpman-Krugman Export Demand

In Figure 4, long-run growth shifts both the export supply and export demand curves out to the right. In contrast to Figure 3, since both export demand and export supply curves shift outward, there is a minimum effect on the price of exports.

References

Acemoglu, Daron, and Jaume Ventura (2002) ``The World Income Distribution,'' The Quarterly Journal of Economics 117, 659-694.

Anderson, James E., and Eric van Wincoop (2003) ``Gravity with Gravitas: A Solution to the Border Puzzle,'' American Economic Review 93, 170-92.

Armington, Paul S. (1969) ``A Theory of Demand for Products Distinguished by Place of Production,'' IMF Staff Papers 16, 159-76.

Barro, Robert, and Jong-Wha Lee (1993) ``International Comparisons of Educational Attainment,'' Journal of Monetary Economics 32, 363-394.

Dixit, Avinash, and Joseph Stiglitz (1977) ``Monopolistic Competition and Optimum Product Diversity,'' American Economic Review 67, 297-308.

Gagnon, Joseph E. (2003) ``Productive Capacity, Product Varieties, and the Elasticities Approach to Trade,'' International Finance Discussion Papers No. 781, Board of Governors of the Federal Reserve System.

Goldstein, Morris, and Mohsin Khan (1985) ``Income and Price Elasticities in Trade,'' in Jones and Kenen (eds.) Handbook of International Economics, Volume II, North-Holland, Amsterdam.

Grossman, Gene, and Elhanan Helpman (1991) Innovation and Growth in the Global Economy, The MIT Press, Cambridge, MA.

Helpman, Elhanan, and Paul R. Krugman (1985) Market Structure and Foreign Trade: Increasing Returns, Imperfect Competition, and the International Economy, The MIT Press, Cambridge, MA.

Krugman, Paul (1989) ``Differences in Income Elasticities and Trends in Real Exchange Rates,'' European Economic Review 33, 1055-85.

Marquez, Jaime (2002) Estimating Trade Elasticities, Kluwer Academic Publishers, Boston.

McKinnon, Ronald (1964) ``Foreign Exchange Constraint in Economic Development and Efficient Aid Allocation,'' Economic Journal 74, 388-409.

Pereira, Alfredo, and Zhenhui Xu (2000) ``Export Growth and Domestic Performance,'' Review of International Economics 8, 60-73.

Senhadji, Abdelhak, and Claudio Montenegro (1999) ``Time Series Analysis of Export Demand Equations: A Cross-Country Analysis,'' IMF Staff Papers 46, 259-73.

Data Appendix

Most of the data are obtained from the World Bank's World Development Indicators 2004 database. Initial per capita PPP GDP and population are obtained from the Penn World Tables version 6.1.27 Initial human capital data are obtained from the Barro-Lee dataset.28 Terms of trade is defined as the ratio of the export deflator for goods and services to the corresponding import deflator. In Table 2, foreign data for each exporter are calculated as world minus exporter data. Data definitions for equation (24) are as follows:29

NX: Real exports of goods and services P$ ^{X}$: Export deflator

E: Nominal gross national expenditures P$ ^{E}$: Expenditures deflator

Y: Real gross output (GDP) P$ ^{Y}$: GDP deflator

Country coverage is described in the following table.

Table A1. Trade Data Coverage by Exporting Country

Country Symbol 1960 1980 2000 Man.&Serv.$ ^{1}$ Industrial$ ^{2}$
Algeria DZA x x x    
Antigua and Barbuda ATG   x x    
Argentina ARG   x x    
Australia AUS   x x   x
Austria AUT x x x x x
Belgium BEL x x x x x
Belize BLZ   x x    
Benin BEN x x x    
Bolivia BOL   x x    
Botswana BWA   x x x  
Burkina Faso BFA   x x    
Burundi BDI x x x    
Cameroon CMR   x x    
Canada CAN   x x x x
Chad TCD x x x    
Chile CHL x x x    
China CHN   x x x  
Colombia COL x x x    
Comoros COM   x x    
Congo (Brazzaville) COG x x x    
Congo (Zaire) ZAR x x x    
Cote d'Ivoire CIV x x x    
Denmark DNK x x x x x
Dominica DMA   x x x  
Dominican Republic DOM x x x x  
Egypt EGY x x x x  
El Salvador SLV x x x x  
Finland FIN x x x x x
France FRA x x x x x
Gabon GAB   x x    
Gambia GMB   x x    
Germany DEU   x x x x
Ghana GHA x x x    
Greece GRC x x x   x
Guinea-Bissau GNB   x x    
Guyana GUY x x x    
Haiti HTI x x x    
Honduras HND x x x    
Hong Kong HKG x x x x  
Hungary HUN   x x x  
Iceland ISL x x x   x
Indonesia IDN   x x x  
Ireland IRL x x x x x
Iran IRN   x x    
Italy ITA x x x x x
Japan JPN x x x x x
Jordan JOR x x x x  
Kenya KEN x x x    
Korea KOR x x x x  
Lesotho LSO   x x    
Luxembourg LUX x x x x x

Table 1. (cont'd.) Trade Data Coverage by Exporting Country


Country Symbol 1960 1980 2000 Man.&Serv.$ ^{1}$ Industrial$ ^{2}$
Madagascar MDG x x x    
Malawi MWI x x x    
Malaysia MYS x x x x  
Mali MLI   x x    
Mauritania MRT x x x    
Mauritius MUS   x x x  
Mexico MEX x x x x  
Morocco MAR   x x x  
Mozambique MOZ   x x    
Namibia NAM   x x x  
Netherlands NLD x x x x x
New Zealand NZL   x x   x
Nicaragua NIC x x x    
Niger NER x x      
Nigeria NGA x x      
Norway NOR x x x   x
Paraguay PRY x x x x  
Philippines PHL x x x x  
Portugal PRT x x x x x
Rwanda RWA x x x    
St. Kitts and Nevis KNA   x x x  
St. Lucia LCA   x x x  
St. Vincent & Grenadines VCT   x x x  
Senegal SEN x x x    
Sierra Leone SLE   x x    
South Africa ZAF x x x x  
Spain ESP x x x x x
Swaziland SWZ   x x x  
Sweden SWE x x x x x
Switzerland CHE x x x x x
Syria SYR   x x    
Togo TGO x x x    
Trinidad and Tobago TTO x x x    
Tunisia TUN   x x x  
United Kingdom GBR x x x x x
United States USA x x x x x
Uruguay URY x x x x  
Venezuela VEN   x x    
Zambia ZMB x x x    
Zimbabwe ZWE   x x    

$ ^{1}$Countries for which manufactured goods and services comprised more than 50 percent of exports in 2000. Source: World Development Indicators 2004.

$ ^{2}$IMF definition.

Appendix: Derivation of Equation (24)

Take the logarithm of equation (23) and totally differentiate. Make the following notational simplifications: P$ ^{X}$/R=PX, P$ ^{E}$*=PE*, P$ ^{D}$*=PD*.

(1) (2) (3)

dlog(NX) = -$ \Sigma)$ dlog(PX/PE*) + dlog(E*/PE*) + dlog[Y/(Y+Y*)]

(4) (5) (6)

+ ($ \Sigma)$-1) dlog(A*) + dlog[(Y+Y*)/Y*] + dlog(Z/Z*)

(7) (8)

+ (1-$ \Sigma)$dlog(PE*/PD*) - dlog{1 + Z Y [PX/(PD* A*)]$ ^{1-\sigma}$/(Z* Y*)}

Terms (1)-(3) above are the same as in equation (24) except that ``d'' is replaced by `` $ \mathrm{p}$''. Making use of dlog(X)=dX/X, term (8) can be written:

Term 8

-{Z Y (1-$ \Sigma)$) PX [PD* A* dpX - PX (PD* dA* + A* dPD*)]/[Z* Y*

(PD* A*)$ ^{3}$] + [Z* Y* (Y dZ + Z dY) - Z Y (Y* dZ* + Z* dY*)]

/(Z* Y*)$ ^{2}$}/{1 + Z Y [PX/(PD* A*)]$ ^{1-\sigma}$/(Z* Y*)}

Use of initial conditions - A*=1, PX=PD*, Z=Z* - allows simplification to

-{Y (1-$ \Sigma)$) (dPX - PD* dA* - dPD*)/(Y* PD*) + [Y* Y (dZ - dZ*)

+ Z(Y* dY - Y dY*)]/(Z Y*$ ^{2})$}/(1 + Y/Y*)

dividing both numerator and denominator by (1+Y/Y*)

[Y/(Y*+Y)](1-$ \Sigma)$)dA*

- [Y/(Y*+Y)](1-$ \Sigma)$)(dPX - dPD*)/PD*

- [Y/(Y*+Y)](dZ - dZ*)/Z - (Y* dY - Y dY*)/[(Y*+Y)Y*]

Term 4

($ \Sigma)$-1)dA*/A* (A*=1)

Combine with first term of simplified term 8 to yield

($ \Sigma)$-1)[Y*/(Y+Y*)]dA*/A* which is the fourth term in equation (24).

Term 5

[Y*/(Y+Y*)][Y*(dY+dY*)-(Y+Y*)dY*]/Y*$ ^{2}$

= [Y*/(Y+Y*)][Y* dY - Y dY*]/Y*$ ^{2}$

= [Y* dY - Y dY*]/[(Y+Y*)Y*]

which cancels out the fourth term of simplified term 8.

Term 6

(Z*/Z)(Z* dZ - Z dZ*)/Z*$ ^{2}$ (Z=Z*)

= (dZ - dZ*)/Z

Combine with third term of simplified term 8 to yield

[Y*/(Y+Y*)](dZ - dZ*)/Z which is the fifth term in equation (24).

Term 7

Use the definition of PE* in equation (22), defining

w = PD* D*/E* and (1-w) = PX X/E*.

(1-$ \Sigma)$) dlog{[w PD* + (1-w) PX]/PD*}

= (1-$ \Sigma)$)[PD*(w dPD* + PD* dW + dPX - w dPX - PX dW) - w PD* dPD*

- PX dPD* + w PX dPD*]/PD*$ ^{2}$

Substituting the initial condition: PX = PD*.

(1-$ \Sigma)$)(dPX - w dPX - dPD* + w dPD*)/PD*

= (1-$ \Sigma)$)(1-w)(dPX - dPD*)/PD*

Under the initial condition of no home bias (A*=1) the share of imports in expenditures (1-w) equals exporter's share of world output [Y/(Y+Y*)].

(1-$ \Sigma)$)[Y/(Y+Y*)](dPX - dPD*)/PD*

which cancels out the second term of simplified term 8.

Footnotes

1.  Assistant Director, Division of International Finance, Board of Governors of the Federal Reserve System. (Mail Stop 19, 2000 C Street NW, Washington, DC 20551; email: [email protected]) I would like to thank Jane Haltmaier, Jaime Marquez, Andrew Rose, and Robert Vigfusson for helpful comments. The views expressed here are my own and should not be interpreted as reflecting the views of the Board of Governors of the Federal Reserve System or of any other person associated with the Federal Reserve System. Return to text

2.  This research dates back at least to McKinnon (1964). For subsequent work, see Pereira and Xu (2000) and the references cited therein. Return to text

3.  Data sources and country coverage are documented in the Data Appendix. Return to text

4.  http://www.perjacobsson.org/lectures.htm.  Return to text

5.  An alternative model consistent with the lack of long-run correlation between export growth and the terms of trade is that of a small open economy whose exports are perfectly substitutable for foreign products. However, an extensive literature shows that for most countries, exports are far from perfect substitutes with foreign products. See, for example, Goldstein and Khan (1985) and Marquez (2002). Return to text

6.  This dummy variable includes all OPEC members plus Cameroon, Rep. Congo, Egypt, Gabon, Malaysia, Norway, Trinidad and Tobago, and Tunisia. Using an OPEC-only dummy, as in Acemoglu and Ventura, does not affect the results. Source: Energy Information Administration, International Energy Annual 2002. Return to text

7.  Acemoglu and Ventura focus on the effect of growth through capital accumulation on the terms of trade. Their model allows for growth in population and technology to increase exports without affecting the terms of trade, through a mechanism similar to that described in the next section. They find a negative and statistically significant effect of per capita growth on the terms of trade using these instruments, which are meant to proxy for the component of growth attributable to capital accumulation. Their results do not carry through to the latest vintage of World Bank data used here, even when the sample is restricted to their 1965-85 period. This may reflect the broader definition of the terms of trade-Acemoglu and Ventura use goods trade only-as well as somewhat different country coverage and possible revisions to the data. Within the original dataset used by Acemoglu and Ventura (from Barro and Lee (1993)) the results are sensitive to the selection of countries in the sample and the use of total versus per capita GDP. Return to text

8.  The criterion was a share of manufactured goods and services in total exports of more than 50 percent. Similar results obtain with a cutoff of 75 percent. Return to text

9.  For simplicity there is no capital stock. But labor can be interpreted as representing all factors of production. Return to text

10.  Varieties refers both to different types of goods-such as televisions, cars, and toothpaste-and to different brands and models of the same type of goods. Return to text

11.  For a review of the theoretical and empirical literature on the Armington export demand equation, see Gagnon (2003). The well-known gravity model of trade is a reduced form based on an Armington demand equation applied to bilateral trade. See, for example, Anderson and van Wincoop (2003). Time-series implementations of the gravity model share the property of the Armington equation that increases in export supply drive down the terms of trade. Return to text

12.  Grossman and Helpman (1991) employ a similar demand system with a richer supply side. Return to text

13.  A well-known property of the Dixit-Stiglitz utility function is that the household purchases a positive amount of every variety available. Thus, it is best considered a representative household rather than an individual household. Return to text

14.  Note that the elasticity of substitution is assumed equal across countries. This assumption aids in the derivation of a linear demand equation for estimation and it is also implicit in the cross-country empirical work of the next section. Return to text

15.  Krugman (1989) employs a similar cost function and obtains the same pricing equation. Return to text

16.  These equations imply that export prices equal domestic prices. Dropping the assumption of equal elasticity of substitution across countries would allow for differences between export and domestic prices. Return to text

17.  The empirical section below checks for robustness to the possibility that taste shocks may affect export prices or output. Return to text

18.  Gagnon (2003) estimates a related equation using bilateral U.S. imports of manufactures. Gagnon (2003) also reviews other empirical tests of the effect of product varieties on trade, most of which focus on direct measures of product variety. Return to text

19.  Note that there is no intercept term in the regressions, consistent with the specification of equation (24) in growth rates. Moreover, the data do not permit the addition of an intercept term, as growth of foreign expenditure is nearly identical for all exporters, creating severe collinearity between this term and an intercept. Dropping the intercept introduces a bias in the coefficient on foreign expenditures coming from taste shocks that are common to all exporters. From the point of view of an exporting country, foreign taste shocks include changes in trade barriers and transportation costs. To the extent that trade barriers and transportation costs have fallen for all exporters, the coefficient on foreign expenditure is biased upward. The remaining coefficients are not affected by this bias. Return to text

20.  There are only 53 countries in this regression (compared to 64 in Figures 1 and 2) because nine countries lacked one or more of the needed series in dollar terms. Return to text

21.  An alternative instrument, the trade balance, was associated with extremely poor first-stage fit and yielded similar results for the coefficient on the ratio of exporter GDP. Return to text

22.  Senhadji and Montenegro (1999) report a median price elasticity of export demand of -0.78 across 53 countries. See, also, Marquez (2002). Return to text

23.  Similar results were obtained using a criterion of 75 percent of exports. Return to text

24.  Similar results obtain for the industrial countries and over the two subsamples. Return to text

25.  As described in Table 2, the cutoff points for this sample split are the 25$ ^{th}$ and 75$ ^{th}$ percentiles of growth in export shares. Return to text

26.  An alternative sample split based on countries with export shares growing either faster or slower than the median yielded a higher coefficient on the sub-sample with fast-growing exports, but the coefficient on the slow-export-growth sub-sample remained positive and highly significant. Return to text

27.  Alan Heston, Robert Summers and Bettina Aten, Penn World Table Version 6.1, Center for International Comparisons at the University of Pennsylvania (CICUP), October 2002. Return to text

28.  See Barro and Lee (1993). A link to their dataset is at http://www.nber.org/data/. Return to text

29.  All countries with available data were used in the regressions except for Bulgaria, which had strongly negative export growth in the second sub-sample that is related to its transition from a socialist to a market economy. No transition economy has data over the 40-year sample. Bulgaria, China, and Hungary have data over the 1980-2000 sub-sample period. Return to text


This version is optimized for use by screen readers. A printable pdf version is available.

Home | Economic research and data | Publications and education resources
Accessibility | Contact us
Last update: October 24, 2006